In this answer we'll start by making the simplifying assumption that the Thrust vector is parallel to the Drag vector and the direction of the flight path with respect to the surrounding airmass.
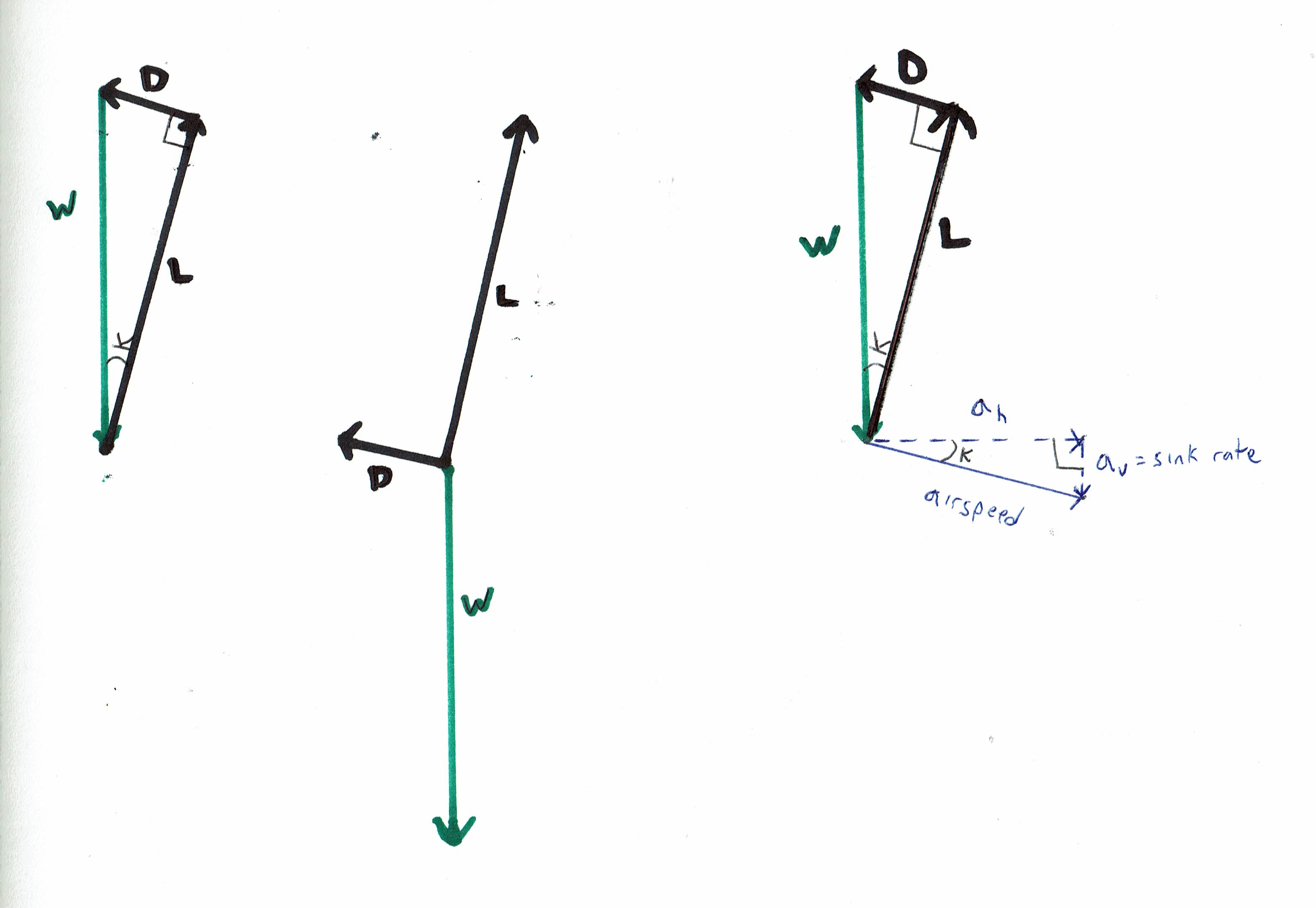
See the vector triangles of Weight, Lift, and Drag for gliding flight illustrated in these related answers (#1, #2). (The diagram in the second link is now reproduced at the top of the present answer as well.)
We can adapt these questions to a powered descent by simply substituting a vector labelled "Drag minus Thrust" in place of the vector labelled "Drag". We'll still have a closed vector triangle.
Once we make this simple substitution, the angle labelled "K" in the diagram at the top of this answer will be the aircraft's angle of descent at some particular angle-of-attack and L/D ratio and Thrust output, in still air. This is also the aircraft's angle of descent with respect to the surrounding airmass.
Note that Lift = Weight * cosine (K).
This means that Lift is less than Weight, and the steeper the still-air glide angle "K", the larger the difference between Lift and Weight.
To achieve the same descent path with respect to the ground with a headwind, we must change to a flatter descent path with respect to the surrounding airmass. Whether we increase Thrust, decrease Drag, or both, we must make the vector we've re-labelled "Drag minus Thrust" become smaller. This will decrease the size of angle K. This will increase the size of the Lift vector.
To achieve the same descent path with respect to the ground with a tailwind, we must change to a steeper descent path with respect to the surrounding airmass. Whether we decrease Thrust, increase Drag, or both, we must make the vector we've re-labelled "Drag minus Thrust" become larger. This will increase the size of angle K. This will decrease the size of the Lift vector.
Since Lift = Weight * cosine (K), the Lift vector must be larger when we are descending with a headwind than when we are descending with a tailwind, if we are achieving the same glide path with respect to the ground.
Note that this is true regardless of whether we're constraining the angle-of-attack to be the same in both cases, or the airspeed to be the same in both cases, or neither. This is also true regardless of whether we're descending on the "front side" of the power curve or the "back side" of the power curve. None of these things modify the fact that to stay on a fixed glide slope relative to the ground, angle "K" must be larger when we are descending with a tailwind than when we are descending with a headwind. Therefore the Lift vector must be larger when we are descending with a headwind than when we are descending with a tailwind.
Of course, as we vary angle K, the variation in the size of the Lift vector will be so small as to be negligible in the real world. For example, when gliding with a still-air glide ratio of 7:1, which corresponds to a still-air glide angle of about 8 degrees, the Lift vector is about .99 * Weight. If we were to change our glide path with respect to the airmass from a 7:1 glide ratio to a glide ratio of infinity (i.e. horizontal flight), the size of the Lift vector would only increase by a factor of about 1.01. Still, technically speaking, the flatter our glide angle with respect to the surrounding airmass (i.e. the larger our still-air glide ratio), the larger the Lift vector must be.
So the basic answer to the question is that the Lift vector is ever-so-slightly smaller when the aircraft is descending with a tailwind than when the aircraft is descending with a headwind, because to stay on the same glide slope with respect to the ground, the still-air glide angle must be larger (i.e. the still-air glide ratio must be poorer) in the former case.
Let's consider one specific idea in the original question--
I was arguing that if you have tailwind you will have to increase
angle of attack which means that parasite drag will increase so the
value of lift will have to increase.
This is a flawed thesis. To fly with the same airspeed, why would you need a higher angle-of-attack when you have a tailwind? We've seen that-- disregarding any effects from a tilted thrust line relative to the direction of the flight path-- the Lift vector actually must be slightly smaller when the still-air glide path is steeper, which describes the tailwind case. To keep the airspeed constant, the aircraft must be flown at a slightly lower angle-of-attack when descending with a steeper still-air glide path (i.e the tailwind case) than when descending with a shallower still-air glide path (i.e. the headwind case).
Now for another twist--
If we complicate the picture by recognizing that the Thrust line may be tilted to point somewhat upward or downward of the direction of the Drag vector and the flight path through the airmass, this may dwarf the effects described above. The original question constrains the airspeed to be the same in the headwind case as the tailwind case, so the aircraft must be flying at nearly the same angle-of-attack in each case. Imagine a case where we are flying our approach "on the back side of the power curve", at a high angle-of-attack, with the engine pointed significantly upward in relation to the direction of the flight path through the airmass. The component of the engine's Thrust vector that acts perpendicular to the flight path effectively counts as part of the "Lift" vector in our vector diagram, meaning that the wing's actual Lift vector must be reduced to smaller than Weight * cosine (K). In a case like this, reducing the power setting to make the glide path steeper may well force the wing's lift to increase. On the other hand, if we are flying our approach at a low angle-of-attack, well on the "front side" of the power curve as per normal practice in a light airplane, it is no longer so obvious which direction the Thrust vector is pointing in relation to the direction of the flight path through the airmass. Since many light aircraft have some built-in down thrust, the Thrust vector may well point slightly downward in relation to the direction of the flight path, so that the wing must produce more Lift when the engine's power setting is increased. This would magnify the effect we discussed earlier in this answer-- the tendency for the Lift vector to be larger when the still-air glide angle is flatter, due to the relationship Lift = Weight * cosine (K).
At the end of the day, all these effects are so small that it's unlikely a pilot could detect the change in the magnitude of the wing's Lift vector for a given airspeed at various power settings and still-air glide angles. But in theory there is some variation in the size of the Lift vector as the power setting and still-air glide angle are changed. And for the simple case where we are assuming that the thrust line acts parallel to the Drag vector, the nature of the variation is such that the Lift vector is slightly larger when the still-air glide path is flatter (i.e. at higher power settings, such as when trying to stay on glide slope with a headwind) than when the still-air glide path is steeper (i.e. at lower power settings, such as when trying to stay on glide slope with a tailwind).
See also these related answers to related questions:
Can we show through simple geometry rather than formulae or graphs that the best glide ratio occurs at the maximum ratio of Lift to Drag? -- a concise answer with clear diagrams
Can we show through simple geometry rather than formulae or graphs that the best glide ratio occurs at the maximum ratio of Lift to Drag? -- a longer answer that emphasizes many concepts essential to the present answer
Why is the L/D ratio numerically equal to the glide ratio? -- similar to link immediately above
'Gravitational' power vs. engine power -- the variation in the size of the Lift vector as the still-air glide ratio changes comes into play here, exactly as it does in the present answer
Does lift equal weight in a climb?

