Does lift equal weight in a climb?

Question

This subject keeps coming up in the discussions and questions such as this one, which asks if lift equals weight in level flight. Good answers there, pointing out that upwards force has many sources. But also some that need clarification.

It is also mentioned at several places on this Aviation SE site, in question & comments, that lift always equals weight if the aeroplane is not accelerating upwards or downwards, since only an acceleration requires extra force according to Newton.

With zero wind, lift is always defined as the force perpendicular to the flight path, but gravity does not tilt with the aircraft axes. My question therefore is also about the sum of all vertical forces: in a steady climb, is the total upwards vertical force from all sources (wing, tail, engines, fuselage) larger than, or equal to the weight of the aircraft.

• If larger: please quantify.
• If equal: please explain why.

Answer 1

It depends on exactly how you define "lift" and "weight". You might say intuitively that lift is all the forces acting on the aircraft in the upward direction, like this:

In this case, lift must equal weight, otherwise the aircraft would be accelerating. That is, it's rate of climb would be changing.

But it's more usual to define lift this way:

Here, lift and weight are equal in magnitude, but in different directions. Of course lift doesn't need to be equal in magnitude: it can be adjusted by the angle of attack. But let's suppose lift is equal to weight and see what happens.

Let's do all our calculations with Earth as the frame of reference¹. It's useful to decompose lift into a sum of vertical and horizontal components so we can analyze the horizontal forces and the vertical forces separately:

Comparing the vertical component of lift with weight, we can see they are not equal:

Considering only the vertical forces drawn here, there is a net downward force on the aircraft. So why then is the rate of climb not decreasing?

A similar transformation happens to thrust. In a climb, thrust provides an additional upwards component. And of course we must also consider drag. Point being in a steady climb, lift (by the conventional definition) is not equal to weight, but the sum of all the vertical components of lift, thrust, and drag do equal weight.

Let's add an arbitrary amount of drag, and enough thrust to balance the vertical forces.

Now the vertical forces are balanced, but the horizontal forces must also be balanced if we want stable flight. Adding all the horizontal forces in my drawing, there's a net force to the left. So this aircraft may be maintaining a steady rate of climb at this instant, but it's losing speed and probably headed for a stall.

Remember, we initially set lift equal in magnitude to weight, and this is what happens. Without changing the direction or magnitude of lift, there's no solution that results in stable flight.

Therefore, a climbing aircraft requires less lift. To maintain this direction and velocity, this pilot must reduce lift by reducing the angle of attack, and increase thrust such that the vectors add to zero and there's no net force on the aircraft. Reducing lift will also reduce drag.

---

¹ Any other frame of reference could work. For example we could use the aircraft as the frame of reference, which would mean lift is always up, but weight would change direction.

Answer 2

In an aircraft that is climbing at a constant vertical velocity, the total of the upward-directed vertical forces is the same as the total of the downward-directed vertical forces.

Were it not so, the vertical velocity would not be constant, since any non-zero balance of the vertical forces would result in an acceleration...

Answer 3

Short answer: No.

Long answer: When the flight path is not horizontal, lift will not be vertical but perpendicular to the direction of motion (in still air). Thrust will also have a vertical component and is different in magnitude from drag, because excess thrust is needed to increase the potential energy of the plane. Note that the vertical component of lift is proportional to the cosine of the flight path angle while the vertical component of thrust is proportional to the sine of the flight path angle, so the thrust part grows more quickly at small flight path angles. Therefore, when climbing, thrust will add some vertical component, so less lift is needed.

Again, in a descent less lift is needed. Now thrust is smaller than drag, and drag, pointing slightly upwards, contributes a vertical component, counteracting weight. So in both cases lift is smaller than weight.

So far, this has been unaccelerated flight. But normally a climb has acceleration components:

• to adjust speed to the change in density (accelerated in order to stay at the same indicated airspeed) or Mach number (decelerated in order to stay at the same Mach number), and

• because the aircraft loses vertical speed as thrust is diminished by the change in density and, in case of propeller aircraft and turbofans, by the increase in true airspeed.

This second, admittedly tiny, effect will add a vertical inertial force which adds to the remaining vertical forces, namely lift and thrust. When this inertial force is considered, the remaining vertical forces are a tiny bit lower than weight.

Answer 4

If we define lift as the component of the total aerodynamic forces on the aircraft that is perpendicular to its direction of motion, then lift will be slightly smaller in a stable climb.

It is probably easiest to analyze the situation in a coordinate system that is tilted such that one of the axes is parallel to the direction of motion. Then all of the forces -- lift, drag, thrust -- work just like in an ordinary coordinate system in horizontal flight. The only difference is that the weight force now has a different direction -- but still the same magnitude.

This means that the component of weight that is perpendicular to the motion is now slightly smaller, and lift must be correspondingly smaller too. The plane's angle of attack will be slightly smaller than in level flight at the same (calibrated) airspeed.

On the other hand, the weight vector now gains a significant component parallel to the direction of motion, and this has to be counteracted by more thrust, lest the aircraft would slow down. (This will much dominate over the small decrease in induced drag that results from the slightly smaller lift).

Answer 5

TL;DNR

Does lift equal weight in a steady state climb? Vertical force is higher in a steady state climb, but lift may be tilted depending on how the climb is executed, is the answer. Tilt the aircraft axis relative to earth axes, and by definition part of the gravity vector is now in the aeroplane thrust/drag axis. That is very clear, and the case that everyone refers to in their answers in great detail. However, a steady state climb can also be executed with the nose pointing straight forward, and then lift is larger than weight. And helicopters are aircraft as well...

Full answer

It depends on the relative axis orientation.

• Gravity is always aligned with earth axes.
• For fixed wing aircraft, lift and drag are aligned with the airflow axes (aligned with the airflow in a steady state starting position). Note that thrust is only aligned with drag at AoA zero.

The thing is that for fixed wing aircraft, a steady state climb is mostly and automatically associated with increasing AoA, which tilts the aircraft axes upwards, resulting in an upward tilt of the airflow axes. But fixed wing aircraft can also climb by increasing speed, which results in a steady state climb with a reduced AoA.

Below is an analysis of the two cases for fixed wing climb, and for helicopters where the airflow axes are rotating with the blades - providing lift, not thrust.

TL;DNR

• Fixed wing climb by increasing AoA: modulus of the lift vector < gravity vector
• Fixed wing climb by increasing velocity: mod lift > mod g
• Helicopter in steady climb: mod lift >> mod g

• Lift L at angle $\alpha$
• Drag D at angle $\alpha$
• Thrust T at angle $\phi$
• Weight W at the vertical

Force equilibrium in unaccelerated flight:

$$ T\cdot cos(\phi) = L\cdot sin(\alpha) + D\cdot cos(\alpha) \tag{H} $$ $$ L\cdot cos(\alpha) + T\cdot sin(\phi) = D \cdot sin(\alpha) + W \tag{V} $$

Equation (V) states that the total upwards vertical force is equal to weight plus a component of aerodynamic drag - of the whole aircraft, wing + fuselage + tail etc. So the total upwards force will always be greater than weight, unless $\alpha$ = 0

Let's have a look at a couple of cases.

1. Climb due to increase in speed, fixed wing

A case pointed out some time ago by Chris, who defined totally uncoupled thrust and lift forces by putting a wing on a pole mounted on a train car. If thrust increases, speed will increase and the wing will climb upwards with a constant velocity $V_z$. This will change angle of attack, and will tilt the lift vector backwards. The wing climbs with constant velocity once the total upwards vertical force is identical to weight, plus the vertical component of drag which points downwards.

Note that thrust is nowhere to be seen in this picture, only aerodynamic forces. Thrust is set at angle $\phi$ = 0 and will be equal to L * sin($\alpha$) + D * cos ($\alpha$). Lift L is tilted backwards by angle $\alpha$, and is greater than the vertical upward force by factor $1/cos (\alpha) $.

So in this case (climb by increase in speed):

• Total upward force is greater than weight by an amount of D * sin $\alpha$.
• Lift is the only contributor to upward force, is tilted back, and is greater than total upward vertical force.

2. Climb due to aircraft pitch up, fixed wing

Now let's have a closer look at the case of a fixed wing aircraft, climbing because of an increase in pitch angle. All the above forces and both equation (H) and (V) are to be considered. Angle of attack $\alpha$ is defined by pitch angle $\phi$, airspeed V, and climb speed $\dot{z}$.

So in this case:

• Total upward force is again greater than weight by an amount of D * sin($\alpha$)
• Both thrust T and lift L are contributors to total upward force. How much each contributes depends on pitch angle $\phi$ and climb speed $\dot{z}$. More axes tilt means: smaller proportion of Lift, larger proportion of Thrust.

3. Helicopter in vertical climb

Now for the helicopter in climb. At first glance, this is a case of only thrust being responsible for the climb action, because the rotor disk delivers vertical thrust downwards. But here is the thing: that is from a fuselage perspective, but now lift is defined relative to the rotating blade airspeed.

Our reference frame is once again earth axes. The vertically climbing helicopter has the same downwards aerodynamic force as the hovering helicopter, plus minor increases due to the vertical drag of the fuselage. The pilot transitioned the helicopter from hover to climb by pulling on the collective, increasing blade pitch and tilting the lift vector backwards (earth axes).

The vertical component of lift is equal to weight plus the downward vertical component of (blade drag + vertical fuselage drag). Lift is greater than its vertical component by a factor of 1/cos $\phi$.

So in this case (climb by increase in pitch):

• Total upward vertical force is greater than weight by an amount of (D * sin($\alpha$) + vertical fuselage drag).
• Lift is the only contributor to upward vertical force and is tilted back, so lift is greater than total vertical force by factor 1/cos($\alpha$).

Conclusion

Case 2. is considered multiple times on this site. Aerodynamic lift may be less than weight, depending on relevant angles and speeds. Thrust must always be higher than in steady horizontal flight by an amount of L * sin($\alpha$).

All cases have a higher upward vertical force than weight: a vertical aerodynamic drag component must be compensated for. Intuitively clear from this example.

@xxaviers answer is accepted. Many other answers are also correct for a steady state fixed wing climb due to aircraft axes tilt relative to gravity.

Answer 6

The title of the question is different from the body of the question.

In the body of the question we read-

My question is purely about the sum of all vertical forces

Obviously for acceleration to be zero, net force must be zero, so net vertical aerodynamic force must be equal to weight. (In this answer, we'll consider the thrust vector to be an aerodynamic force.)

in a steady climb, is the total upwards vertical force from all sources (wing, tail, engines, fuselage) larger than, or equal to the weight of the aircraft

In a steady climb, net vertical force must be zero, so net vertical aerodynamic force must be equal to weight. However, this does not mean that the sum of all the upward vertical forces is equal to weight. It is not, because one of the aerodynamic forces--the drag vector-- has a downward component in a climb. Therefore the sum of all the upward vertical forces must be equal to weight plus the downward vertical component of the drag vector.

The title of the question, on the other hand, reads:

Does lift equal weight in a climb?

This is a distinctly different question-- and a more interesting question-- than a question about the net vertical force.

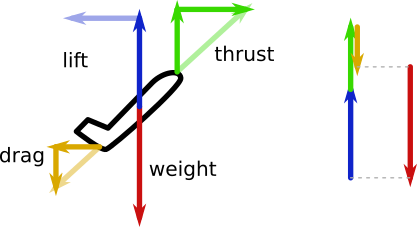
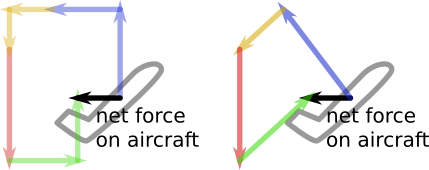
In the context of fixed-winged flight, Lift is defined to act perpendicular to the flight path through the airmass, and Drag is defined to act parallel to the flight path through the airmass. For the purposes of the answer, we'll assume that Thrust acts parallel to the flight path through the airmass, although this clearly not always exactly true. This simplifying assumption leads to the following vector diagram:

Powered climb at climb angles of 45 and 90 degrees:

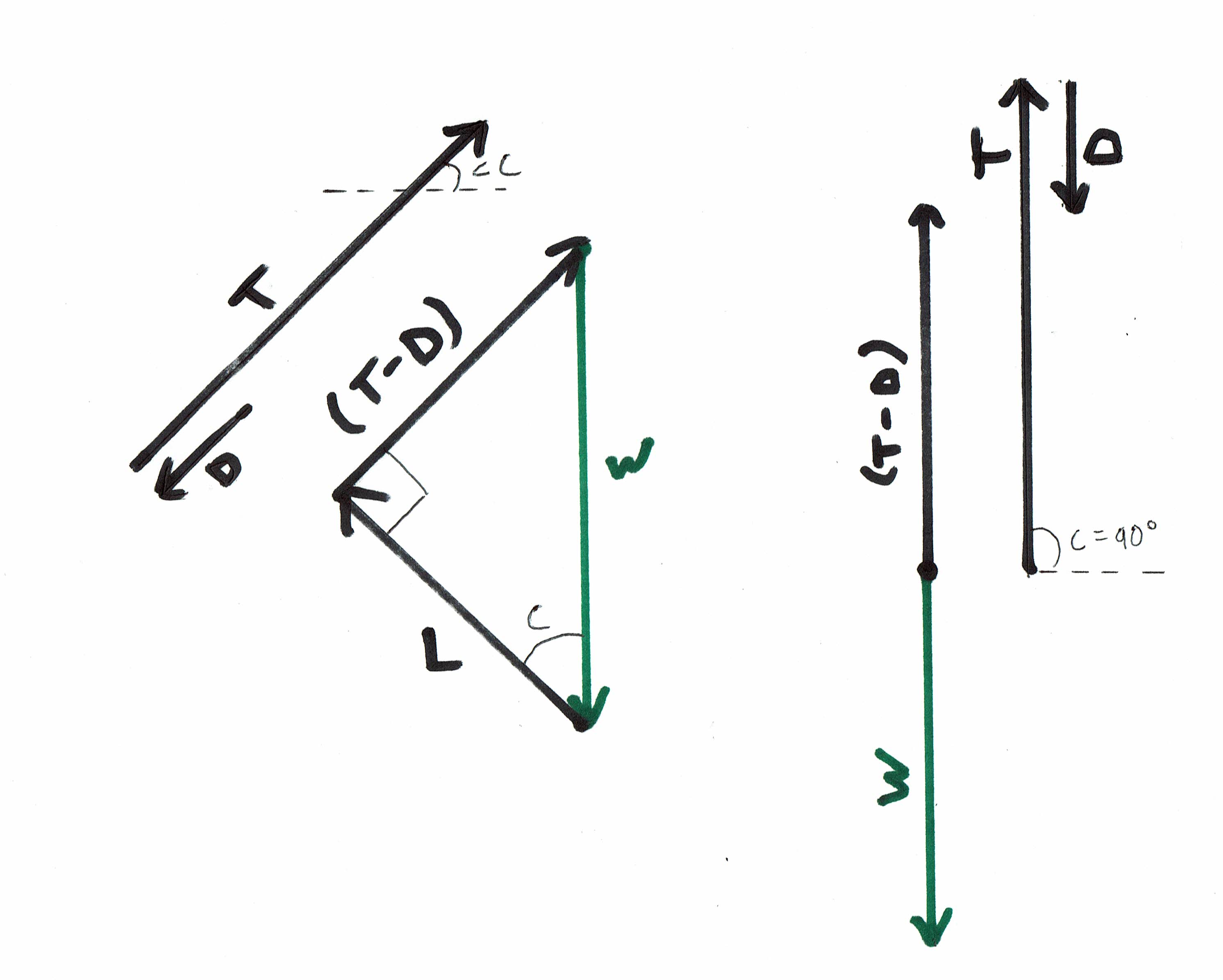
In the vector diagrams above, "angle c" is the climb angle-- it is 45 degrees in the left-hand figure, and 90 degrees in the right-hand figure.

We can see that in a powered climb, Lift = Weight * cosine (climb angle), where the climb angle is measured relative to the airmass (an important distinction in the case of gliding flight-- an unpowered climb in a thermal updraft is still a descent in relation to the airmass!)

Clearly, Lift is less than Weight in a powered climb. For example, if the climb angle is 45 degrees, Lift = .707 * Weight. If the climb angle is 90 degrees, Lift must be zero.

The same is also true in a descent-- Lift = Weight * cosine (descent angle), so Lift is less than Weight. This is explored in more detail in some of the links given at the end of this answer.

Note that we've taken the approach of combining the Thrust and Drag vectors into a single (Thrust-Drag) vector, and then we've arranged this vector into a closed vector triangle with Lift and Weight. Whenever vectors can be arranged nose-to-tail into into a closed polygon-- a triangle in this case-- this shows that net force must be zero, meaning that acceleration is zero and velocity i constant. For clarity we've also drawn the individual Thrust and Drag vectors outside the vector triangle. These are redundant with the (Thrust-Drag) vector.

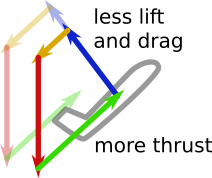
Varying the climb angle and/or the L/D ratio:

Note that for a given aircraft in a given configuration, any given angle-of-attack is associated with specific values for the lift coefficient, drag coefficient, and ratio of lift coefficient / drag coefficient. Lift is proportional to lift coefficient * airspeed squared, and drag is proportional to drag coefficient * airspeed squared, so the ratio of the lift coefficient / drag coefficient is also the ratio of Lift / Drag. So for a given aircraft in a given configuration, any given angle-of-attack is associated with specific ratio of Lift to Drag.

If the left-hand diagram above and the middle diagram above both represent the same aircraft in the same configuration, then the aircraft must be flying slightly slower in the middle diagram. That's the only way the L and D values can both be slightly smaller, for the same L/D ratio. Adding power to increase the climb angle, while holding the angle-of-attack constant, makes the airspeed decrease slightly. However in the case illustrated here, the change in airspeed would be too tiny to ever notice in practice-- it would be equal to the square root of the change in the value of the magnitude of the lift vector or drag vector.

If all the diagrams represent the same aircraft in the same configuration of flaps etc, then the right-hand diagram (5:1 L/D ratio) would represent a lower angle-of-attack than the left-hand or middle diagrams (10:1 L/D ratio). (We'll ignore the other possibility that the 5:1 case represents mushing flight very near stall, where drag is very high.) A lower angle-of-attack means a lower lift coefficient, yet the size of the lift vector is the same, so the airspeed must be higher in the case illustrated in the right-hand diagram. Therefore the climb rate is also higher. In short, when we increase thrust to increase our climb rate, we also must reduce angle-of-attack, if for some reason we wish to keep our climb angle constant rather than allowing it to increase.

Powered climb at 45-degree climb angle at 8 different ratios of Lift to Drag:

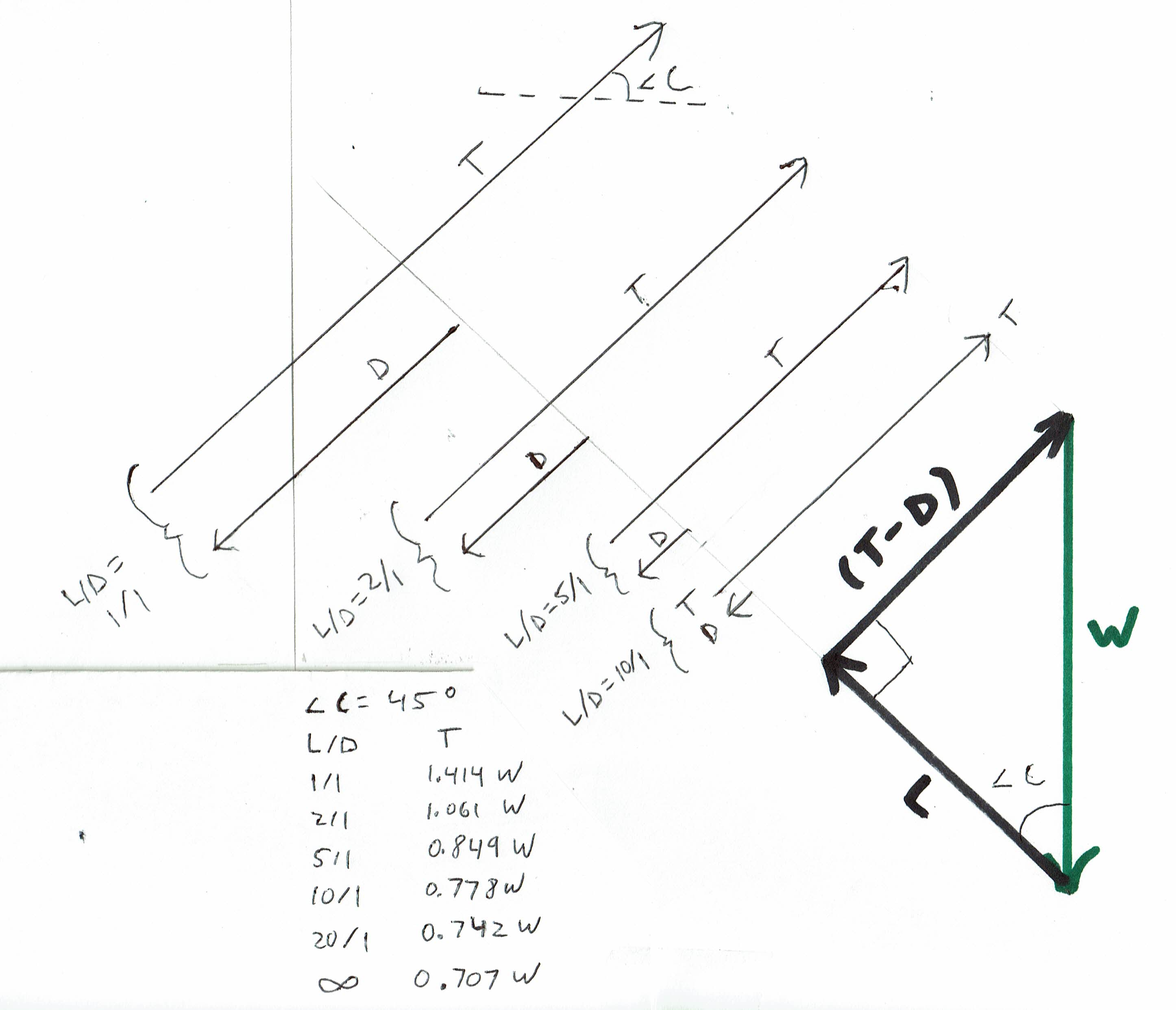
Note that as we decrease our L/D ratio, it takes more and more thrust to maintain the same 45-degree climb angle. In the case where the L/D ratio is 2/1, thrust must actually be greater than weight! This is a bit counterintuitive, as we could obviously climb straight up with some small but non-zero airspeed if thrust were only slightly greater than weight. However, that vertical climb would be conducted at a very low airspeed. In the diagram above, if all cases represent the same aircraft in the same configuration, by constraining the climb angle to be constant, so that L also must remain constant, we're constraining the airspeed to get progressively higher and higher as we reduce the angle-of-attack, lift coefficient, and L/D ratio. Hence the huge increase in drag, and thrust-required, as we reduce the angle-of-attack, lift coefficient, and L/D ratio.

As we explore climb angles closer and closer to 90 degrees, the L/D ratio has less and less influence on the thrust required. A figure similar to the one above, but for a climb angle of 60 or 70 degrees, would show less increase n thrust-required as we decrease the angle-of-attack, lift coefficient, and L/D ratio than we see at a climb angle of 45 degrees. This also implies that we're forcing less of an increase in airspeed as we decrease the angle-of-attack, lift coefficient, and L/D ratio in such a case. That makes sense-- as thrust carries more and more of the aircraft weight, the dynamics of the wing have less and less influence upon airspeed. In the case of a truly vertical climb, the wing must be at the zero-lift angle-of-attack and the L/D ratio must be zero. In such a case, of course, the drag force still varies with airspeed, and so the faster we want to fly straight up, the more thrust we need.

For the sake of clarity, this answer has focussed on some rather steep climb angles. It's important to also keep in mind that for shallow climb angles (or descent) angles that are typical of general aviation light aircraft, the cosine of the climb angle is not much smaller than 1, and so Lift is nearly equal to Weight (specifically, Lift is only slightly less than Weight.) Since Weight doesn't vary with climb or dive angle, we can conclude that for shallow climb or dive angles-- with no other accelerations going on (specifically, the flight path isn't curving up or down, and the wings aren't banked so the flight path isn't curving to describe a turn)-- Lift is also nearly constant, regardless of whether the aircraft is climbing, descending, or neither. This means if the climb or descent angle is shallow and the net G-load is one, the airspeed indicator can also be interpreted as an angle-of-attack gauge. Why should this be so? To keep lift nearly constant, it must be approximately true that the lift coefficient is varying in inverse proportion to the square of the airspeed. This establishes a nearly fixed relationship between airspeed and angle-of-attack, for shallow climb or descent angles and net G-loadings near one. If the airspeed is low, the lift coefficient and angle-of-attack must be high, and if the airspeed is high, the lift coefficient and angle-of-attack must be low, regardless of whether the aircraft is climbing at a shallow angle, descending at a shallow angle, or flying horizontally. So the airspeed indicator is in essence an angle-of-attack gauge at shallow climb or descent angles. At very steep climb angles where lift is quite a bit less than weight, things get more complicated-- a given angle-of-attack will be associated with a lower airspeed than in horizontal flight, and a given airspeed will be associated with a lower angle-of-attack than in horizontal flight. In the most extreme case where the aircraft is climbing straight up, lift must be zero, so the lift coefficient must be zero, and the angle-of-attack must be nearly zero (actually it must be slightly negative, unless the airfoil is completely symmetrical), no matter what the airspeed indicator reads. Clearly the airspeed indicator cannot serve "double duty" as a guide to angle-of-attack in such a situation.

We've also assumed throughout this answer that the Thrust vector acts parallel to the flight path through the airmass. Obviously, if this isn't true, then the equation lift = weight * cosine (climb angle) is also no longer true. To take an extreme case, note that when the exhaust nozzles of a Harrier "jump jet" are pointed straight down, the wing is "unloaded"-- the plane can hover at zero airspeed with zero lift, supported entirely by thrust. Conversely, during a glider winch launch, the towline pulls steeply downward on the glider. This too can be viewed as a form of "vectored thrust"-- but now the load on the wing is increased, rather than decreased, so the wings must generate a lift force that is much greater than the aircraft's weight. At any rate, it's best to thoroughly understand the simple case where the thrust vector acts parallel to the flight path, before going on to consider more exotic cases.

To see a vector diagram of the forces in climbing flight from an outside reference source, see the diagram below. This diagram shows the same relationships as the other diagrams included in this answer, but the forces have not been arranged into a closed vector polygon, so it is less obvious that the net force is zero.

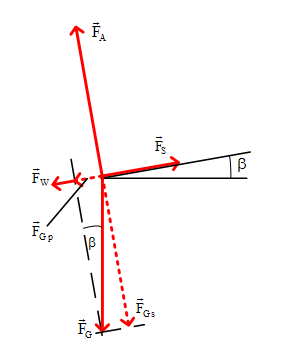
Above is a vector diagram showing the forces in a stabilized, linear, constant-airspeed climb -- from https://systemdesign.ch/wiki/L%C3%B6sung_zu_Steigflug

FS = thrust

FW = drag

FGp is the component of weight that acts parallel to the flight path, and is ALSO exactly equal in magnitude and opposite in direction to (thrust - drag)

FGs is the component of weight that acts perpendicular to the flight path, and is ALSO exactly equal in magnitude and opposite in direction to lift.

FA = lift

FG = weight

Angle beta is the climb angle-- the angle between the flight path and the horizon.

Addendum:

Another answer to the present question addresses a "climbing by increasing airspeed" case, which is also characterized by a completely fixed pitched attitude. Perhaps a better description of this case is "entering a climb without pitching up at all". This introduces a downthrust component relative to the flight path. This downthrust is the root cause of the increase in the lift vector above and beyond the "standard" value of weight * cosine (climb angle).

The present answer assumes no downthrust or upthrust. If downthrust or upthrust is present, the full equation for the magnitude of the lift vector is weight * cosine (climb angle) + thrust * sine (downthrust angle), where the downthrust angle is measured relative to the flight path, not the horizon. Treat upthrust as negative downthrust.

When we start in level cruise with no downthrust, and then enter a climb without pitching up at all, the downthrust angle is now so large that lift is indeed greater than weight. The climb angle and the downthrust angle are the same in this situation. Note that as we increase thrust and begin climbing without allowing the aircraft to pitch up at all, the wing's angle-of-attack must decrease, which likely means that we are pushing forward on the control yoke or stick. Naturally, this is not the normal way to conduct a climb! See this ASE answer for more on the "climbing without allowing the aircraft to pitch up" case, in the context of an another analogous situation -- the "propeller train".

See these related answers to related questions:

"Are there any situations where having high lift but low lift to drag ratio would be beneficial?"

"What produces Thrust along the line of flight in a glider?"

"'Gravitational' power vs. engine power"

"Descending on a given glide slope (e.g. ILS) at a given airspeed— is the size of the lift vector different in headwind versus tailwind?"

"Are we changing the angle of attack by changing the pitch of an aircraft?"

"Is excess lift or excess power needed for a climb?"

Answer 7

No the lift will not equal the weight for an aircraft climbing (at constant velocity).

I cannot draw where I am at, so bear with me.

For an aircraft travelling at constant velocity, not undergoing acceleration, either vertically or horizontally, the lift generated by the wing will be less than the aircraft weight. You can see that the lift component will be less than the weight vector as you increase the climb angle. For example at a climb angle of 45 degrees, the lift component will equal square-root(2)/2 of the weight (or roughly 71% of the weight).

So how can the aircraft continue in a straight path upward? The engines provide thrust that applies a force equal to the difference in lift and weight. You can see this if you draw a force-balance diagram (which I will try to do later).

Answer 8

In flight dynamics (not aerodynamics), Lift is in the opposite direction along the stability Z axis. This is also where Lift Coefficient (CL) is calculated from.

It's not in the body axis, not in the earth axis, but in the stability axis. However you can use both wind Z axis and stability Z axis as they're the same for CL, but only stability X axis for CD.

Here's the definition by NASA TM 107601:

By DTIC ADA 189675:

Assuming the thrust is not deflected and is along the body X axis, then you can cast the thrust and weight onto the stability frame:

• Forces on the stability Z axis: Lift + Thrust * sin(α) - Weight * cos(pitch-α)
• Forces on the stability X axis: Thrust * cos(α) - Drag - Weight * sin(pitch-α)

Answer 9

Upon further consideration, I concur with the Answers concluding that, in a climb, Lift is less than Weight.

The applicable formula which appears in several engineering sources indicates that, in a climb: Lift = Weight X Sine(Climb Angle) and Thrust = Drag + Weight X Sine(Climb Angle) (See, e.g., this document.) Since the Sine is always less than 1 in a climb, Lift is always less than Weight in a climb. (Edit: Deleted reference to "vertical" Lift. The formula relates to total Lift since you want Lift = 0 in vertical climb.)

My initial Answer was that Lift is (of course) greater than Weight in a climb. This seemed to be "common sense" and was based on what I was taught during flight training. For example, according to the FAA "When an airplane enters a climb, excess lift must be developed to overcome the weight or gravity. This requirement to develop more lift results in more induced drag, which either results in decreased airspeed and/or an increased power setting to maintain a minimum airspeed in the climb." [Airplane Flying Handbook (FAA-H-8083-3B), p. 3-16]

It turns out that the FAA analysis is only partially correct. The real reason for increasing Lift is not to climb, but to change the flight path of the aircraft to match the desired Climb Angle. Once that is done, the aircraft is achieving most of the desired Climb Rate because the aircraft is now flying upwards. The FAA statement fails to mention that the main reason you need to add power is to maintain speed to offset the effects of gravity while you "climb the incline".