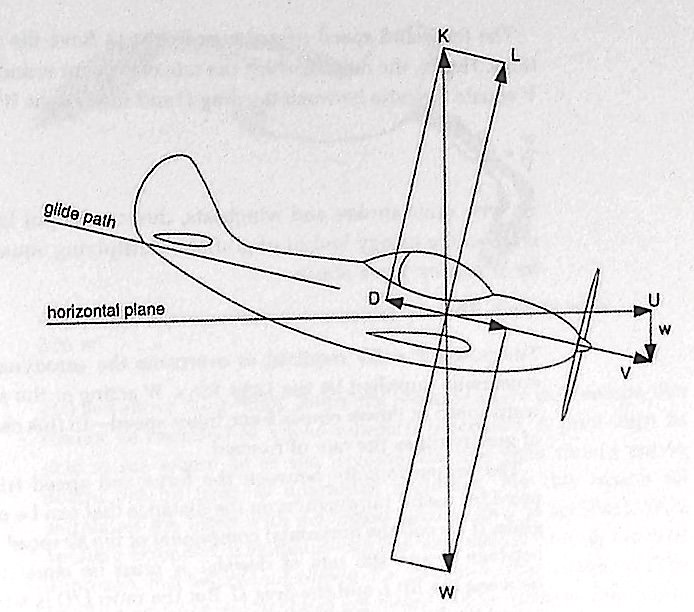
A glider gets the power it needs to fly from the decrease of gravitational potential energy associated to the descent. My question is: for the same weight $W$, airspeed $V$, and a prop efficiency of 100%, is the amount of ‘gravitational power’ $W · w$ that drives a plane in an unpowered glide exactly identical to the ‘engine power’ it needs for s/l, unaccelerated flight?
Short answer yes, a powered aircraft at any given airspeed in straight and level flight (note that a glide path is also "level", at constant airspeed) needs to use the same amount of energy to over come drag as the glider.
The potential energy for the glider is altitude. The potential energy for the powered aircraft is fuel. The conversion of potential energy to kinetic energy gives the aircraft velocity and matches drag forces.
There for, gliding is powered flight! They might "fill up" by catching a thermal, or by having someone tow them up.
The power applied by any given source to a moving vehicle is always equal to the force component exerted by that source in the vehicle's direction of travel, times the velocity of travel.
In steady-state flight, whether we are gliding or flying or horizontally under power, the Drag vector must be opposed by some other force. In steady-state horizontal powered flight, assuming that the thrust line is exactly parallel to the flight path (as seems to be implied by the question), the Thrust from the motor exactly opposes Drag. Hence the power applied by the motor equals Thrust * airspeed, which is also equal to Drag * airspeed.
What is the power applied by gravity to an aircraft in gliding flight?
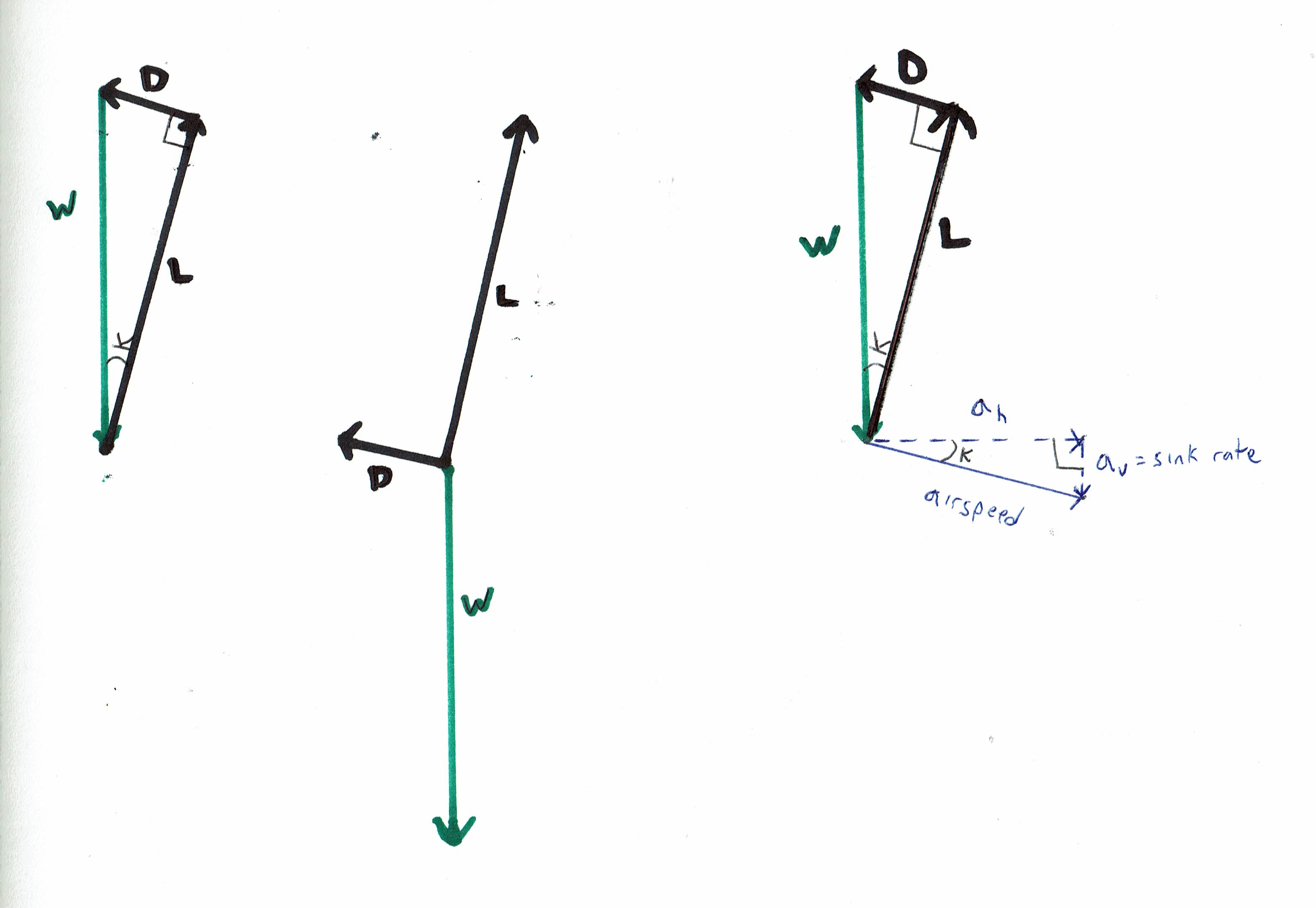
See the three diagrams representing gliding flight illustrated immediately below. These are three different ways of depicting the same situation-- a steady-state glide at one particular L/D ratio. On the right-hand diagram, we've included the velocity (airspeed) vector, and its horizontal and vertical components. The vertical component of the velocity vector is the sink rate. The force vector triangle and the velocity vector triangle are geometrically similar, each having one corner with a 90-degree angle, and one corner equal to the glide angle.
In gliding flight, the net power applied by gravity to the aircraft will be equal to the airspeed times the component of the Weight vector that acts in the direction of the airspeed vector. The component of the Weight vector that acts in the direction of the airspeed vector is Weight * (cosine glide angle), so we can say that the power applied by gravity is airspeed * Weight * (cosine glide angle). But airspeed * (cosine glide angle) is the sink rate. So the power applied by gravity is Weight * sink rate.
Since Drag is the only aerodynamic force that has a component parallel to the airspeed vector-- in fact, the Drag vector is exactly parallel to the airspeed vector-- we can say that all the power applied by gravity to the gliding aircraft is acting to overcome Drag. It is exactly analogous to the power applied by the motor in level flight. In gliding flight, the magnitude of the Drag vector is exactly equal to (Weight * cos glide angle). In other words, in gliding flight, the magnitude of the Drag vector is exactly equal to the component of the Weight vector that acts in the direction of the airspeed vector.
If we could transition from gliding flight to powered flight with no change in airspeed and no change in the magnitude of the Drag vector, then the power required would stay exactly the same. The source of the power would simply change from gravity to the motor. The power required in horizontal flight would equal airspeed * Weight * cosine glide angle, which is also equal to Weight * sink rate.
For reasonably high L/D ratios (glide ratios), it's a very good approximation to say that the scenario described above is true. But in reality, the truth is that when we transition to from gliding flight to powered flight, the Lift vector must become slightly larger. This means that we must either increase the angle-of-attack and change the lift and drag coefficients, or we must increase the airspeed. Therefore it is not exactly true to say that the power required in horizontal flight equals Weight times the sink rate that we see in gliding flight at the same airspeed, or at the same angle-of-attack.
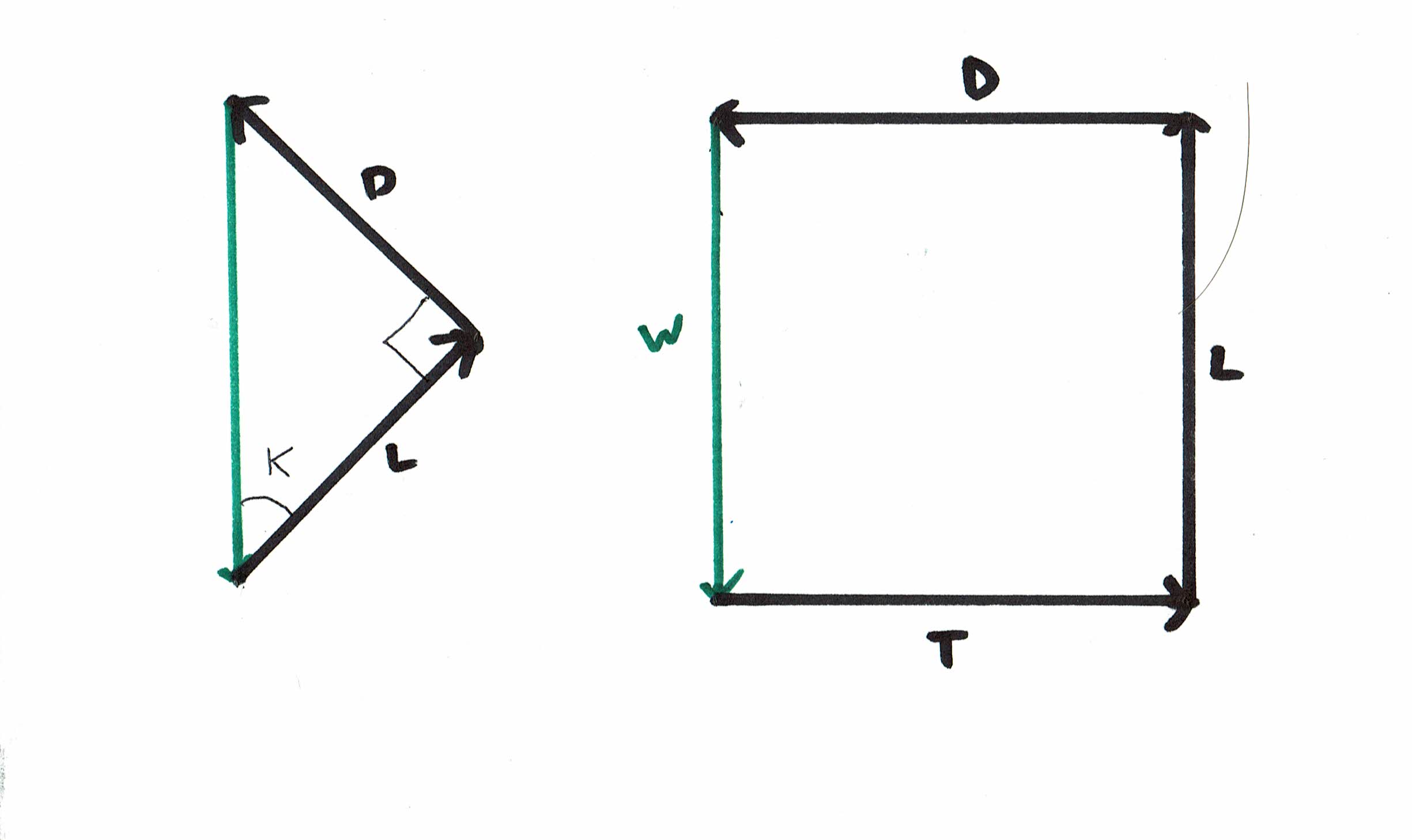
Let's explore this some more with some vector diagrams. To make things clearer, we'll take the case of an aircraft with a very poor L/D ratio of one to one.
The left-hand diagram shows the gliding case. Note that the Drag vector bears a significant amount of the aircraft weight, which "unloads" the wing. The Lift vector and the Drag vector each are equal to .71 * Weight. When we transition to powered flight, we'll hold the angle-of-attack constant, which means that the lift and drag coefficients will stay constant, which means that the Lift/Drag ratio must stay constant. The only way we can make a closed polygon (in this case a square) out of the Lift, Drag, Weight, and Thrust vectors, while preserving the same 1/1 L/D ratio that we had in gliding flight, is to increase the size of the Lift and Drag vectors, so that the Lift vector (and in this case, also the Drag vector) becomes equal in size to the Weight vector. Since we haven't changed the angle-of-attack, this can only mean that we've increased the airspeed. At this particular L/D ratio, the airspeed associated with gliding flight is lower than the airspeed associated with horizontal powered flight by a factor of (square root of .71) = .84. Both the increased airspeed and the increased Drag vector cause an increase in required power in horizontal flight, compared to the power exerted by gravity in gliding flight at the same angle-of-attack.
In this particular (admittedly extreme) case, the power required for horizontal flight is greater than (weight * sink rate) by a factor of (1/ (.71 * .84)) = 1.68, where "sink rate" means the sink rate in gliding flight at the same angle-of-attack as we have in the powered case.
More generally, the power required for horizontal flight works out to weight * sink rate * / ((cosine ( arctan (D/L)))^1.5), where "sink rate" means the sink rate in gliding flight at the same angle-of-attack as we have in the powered case.
For comparison, here is a table of the increase in power required for horizontal flight beyond the value predicted by (weight * sink rate), for various L/D ratios, where "sink rate" means the sink rate in gliding flight at the same angle-of-attack as we have in the powered case--
L/D 1/1 -- power required for horizontal flight is greater than weight * sink rate by a factor of 1.68
L/D 2/1 -- power required for horizontal flight is greater than weight * sink rate by a factor of 1.18
L/D 5/1 -- power required for horizontal flight is greater than weight * sink rate by a factor of 1.030
L/D 8/1 -- power required for horizontal flight is greater than weight * sink rate by a factor of 1.012
L/D 10/1 -- power required for horizontal flight is greater than weight * sink rate by a factor of 1.0075
Clearly, for most purposes this effect can be considered to be negligible for L/D ratios above 5/1 or so. But the question did ask whether the power required in the gliding and powered cases was exactly identical.
The original question was phrased to hold airspeed constant between the gliding and powered cases, not angle-of-attack. To hold the airspeed constant as we transition from the gliding case to the powered case, we must increase the angle-of-attack to increase the lift coefficient to provide the extra lift needed in the powered case. Now the L/D ratio is almost certainly not remaining constant. Since airspeed is now constant, the change in the required power will be directly proportional to the resulting change in the Drag vector. And the resulting change in the Drag vector depends upon where we are on the L/D versus airspeed curve. If we are in high-speed cruise well above the best L/D speed, the Drag vector will actually be smaller in level flight than in gliding flight. If we are flying slower than best L/D speed, the Drag vector will be larger in level flight than in gliding flight. There is one special case where we happen to gliding with an angle-of-attack slightly lower than the angle-of-attack yielding the maximum L/D ratio and minimum Drag, and as we transition to horizontal flight, we increase the angle-of-attack and end up with an angle-of-attack slightly higher than the angle-of-attack yielding the maximum L/D ratio and minimum Drag, and the net change of Drag is exactly zero.
Again, these effects will be negligible at reasonably high L/D ratios. As we transition from the gliding case to the powered case, the Lift vector only needs to increase in magnitude by a factor of 1 / ((cosine ( arctan (D/L))). This factor drops to less than 1.01 for L/D ratios higher than 7/1. The corresponding change in the Drag vector depends on where we are on the L/D curve, but it will clearly be negligible for most practical purposes.
In summary:
for the same weight $W$, airspeed $V$, and a prop efficiency of 100%, is the amount of ‘gravitational power’ $W · w$ that drives a plane in an unpowered glide exactly identical to the ‘engine power’ it needs for s/l, unaccelerated flight?
No, the power requirements are generally not exactly identical in the powered case and the gliding case, because the Lift vector must be slightly larger in the powered case, which implies that the angle-of-attack must be slightly higher in the powered case. Therefore the Drag vector is unlikely to be identical in size between the two cases. But for most practical purposes, in aircraft with reasonably high L/D ratios, the difference in Lift, Drag, and power required between the gliding and powered cases is negligible.
See also related answers to related questions:
Can we show through simple geometry rather than formulae or graphs that the best glide ratio occurs at the maximum ratio of Lift to Drag? -- a concise answer with clear diagrams
Can we show through simple geometry rather than formulae or graphs that the best glide ratio occurs at the maximum ratio of Lift to Drag? -- a longer answer that emphasizes many concepts essential to the present answer
Why is the L/D ratio numerically equal to the glide ratio? -- similar to link immediately above
Descending on a given glide slope (e.g. ILS) at a given airspeed— is the size of the lift vector different in headwind versus tailwind? -- the variation in the size of the Lift vector as the still-air glide ratio changes comes into play here, exactly as it does in the present answer
What produces Thrust along the line of flight in a glider?
An airplane has an engine that pushes its flight. What force pushes a glider to fly?
