Other answers have already pointed out that
In a steady-state descent, for the simple case where the thrust vector is considered to act parallel to the flight path, lift =weight * cosine (descent angle).
Therefore, the larger the descent angle, the smaller the lift vector must be.
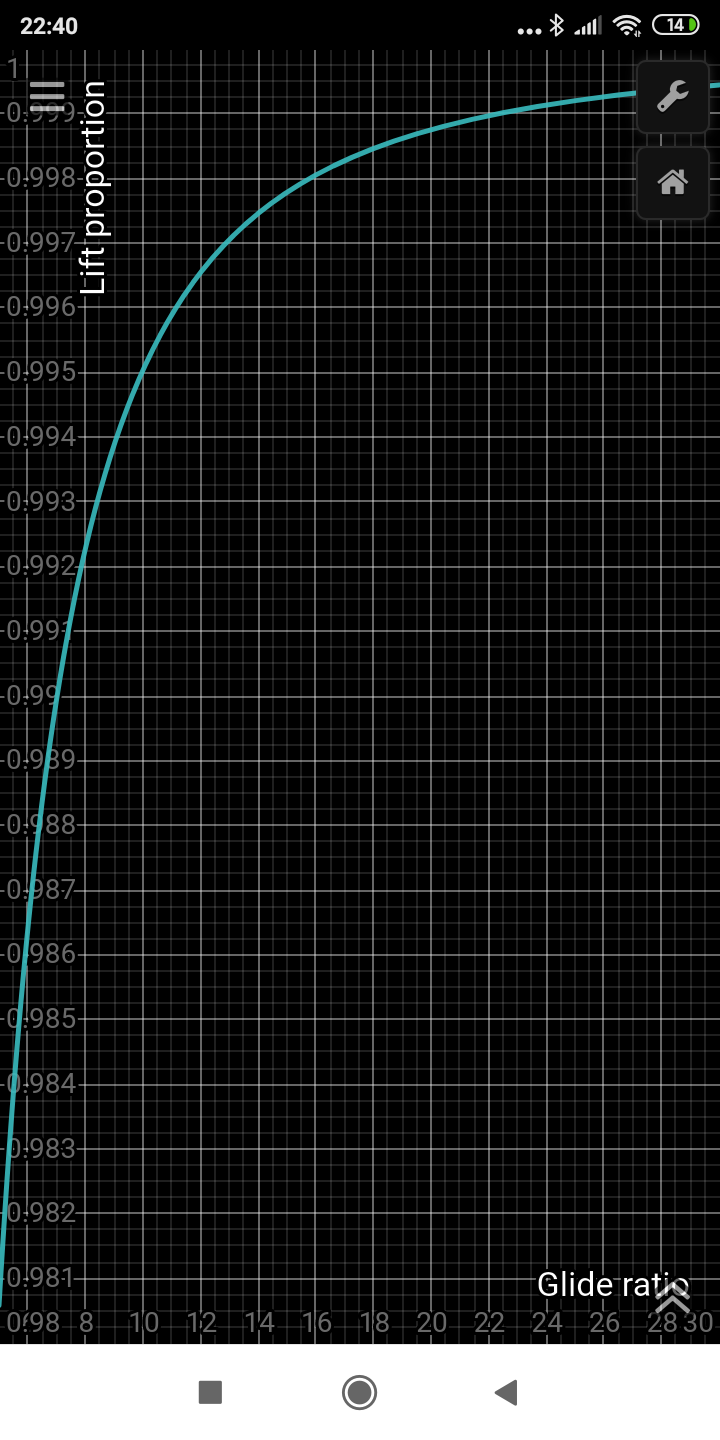
However, for reasonably small descent angles-- say 20 degrees or less-- this effect is very small, and (if we assume the thrust line acts parallel to the flight path), lift remains very nearly equal to weight.
For example, at a 10 degree descent angle, which in the context of an instrument approach would be considered absurdly steep, lift is still about 98% of weight. Going from a 10:1 descent ratio (5.7 degree descent angle) to a 5:1 descent ratio (11.3 degree descent angle), we very nearly double the sink rate (if airspeed is constant), but the lift vector only changes from 99.5% of weight to 98.1% of weight, a change of about 1.5% (i.e. the ratio between the two numbers is about 1.015).
Things are different at steeper descent angles, especially well over 30 degrees, where the (drag minus thrust) vector carries a significant part of the aircraft weight-- all of it, in the extreme case of a vertical dive. At steep descent angles, further increasing the descent angle does significantly reduce the magnitude of the lift force. (Also, at very steep descent angles-- above 45 degrees-- increasing the magnitude of the (drag minus thrust) vector actually decreases the sink rate, rather than increasing it.)1
As long as the descent angle is small, when we say we increase the descent angle and sink rate "by reducing lift" -- e.g. by opening spoilers-- it is technically true that the lift force is slightly reduced, but if we are envisioning a significant effect, what we really mean must be something else entirely.
In some cases, we might really mean that we are reducing the lift coefficient. The descent angle is determined by the ratio of Lift to (Drag minus Thrust), which in the power-off case, is exactly equal to the ratio of the lift coefficient to the drag coefficient. This gives some insight into how reducing the lift coefficient as well as increasing the drag coefficient, can help us to descend more steeply.
However, we have to be careful about how we apply this idea as well, for at least a couple of reasons. One, because it gives no insight into how we can descend by deploying flaps, or by reducing thrust. And two, because it gives no insight into any case where we are holding the airspeed constant. Whenever the airspeed is constant, the lift coefficient and the lift force are directly related, so the lift coefficient must be directly related to weight * cosine (descent angle). For shallow descent angles, we've already noted that the value of this expression only decreases slightly even when we make a large increase in the descent angle and sink rate.
So what is really going on when we increase our descent angle and sink rate by deploying spoilers, or deploying flaps, or reducing power, while holding airspeed constant?
If we deploy the flaps, this will have the initial effect of increasing the lift coefficient as well as the drag coefficient. If we don't want to lose airspeed, we'll need to decrease the angle-of-attack of the wing to return to (very nearly) the original lift coefficient. Decreasing the angle-of-attack in this manner slightly reduces the net increase in drag coefficient that would otherwise be created by deploying the flaps, but the drag coefficient is still higher than it was before we deployed the flaps. Unless the airspeed is quite low, we have more drag force and a higher descent angle and a higher sink rate when the flaps are down than when they are up. The higher the airspeed, the more pronounced this effect. When the flaps are deployed to a very large deflection, such as 45 degrees or more, lowering the nose to increase the airspeed can be a very effective way to create a very large increase in the descent angle. Another way to say this is to note that deploying the flaps creates a very large downward shift (toward a higher sink rate) in the far right-hand portion of the "polar curve" of airspeed versus vertical speed.
If we increase the descent angle by opening the spoilers, this will have the initial effect of reducing the lift coefficient as well as increasing the drag coefficient. If we don't want to gain airspeed, we'll need to increase the angle-of-attack of the wing to return to (very nearly) the original lift coefficient. The increase in angle-of-attack also further increases the drag coefficient. The net result on the sink rate and descent angle is somewhat similar to if we had deployed the flaps, except that there is no decrease in stall speed. Rather, the stall speed is raised.
(Note that increases or decreases in angle-of-attack (as measured at the fuselage or the unflapped part of the wing) do not directly correspond to identical increases or decreases in pitch attitude, especially if we're simultaneously making a change in configuration, which also affects the flight path. Note also that for simplicity this answer assumes wing area is constant-- the increased wing area due to deploying Fowler flaps, for example, is just treated as an increase in the lift and drag coefficients. Note also that throughout this answer, for simplicity we treat the thrust vector as acting parallel to the flight path through the airmass.)
At the end of the day, while flying at any given constant airspeed, for shallow descent angles, we increase our descent angle and our descent rate by reducing thrust or by adding drag. The closed vector diagram of Weight, Lift, and (Drag - Thrust) does force a small decrease in the value of the Lift vector whenever we increase the descent angle, but for shallow descent angles, this effect is very small.
Note that if we are operating on the "front side" of the thrust-required curve (i.e. not at extremely low airspeeds), simply moving the control stick forward is another way to add drag, even though the drag coefficient is actually reduced by the decrease in angle-of-attack. What we're really doing here is reducing both the lift and drag coefficients, which leads to a higher airspeed, which (for small descent angles) restores the lift force to (very nearly) its original value, but at cost of a net increase in drag. So the descent angle and sink rate are both increased.
Footnotes:
- Equation needed, to prove that this statement is true, for a given constraint (e.g. constant lift coefficient? Or constant descent angle?)
Related ASE questions--
Descending on a given glide slope (e.g. ILS) at a given airspeed-- is the size of the lift vector different in headwind versus tailwind?
(this answer contains vector diagrams relevant to the present answer, and also includes (near the end) a discussion of effects arising from the thrust line not being parallel to the flight path)
'Gravitational' power vs. engine power -- the variation in the size of the Lift vector as the flight path changes from horizontal to descending comes into play here, exactly as it does in the present answer
Does lift equal weight in a climb?