First of all, note that the diagram linked in the question is erroneous. The equations may be right, but the forces are drawn in the wrong proportion-- lift is erroneously shown as greater than weight, when it should be less than weight. Also thrust appears to be about equal to drag, when it should be distinctly greater than drag. Only when lift is less than weight and thrust is greater than drag can we build a closed vector triangle-- meaning that net force is zero-- from weight, lift, and (thrust minus drag ). For more, see this answer to a related question Does lift equal weight in a climb? . (If you want to see a similar diagram from an outside source, see the one included in this answer to a related question Is excess lift or excess power needed for a climb? )
Just as NASA also messes up the proportions in this diagram for gliding flight-- https://www.grc.nasa.gov/www/k-12/airplane/glidvec.html -- again, lift is shown as being greater than weight, when it should be less than weight, so we can build a closed vector triangle from weight, lift, and drag. For more, see this answer to a related question What produces Thrust along the line of flight in a glider?
Now as to your questions-- to a first approximation we can think of our pitch control inputs-- the position we are placing the control stick or yoke in, in the fore-and-aft direction-- as most directly governing angle-of-attack, not pitch attitude in space. Pitch attitude in space is influenced by the climb angle which is influenced by power setting. Now, there are all kinds of inter-relationships that complicate things-- for example on a high-wing plane with flaps down, adding power may produce a strong downwash over the tail that tends to lead to an increase in angle-of-attack. But to a first approximation we can think of our pitch control inputs as governing angle-of-attack. There is a slight delay between a change in pitch control input and a change in angle-of-attack, due to the aircraft's rotational inertia in the pitch axis.
If I tell you that I've increased my aircraft's pitch attitude by 10 degrees but I don't tell you what I did with the elevator control to make that happen, nor do I tell you whether I've added power or not, then you have no way to guess whether I've kept the angle-of-attack constant and started climbing due to added power, or I've managed power as needed to keep altitude constant while transitioning to a higher angle-of-attack and lower airspeed, or any number of other possibilities-- I might even have put the stick or yoke forward to decrease the angle-of-attack and still added enough power that the aircraft transitioned into a climbing flight path resulting an in increase in pitch attitude. For example when a jet fighter aircraft is climbing vertically on raw thrust alone, the control stick is probably forward of the position where it would be during horizontal flight at the same airspeed. Certainly the angle-of-attack is lower in the vertical climb, than in horizontal flight at the same airspeed!
From the standpoint of what is really going on physically with the aircraft, most flight training curricula vastly over-emphasize the idea that the pilot is directly controlling the aircraft's pitch attitude. What he's really doing is controlling angle-of-attack and power setting. Yet the former way of looking at things works well enough in actual practice (e.g. flying an ILS glide slope by referring to an attitude indicator rather than an angle-of-attack meter as the primary guide to pitch control) and is simpler to think about.
A key point is that our flight operations are usually conducted on the "front side of the power curve", where for a given power setting, an increase in angle-of-attack usually results in an increased climb rate and an increased climb angle. Therefore moving yoke or stick aft results in an increased angle-of-attack AND an increased climb angle (or a decreased glide angle) AND an increased pitch attitude. On the "back side of the power curve", like just above stall speed, an increase in angle-of-attack will generally lead to a decreased climb angle or increased sink angle, and the aircraft will end up in a more nose-down pitch attitude, so the idea that we're somehow directly controlling pitch attitude with the control yoke or stick no longer works very well.
Your question indicates a desire to better understand some of the physical relationships at play. Lift is proportional to (lift coefficient * airspeed squared). Lift coefficient is determined by angle-of-attack, with higher angles-of-attack creating higher lift coefficients. As shown in the vector diagrams attached to the two links given at the start of this answer, for shallow to moderate climb or dive angles, lift is NEARLY equal to weight. Actually, lift is a little less than weight unless the flight path is exactly horizontal, but for shallow to moderate climb or dive angles, the difference is small. Since weight is staying constant, we can conclude that for shallow to moderate climb or dive angles-- with no other accelerations going on (airspeed is staying constant or changing only slowly, and the flight path isn't curving up or down, and the wings aren't banked so the flight path isn't curving to describe a turn), lift is also staying nearly constant. This means that for shallow to moderate climb or dive angles, airspeed ends up being a pretty good guide to angle-of-attack-- to keep lift nearly constant, if the airspeed is low, the lift coefficient and angle-of-attack must be high. So the airspeed indicator is in essence an angle-of-attack gauge. At very steep climb angles where lift is quite a bit less than weight, things get more complicated-- if the aircraft is climbing straight up, lift must be zero, so lift coefficient must be zero, and angle-of-attack must be nearly zero (actually it must be slightly negative, unless the airfoil is completely symmetrical), no matter what the airspeed indicator reads.
In actual practice in general aviation, commercial aviation, etc, a shallow to moderately steep climb is NORMALLY carried out a higher angle-of-attack and lift coefficient--and therefore a lower airspeed-- than we'd use for high-speed cruising flight. It's more efficient this way, and it also gives us the most climb performance out of a given, limited amount of thrust available. Why? Because a high lift coefficient also correlates with a high ratio of (lift coefficient to drag coefficient), which means a high ratio of lift to drag. For shallow to moderate climb angles, the higher the L/D ratio we can achieve, the steeper we can climb for a given amount of thrust. This is explored in more detail in the first link given in this answer. To look at climb rate rather than climb angle, we'd have to look at a chart of (power-available minus power-required) at various airspeeds or various angles-of-attack, but we'd come to a similar conclusion-- our best climb performance will be achieved at an angle-of=attack well above what we'll be using in high-speed cruising flight.
The diagram in the original question doesn't touch in any way on the relationship between airspeed, angle-of-attack, lift coefficient, magnitude of lift vector, and L/D ratio, so it doesn't help us to understand why a shallow to moderately steep climb is normally carried out at a higher angle-of-attack than we'd use for high-speed cruising flight.
Your question included the statement "if I pitch the airplane up, but also increase power and am able to maintain the same speed, then no, the AoA hasn’t changed, although it may have varied in the transition between one situation and the other." For shallow to moderate climb angles, your statement is true for all practical purposes, but it is not EXACTLY true. If we want to be very precise about it, we could note that since lift is slightly reduced in the climb, if airspeed stayed constant than angle-of-attack must have been slightly reduced, and if angle-of-attack stayed exactly then airspeed must have been slightly reduced. This same idea came up in these two related answers to related questions, though in these cases the lift vector was reduced because the aircraft was in a descent rather than a climb -- 'Gravitational' power vs. engine power and Descending on a given glide slope (e.g. ILS) at a given airspeed-- is the size of the lift vector different in headwind versus tailwind?
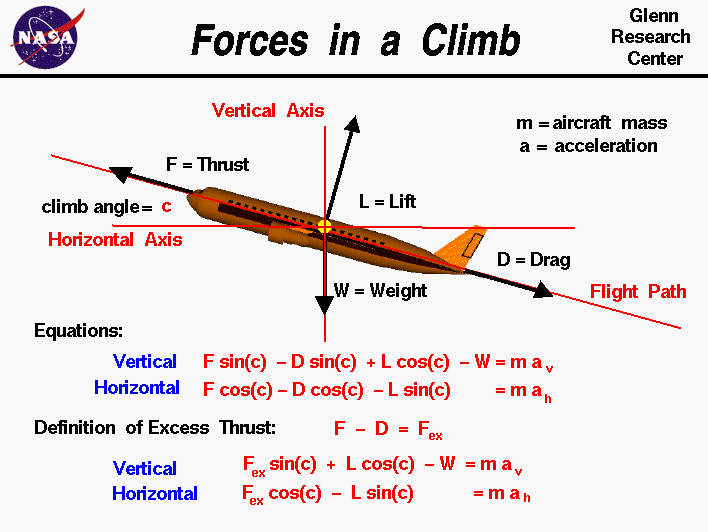Please explain in reference with these forces ( in the pic )
