How can an airplane keep going forward if it has no thrust?

Question

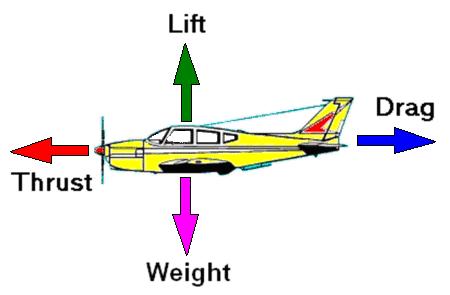
Every pilot is familiar with the basic model of aircraft forces:

If lift is greater than weight, the plane climbs, if less, descends. If thrust is greater than drag, the plane accelerates, if less, decelerates. In unaccelerated flight, all must be equal.

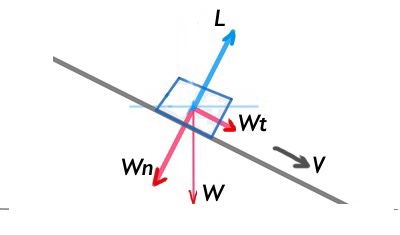
In an engine-out situation, there is no thrust, so if a constant glide ratio is to be maintained, I thought the plane must be pitched forward so the forward component of lift does the job thrust would normally do in cancelling drag.

However, in the Airplane Flying Handbook, 4-3, figure 4-2, it shows an example lift-drag diagram that shows $L/D_{max}$ ($= V_{BG}$) to be about $6°$ AoA. Wouldn't that result in lift having a backwards component and slowing down the aircraft to the point of stall?

What am I missing here?

Answer 1

Angle of attack is not equal to pitch angle. Yes you can decompose weight into a forward and a perpendicular component - to airstream! Or you can draw lift relative to airstream.

In this drawing, pitch angle is zero and AoA = 6 deg. Lift now has a forward component relative to gravity.

Answer 2

If at zero airspeed and zero absolute altitude this were to happen, then yes the aircraft would stall and be incapable of flying. Then again, at this point, it would not matter, AS THE AIRPLANE IS ALREADY ON THE GROUND IN THIS STATE.

In all seriousness, though, the airplane would not slow and stall unless you attempted to remain at a constant altitude. In a constant airspeed glide, however, where the airplane is descending at a shallow angle relative to the ground, it can be shown that the force of gravity is no longer perpendicular to the longitudinal axis of the aircraft and will have a component vector which is parallel to the longitudinal axis and opposed to the direction of both the induced and parasite drag vectors.

Another way to think of this is to consider the energy state of the airplane at the time the engine quits. Its total energy is the sum of its potential energy, proportional to its absolute altitude, and its kinetic energy, proportional to the square of the groundspeed. Once engine thrust is lost, that energy state begins to be drained away by the force of drag applied over a distance. In order to maintain airspeed, the aircraft must begin to convert its potential energy into kinetic energy, causing the airplane to descend slowly until it reaches the ground.

Answer 3

The weight $W$ of the glider has two components, $Wt$ and Wn. $Wt$ is in the same direction as $V$ and $Wn$ is perpendicular to $V$. Aerodynamic $F$ also has two components $L$ and $D$, where $L$ is perpendicular to $V$ and $D$ is parallel to $V$.

When $L = Wn$:

$Wt> D$, the linear acceleration of the glider is positive; $Wt <D$, the linear acceleration of the glider is negative; $Wt = D$, the linear acceleration of the glider is zero.

Some people think that there are only $L$, $D$, and $W$ on the glider. This is not entirely correct. Simply put, there are only two forces on the glider: one is aerodynamic $F$, and the other is gravity $W$. $L$ is only the component of $F$ in a direction perpendicular to $V$; $D$ is only the component of $F$ in a direction parallel to $V$. Some people think that $Wn$ does not exist, which is also incorrect. $Wn$ is the component of $W$ in a direction perpendicular to $V$; $Wt$ is the component of $W$ in a direction parallel to $V$.

What pushes the block downward? $V$ is the speed at which the block moves downward. The weight of the block has two components: Wn perpendicular to $V$ and Wt parallel to $V$. $L$ is the reaction force of the slope (inclined plane) on the block. It is not the $L$ push block that moves downward, because $L$ has no component in the direction of $V$. Wt is the force that pushes the block downward.

Answer 4

Here is a simple way to think of this problem:

Without thrust from its engine, the airplane begins instead to "coast downhill" like someone on a bike- and the pilot can choose whatever "slope" he or she wishes to follow, consistent with the need to keep the wings generating lift and the control surfaces to generate directional control forces.

In a gliding descent like this, the weight of the plane times its descent rate in feet per second yields the horsepower being burned off by drag at that combination of pitch attitude and airspeed.

So in unpowered flight, the airplane flight manual will specify the best glide angle for a given load which minimizes drag and hence maximizes the time aloft- and thereby furnishes the pilot with the best opportunity to pick out a suitable landing spot.