I recently checked about the max takeoff weight of an A380 and the thrust it can provide at TO/GA power and I was quite shocked by the results. The total thrust by the engines was only about 127000 kgs whereas its max takeoff weight was 560,000, almost 4x the thrust by engines.
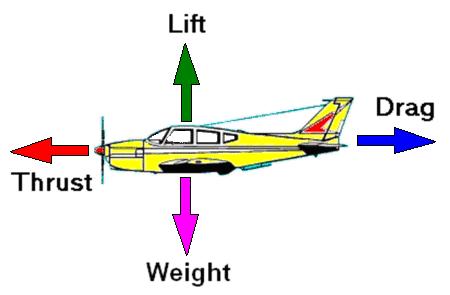
A wing's shape allows it to deflect a large volume of air downwards while incurring a small amount of drag, which in turn means a relatively small amount of thrust (compared to the weight of the plane) is required to push it forwards. The critical value involved is the lift-to-drag ratio, which varies based on a number of factors but might be 60:1 for gliders or 20:1 for airliners.1
Perhaps an intuitive way to understand how this is possible is with a diagram. In this simplified representation, the wing is the gray box and it's moving to the right, so the blue arrows represent the direction the air is flowing relative to it.
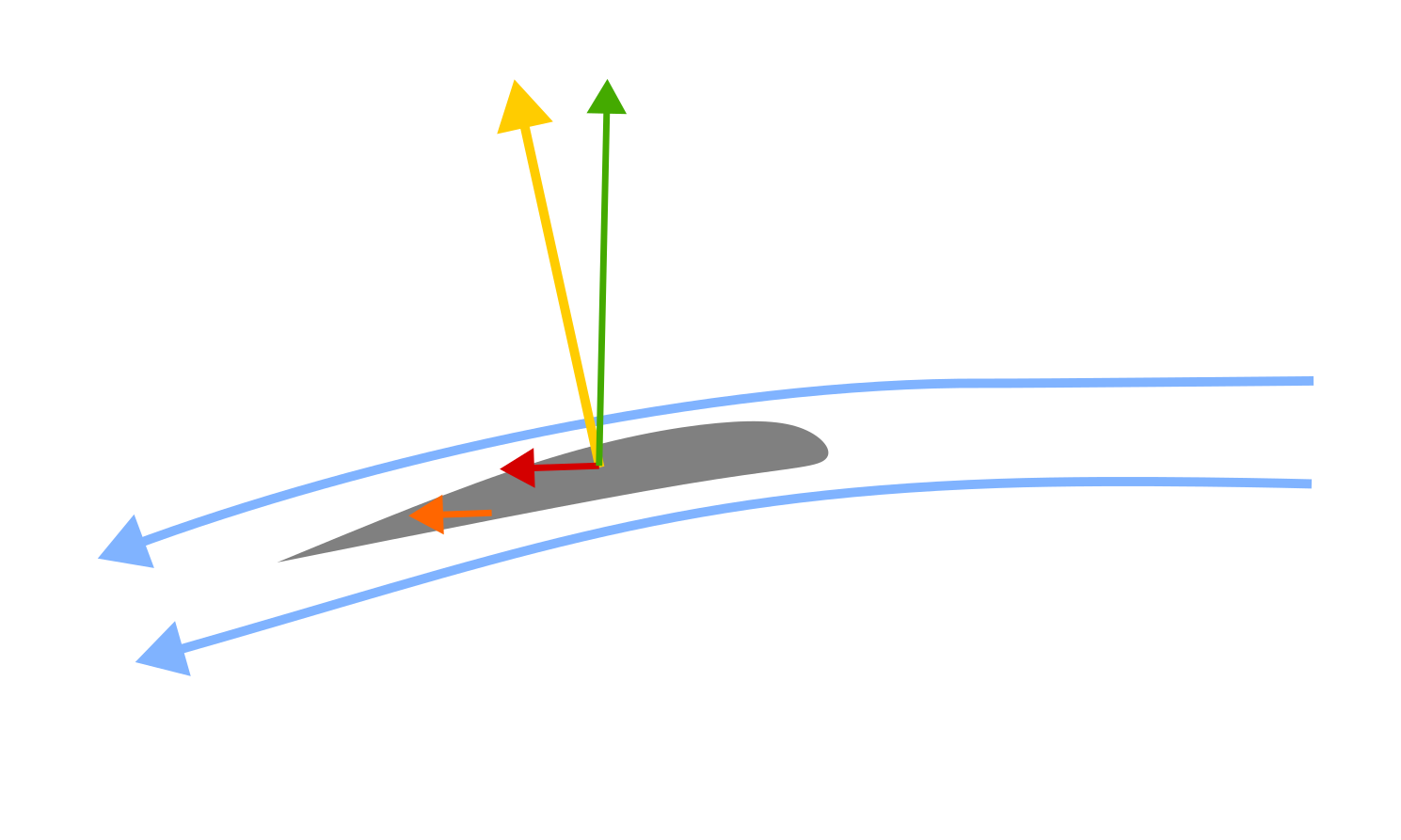
As the wing moves through the air at an angle, it will produce a force perpendicular to itself (the yellow arrow). At low angles of attack (that is, the angle between the direction the wing is pointing and the direction the plane is moving), you can see that the vertical component (lift, colored green) is much greater than the horizontal component (lift-induced drag, red). There some additional "parasitic" drag created even if the wing is pointing straight into the wing (orange arrow), but even if you add this onto the induced drag, the lift is still much greater than the total drag.
Now for your second question: If you increase the wingspan (and in turn wing area), you will increase the lift at a given airspeed, but you will also increase the drag as there is now more wing area that has to be pushed through the air. This will reduce the top speed of the aircraft but will probably allow it to fly at a lower airspeed. The ratio of lift to drag actually will improve slightly due to what happens when aspect ratio (the ratio of wingspan to the distance from front to back of the wing) is increased - see here for a detailed explanation. This is the reason for gliders having extremely skinny wings.
Answering your third question is tricky, as it depends on a number of factors, but my intuition says increasing the wingspan should result in less power required to get off the ground, up to a point. Longer wings are weaker, so they will need more structural weight to support them. There will come a point where the extra lift generated by adding more wingspan will not be worth the weight and drag caused. I suggest an optimized design would be something along the lines of human-powered aircraft like the Gossamer Albatross, as these have to work around the low power-to-weight ratio of humans.
1. These numbers are actually lift-to-drag ratios for the whole plane (so including the drag from the body), so the lift-to-drag ratio of the wing itself is actually a little bit better. The ratio for the whole plane is what matters when trying to calculate how much thrust it needs to make a certain amount of lift, so that's why I've used it here.
Lift is produced when the wings push air down. Doing so inevitably involves some drag that would tend to slow the airplane down or force it to lose altitude. The engine only needs to provide enough thrust to counteract drag, which is generally several times less than the lift.
Yes, flying airplanes with small motors and extremely long wings is possible. The downside is that these airplanes tend to be slow. Some extreme examples are the MIT Daedalus (where the "engine" is a person) and Solar Impulse (a solar plane with a wingspan similar to an A340, but some 100x lighter).
Some people even fly gliders, essentially airplanes with no engine at all, for long distances!
Just to complement a bit the other answers.
How can the wing generate more lift than the thrust?
The question is based on the false premises that:
Regarding 1.:
Lift can be bigger than thrust but it doesn't have to. The ratio 1:4 that you've calculated is absolutely no fixed value and it's related to the type of mission that the airplane has to fly more than to the physics behind. A value 1:4 till 1:5 is quite typical for a jetliner but a value 1:1 (or even higher - more than 1.25:1 can be reached by a F-22 for example) is more typical for a jetfighter: for a jetfighter your original question is therefore just the other way around. And a glider doesn't even have a proper thrust source so that that ratio just become infinite.
Regarding 2.:
The total thrust by the engines was only about 127000 kgs whereas its max takeoff weight was 560,000, almost 4x the thrust by engines. How does this make sense?
This totally makes sense if you consider how the four main forces act upon an airplane (picture from this question):
As you can see, thrust wins drag and lift wins weight and in general for an airplane there is no direct coupling between lift and thrust: if your airplane had twice as much drag, then the thrust generated by the engine should simply be twice as much but weight and lift wouldn't change. Or, if your airplane were 20% lighter then the lift should simply be 20% smaller but drag and thrust wouldn't change.
Obviously the whole story is not plain black and white as just described: in my first example, if drag doubles then thrust must double, then the engines become bigger and heavier, then weight increases, then lift increases, then drag (due to lift) increases and then we've just entered in a vicious circle. Anyway the general idea that weight and thrust are acting in two different directions holds true.
You are able to pull a sled or a cart way heavier than yourself, uphill, pulling it with a force lower than its weigth. Of course, if the path is way too much inclined, you will fail.
It is the inclined path that helps. I will skip the geometry pictures here as everyone has the intuitive understanding on the matter.
In the aviation context, it is the pilot who decides the inclination of the climb by controlling the pitch angle (how much the nose is up or down) and the control surfaces positions.
At a speed, the air under the wing behaves as a snow surface that is generally as much inclined as the wing is. Happy climbing!
The airplane behaves as a sled when going downhill as well, it gains speed and one has to deal with the unwanted speed in order to, e.g. land safely.
