Is it right that basically an airplane just needs to accelerate to climb?
Greater velocity of an airplane leads to greater lift - and since its weight remains constant (or even decreases) - a greater lift leads to a gain in altitude without the need to pitch. But why do airplanes climb by "pointing the nose up"? Is it to climb as fast as possible and to need less horizontal space?
A climbing aircraft needs less aerodynamic lift than in horizontal flight, not more.
Now I have your attention, I hope. The reason is quite simple:
Lift equals weight, and just because the pilot chooses a different flight path angle, the weight of the aircraft does not change. The total of all lifting forces must still balance the weight, but in climb you get a small lifting contribution from the engine(s) because its (their) thrust will point upwards just like the rest of the airframe.
Don't let the many arrows and greek letters confuse you. To be in equilibrium, lift (L, dark blue), drag (D, red), thrust (T, green) and weight (m⋅g, black) must add up such that they can be combined into a closed run of vectors. I've done this with the lighter-colored vectors around the weight. Since the flight path points upwards, so does the thrust which now has a small vertical component. The lift vector can be a little shorter now.
Consider the extreme case of vertical climb: Now all thrust supports the weight, and aerodynamic lift is no longer needed.
There is a second, much more subtle effect: When you climb, air gets thinner and engine performance goes down proportionally. At the same indicated air speed, the aircraft will continually decrease its climb speed, and this deceleration frees up a tiny inertial force, which again adds to lift and counteracts weight.
Conversely, at the beginning of a climb phase the aircraft needs to create momentarily more lift to accelerate itself upward. Only then, when climb speed increases, lift must be bigger than weight to overcome the inertial effect which at this moment works downwards. For the supernerds: If you integrate the lift deficit over time of the aforementioned effect and the extra lift over time for climb acceleration, both cancel exactly.
To answer your question directly: To climb you need to increase excess energy, not speed. This is normally done by increasing engine power output, or by trimming the airplane at a lower speed where drag is lower, so more power remains for climbing. This question contains more details on how to get an aircraft to climb. Note especially @SteveV.'s bucket analogy.
If you use the airplane's kinetic energy as its source of thrust, the same mechanism can be applied to instationary climbs, where speed is traded for altitude, like in gliders.
The nose-up attitude is simply the result of a different flight path. Since the required aerodynamic lift will be almost the same, the angle of attack will also be almost the same and the whole aircraft needs to fly nose-up. This is similar to a car which has the same attitude towards the road, but when you drive uphill, both car and road will be tilted upwards.
This analogy breaks down when you change speed - flying at lower speed needs more angle of attack to still create the same lift, and this nose-up change will be added to your attitude angle.
Consider the relative airflow. When an airplane is not climbing, the relative airflow is horizontal, and so the angle at which the air meets the wings, ie the angle of attack, is measured from the horizon (Case A in the diagram). However, when an air-plane is climbing, the relative wind is tilted downward by the climbing component of the airplane's velocity. If the airplane did not tilt the nose up, the angle of attack would approach zero as the rate of climb increased, reducing lift and efficiency (Case B), so the air-plane must tilt up the nose to keep the angle of attack in an efficient range (Case C). !
While the answer from @Peter Kämpf is all true and sound, i think it misses a point and does not really answer the OP's primary question.
Is it right that basically an airplane just needs to accelerate to climb?
Yes this is basically right. Higher horizontal speed produces more lift so it'll make the aircraft climb. https://www.grc.nasa.gov/www/k-12/WindTunnel/Activities/lift_formula.html
But it's not the only way to make an aircraft climb. Increasing pitch (while also giving more thrust) is the other and has been explained by Peter.
Which is more efficient? An aircraft is designed for optimal efficiency at cruise speed and level flight. So you may want to keep your speed within a narrow range around that optimum. Raising airspeed will also raise drag (to the square of v) see https://www.grc.nasa.gov/www/k-12/airplane/drageq.html Drag is what you absolutely want to minimize because it is energy that is completely lost (transformed into heat).
This is why increasing pitch/AoA, while maintaining airspeed constant is the better way to do. This way drag stays about the same. Of course you still need to provide more thrust (thus energy), as now part of your thrust is directed downward (and part of your lift backward), but you are converting this energy more directly to altitude, eliminating the loss in drag.
So to answer your question, yes it is possible to climb in a strict horizontal attitude by increasing airspeed, but it is more energy-efficient to climb by increasing pitch. (Thrust being increased both ways)
You can answer this question empirically.
Trim your plane for straight and level flight and set the power to Vy. Look at the AI or visual horizon, and note the pitch attitude.
Now set the power to its Vy climb setting and configure the aircraft (ball, cowl flaps, mixture, prop, etc) to climb configuration, but don't retrim the elevator. Adjust ailerons to maintain straight flight.
The airplane will pitch up by itself to its Vy climb attitude.
I do think that you must consider the type of aircraft here! If I'm a hotshot new F-22 pilot with an obnoxious thrust to weight ratio trying to intercept some baddies and I need to quickly reach altitude, you can bet I'm gonna put the nose up and go like a rocket.
But really though, it's all about velocity vectors. If you want to go up, then travel up! The engines propel in the direction of the nose. (Unless you are that hotshot F-22 pilot from before). Also consider aircraft have speed limits under certain altitudes, and also consider that ole' Bernoulli is not the only reason airplanes fly, Mr. Newton has something to say about this as well.
Because most of the lift comes from the angle of attack (AoA) of the wings. Higher AoA means more lift (up to a point).
Also most aircraft will pitch up as they increase speed due to design.
As a general rule of thumb and without a long drawn out technical explanation of how and why here is a simple answer that my 8 year old son could grasp. In straight and level flight, if you reduce power without altering the Aircraft's attitude the Aircraft will descend, conversely if you increase the Aircraft's power it will climb. Now the same Aircraft without altering its power settings if you alter its attitude by lifting the nose it will slow down hence with the same power settings in you lower the nose the Aircraft will increase its speed. So your rule of thumb is "power equals height" and "attitude equals speed". Go and take a flying lesson and try it, and you will see what I mean.
Normally in an aircraft you change your altitude using the power. If you increase power, your altitude increases. If you reduce power the aircraft descends. In both cases the aircraft is normally at a near-level pitch angle.
The reason for this behavior is that the wing is permanently tilted upward by a certain amount, called the "chord angle" or "angle of incidence". The angle is chosen so that in normal conditions, with medium power the aircraft will stay at the same altitude. If the wings were flat, the aircraft would tend to descend constantly.
The main exception to the above is when you are taking off and want to gain altitude rapidly for safety reasons. In this case, the stick or yoke is pulled back and the aircraft tilts upward and climbs rapidly. What causes this is the elevator (or horizontal stabilizer) which is located on the tail of the aircraft:
The elevator allows the pilot to change the pitch of the wings. The more wing surface that is exposed to the air, the greater the upward force. You can demonstrate this yourself by holding your hand outside the window of a fast moving car. If you hold your hand level and then tilt the leading edge up, your hand will be forced upward by the wind, and vice versa. If you tilt the leading edge of your hand down, then your hand will be forced down by the wind. The same thing happens to an aircraft.
In both cases the aircraft is normally at a near-level pitch angle. nope, not even close. you need a certain aplha to generate lift and in climb your pitch is that alpha PLUS the climb slope (gamma): see diagram in Peter's answer.
$\endgroup$
The pilot choses a different flight path. This new flight path is going higher in altitude and by that is changing the potential energy. mass*gravity *9.81*delta Hight. We need to fly slower with a lower drag and use the extra energy to climb or we need to increase the power to the propellor to overcome the change in potential energy. When the altitude is changing we need also to increase the speed because of the lower air density. The lower air density is effecting the lift and the trust the prop can deliver for a giving RPM
We can calculate the trust by looking into the force vectors Lift and Weight. When the plane change course the lift vector and weight vector who were in opposite direction are in a climb path working under a small angel, the climb rate. To counter the weight we need to increase the lift from lift r1 to a lift r2. But the result is also a vector drag r1. This drag vector is added to the drag in level flight. As a conclusion we can say that we need to increase the trust to overcome the added drag and we need to increase the lift to counter the weight.
a greater lift leads to a gain in altitude
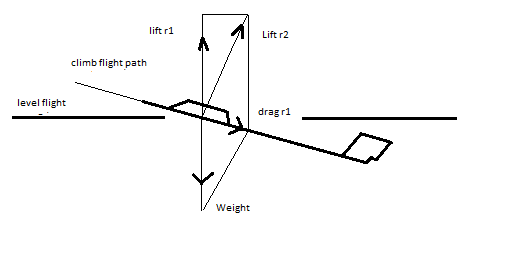
Here's where you've first gone astray. Lift is actually less than weight in a sustained linear climb. The fundamental thing that makes a sustained steady-state climb possible is that the Thrust vector is pointing upward rather than horizontally, which is only true when Thrust is greater than Drag. We'll return to this point later in this answer.
But why do airplanes climb by "pointing the nose up"?
Regardless of whether we choose to 1) climb at a high (but constant) angle-of-attack and a lower airspeed, or to 2) accelerate to a higher airspeed and climb at a low (but constant) attack, the aircraft will be somewhat nose-high in the climb because the flight path is aimed upwards, and the pitch attitude of the fuselage is the sum of the climb angle of the flight path plus the angle-of-attack of the wing minus the angle-of-incidence (i.e. the "rigging angle" of the wing relative to fuselage).
A third way to climb would be to keep the same pitch attitude that the aircraft had in level (constant-altitude) flight, but this would constrain the angle-of-attack to stay very low-- the higher the climb rate and the steeper the climb path, the lower the angle-of-attack would be forced to go. This is not the kind of feedback loop that leads to high rate of climb!
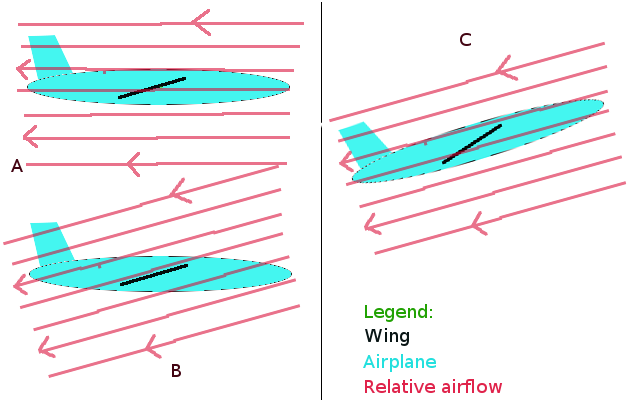
To understand why, in the artificial situation where the pitch attitude of the aircraft is constrained to be fixed, the angle of the climb path affects the angle-of-attack of the wing, you have to understand that the airflow or "relative wind" felt by an aircraft in flight is exactly opposite in direction to the aircraft's path of travel through the airmass-- which in this case is the path of the climb. (For simplicity we're assuming no wind or updraft/downdraft -- those things can change the climb angle achieved relative to the ground without changing the "relative wind" felt by the airplane, but that's not really what this question was about.) Understanding that the relative wind "felt" by an airplane is always exactly opposite to the airplane's flight path through the airmass, is one of the most important things in understanding how an airplane flies.
Therefore even in an aircraft with an unusually high angle -of-incidence like the B-52, the aircraft will be nose-high in a steep climb.
In theory an aircraft even an aircraft with zero angle-of-incidence could generate lift with the fuselage exactly horizontal. If the flight path were climbing slightly, then the wing would be flying at a slightly negative angle-of-attack, but a cambered airfoil can still create lift in such a situation. But the aircraft would generate a much higher ratio of Lift to Drag if the wing were at some higher angle-of-attack. Even though Lift is less than Weight in a climb, a high ratio of Lift to Drag is still correlated to a steep climb angle. See this related ASE answer to learn why: Does lift equal weight in a climb?
The highest L/D ratios are generated at relatively high angles-of-attack. So this is when we will see the steepest climb angle. The highest climb rate comes at a somewhat lower angle-of-attack, but the aircraft's nose will still be pitched well above the horizon, because of the simple fact that the pitch attitude of the fuselage is the sum of the climb angle of the flight path plus the angle-of-attack of the wing minus the angle-of-incidence of the wing relative to the fuselage.
Is it right that basically an airplane just needs to accelerate to climb?
No, for a steady-state linear climb at a constant airspeed, the aircraft also has to create more Thrust than Drag, and it also has to point the Thrust vector upwards.
At this point we need to revisit the paragraph beginning "A third way to climb would be to keep the same pitch attitude that the aircraft had in level (constant-altitude) flight". There is actually another problem here besides the fact that we'd be forcing the wing to fly at a very low angle-of-attack, where the L/D ratio is poor. The other problem is that the Thrust vector is staying horizontal, and thus a sustained steady-state climb is possible.
(Naturally, we can zoom-climb or even loop a glider with no thrust at all. In loop or zoom climb the requirement for a close vector polygon of Lift, Weight, Drag, and Thrust (if present) vanishes, so the constraints are completely different than in a sustained steady-state climb.)
Consider the case of an aircraft like the B-52. The wing is mounted at a high angle-of-incidence to the fuselage to accommodate the "bicycle" landing gear design by allowing a no-rotation takeoff, and to reduce drag in long-range cruising flight. Even with the fuselage level relative to the airflow, the wing is at an efficient angle-of-attack, with a high L/D ratio. If the aircraft is creating more Lift than its Weight, does this mean that it is established in a steady-state climb? No, it means the flight path will curve or bend upwards, causing the aircraft to pitch upwards, which gives the Thrust vector an upward component. At this point Lift will actually decrease slightly to a value that is smaller than Weight as the aircraft settles into a steady-state climb with Thrust greater than Drag, the nose pointing above horizon, and the Thrust vector pointing upwards and helping to support part of the aircraft's weight.
Note that as we change the angle-of-attack of the wing and change the ratio of Lift coefficient to Drag coefficient, for shallow to moderate climb or descent angles, the airspeed eventually responds in such a way that Lift actually stays almost constant, while Drag varies greatly. The reason we choose an optimum angle-of-attack for climbing is really not to maximize Lift, but rather to minimize Drag and thus maximize the ratio of Thrust to Drag. But regardless of whether we've chosen an angle-of-attack that yields a high L/D ratio or a low L/D ratio, if the Thrust vector is pointing horizontally rather than upward, then we aren't climbing - at least not for more than a brief instant. (More on this later!)
Again, for more on the relationship between Thrust, Drag, Lift, and Weight in a climb, see the related ASE answer Does lift equal weight in a climb?
A closing note-- an exotic situation which is not characteristic of normal free flight (meaning that the aircraft is not connected by a towline to another vehicle that is providing the thrusting force) was discussed in this related ASE question and answer. The situation involves a wing sliding up and down on a pole attached to a cart. In this case, even though the thrust vector can be construed to be horizontal, the wing can indeed climb slowly up the pole while maintaining a constant level pitch attitude, but its angle-of-attack relative to the airflow will be decreased as its climb rate increases, causing a self-limiting effect on the climb rate, as discussed in the present answer.
And now a closing note to the closing note-- earlier, we stated "if the Thrust vector is pointing horizontally rather than upward, then we aren't climbing." We've also noted that a glider can be looped with no Thrust at all. A powered plane can also be "zoom climbed" even if Thrust is less than drag, but the airspeed will be decreasing. Note that during the "zoom climb", the thrust line is still usually pointing upward.
Can we come up with a really contrived case where we "zoom climb" without pitching up at all? Yes we can-- but the climb will be very brief. For example, let's say that we're pulling out of a loop. Let's say that we're "pulling" 4G's-- the Lift vector is four times the aircraft's Weight. Just before we reach a horizontal pitch attitude, the airspeed will typically be decreasing, meaning that Drag is greater than Thrust. As we continue to pull up, there will be an instant in time where the pitch attitude is exactly horizontal, but Lift is still much greater than Weight. At that instant, if we relax the back pressure and move the stick forward as needed to exactly freeze the aircraft's pitch attitude, the flight path will still continue to curve upward for a very brief interval of time, until the upward curve of the flight path decreases the angle-of-attack of the wing to the point where Lift vector is equal to the Weight vector, or more precisely, the point where the Lift vector is equal to the component of the Weight vector that acts perpendicular to the flight path. At that instant the centripetal acceleration is zero. The linear acceleration cannot be zero-- as we continue to hold the pitch attitude of the fuselage constant, the airspeed will decrease, and then the flight path will curve downward again until it is exactly horizontal. When the Thrust vector is exactly horizontal, steady-state flight is only possible in the horizontal direction, not in the upward or downward direction. From the pilot's point of view, what has happened is that we've reach a level pitch attitude and then we've rather rapidly "unloaded" the wing to near 1-G condition and transitioned to approximately horizontal flight. The fact that the aircraft did climb very briefly with the fuselage exactly level would probably be impossible to detect without special instrumentation. But yes, technically, it is possible to achieve a very brief interval of climbing flight with the Thrust vector remaining exactly horizontal, and in fact something close to this happens almost every time we transition from a dive to an horizontal pitch attitude, unless we somehow manage to control the throttle in such a way that the airspeed remains exactly constant during the final portion of the pull-out.
It should be clear by now to the reader that this very brief interval of climbing flight with a fixed horizontal pitch attitude, is not the dynamic that we see during any steady-state climb.
The answer is quite simple and has very little to do with lift. The useful power from a thrust $F_t$ on a body with speed $v$ is given by $P=F_tv\cos(\theta)$, where $\theta$ is the angle between the direction of thrust and the direction of movement. This is equal to the difference between the angle of attack and the chord angle.
In other words, for a given thrust from the on the propeller, you get the most energy out of it when the propeller is pointed directly into the relative wind (i.e. for a specific angle of attack). The farther apart these directions are, the more power is being wasted. An extreme example of this is slow flight, where full power may still be inadequate to hold level flight at extreme angles of attack.
In other words, while climbing in a level attitude is possible, it is inefficient in the same way that slow flight is, though to a lesser extent. You get more performance with the same power by allowing the nose to pitch up.
The wings also have a most-efficient angle of attack (best lift-to-drag ratio), which further enforces the idea that certain angles of attack are more efficient than others.
According to Wikipedia and what I recall from my earliest days in training as a private pilot:
Relation between angle of attack and lift[edit] A typical lift coefficient curve. The lift coefficient of a fixed-wing aircraft varies with angle of attack. Increasing angle of attack is associated with increasing lift coefficient up to the maximum lift coefficient, after which lift coefficient decreases.
As the angle of attack increases so does lift. Exceeding the Critical Angle of Attack further illustrates this point.
