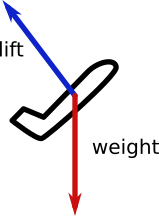
It depends on exactly how you define "lift" and "weight". You might say intuitively that lift is all the forces acting on the aircraft in the upward direction, like this:
In this case, lift must equal weight, otherwise the aircraft would be accelerating. That is, it's rate of climb would be changing.
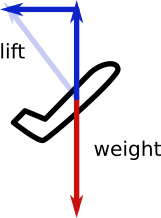
But it's more usual to define lift this way:
Here, lift and weight are equal in magnitude, but in different directions. Of course lift doesn't need to be equal in magnitude: it can be adjusted by the angle of attack. But let's suppose lift is equal to weight and see what happens in this situation where it is.
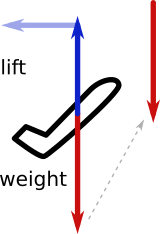
Let's do all our calculations with Earth as the frame of reference1. It's useful to decompose lift into a sum of vertical and horizontal components so we can analyze the horizontal forces and the vertical forces separately:
Comparing the vertical component of lift with weight, we can see they are not equal:
Considering only the vertical forces drawn here, there is a net downward force on the aircraft. So why then is the rate of climb not decreasing?
A similar transformation happens to thrust. In a steady climb, thrust provides an additional upwards component. And of course we must also consider drag. Point being in a steady climb, lift (by the conventional definition) is not equal to weight, but the sum of all the vertical components of lift, thrust, and drag do equal weight.
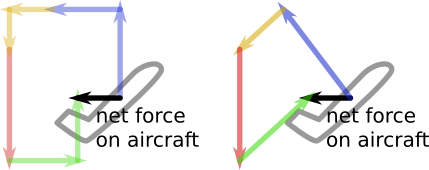
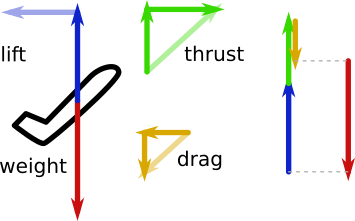 Let's add an arbitrary amount of drag, and enough thrust to balance the vertical forces.
Let's add an arbitrary amount of drag, and enough thrust to balance the vertical forces.
Note there's a similar balance required in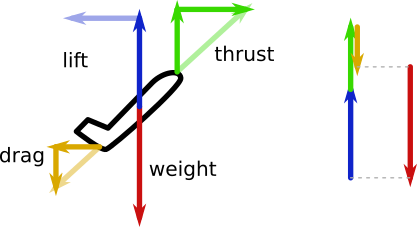
Now the vertical forces are balanced, but the horizontal directionforces must also be balanced if we want stable flight. If you were to add upAdding all the horizontal forces in my drawing, you'll see there's a net force to the left, meaning while the. So this aircraft may be maintaining a steady rate of climb is constantat this instant, the groundbut it's losing speed is decreasing, and likely the airspeed with itprobably headed for a stall.
Remember, we initially set lift equal in magnitude to weight, and this is what happens. Without changing the direction or magnitude of lift, there's no solution that results in stable flight.
Therefore, a climbing aircraft requires less lift. To maintain this rate of climbdirection and velocity, this pilot must: reduce lift by reducing the angle of attack, and increase thrust such that the vectors add to zero and there's no net force on the aircraft. Reducing lift will also reduce drag.
- continually increase thrust, until the aircraft is flying straight up purely on thrust with no lift (perhaps I should have drawn a military aircraft), or
- continually increase angle of attack to offset the loss of lift due to decreasing airspeed, ultimately resulting in a stall, or
- decrease angle of attack (thus decreasing lift and drag) and increase thrust to bring all the forces into balance.
A shortcoming of using a graphics editor as a flight computer, I suppose :)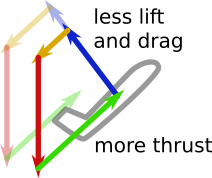
1 Any other frame of reference could work. For example we could use the aircraft as the frame of reference, which would mean lift is always up, but weight would change direction.