How does the aspect ratio of a wing impact its lift?

Question

The wing lift formula shows that lift of a wing is proportional to its area.

$ L = {\dfrac 1 2 \times \rho V^2 \color{magenta}S C_L} {\small \begin{align} &{} &&\text{where:} &&L = \text{lift,} &&\rho = \text{density of air,} \\ &{} &&{} &&V = \text{velocity,} &&\color{magenta}S = \text{wing area,}\\ &{} &&{} &&{}&&C_L = \text{coefficient of lift.} \end{align}} $

So why are most conventional wings shaped the same (swept back rectangles)?

Imagine a conventional airplane but with the wings shaped as 2 thin long rectangles attached to a fuselage from the cockpit until the tail with the same area as the original wing.

With all other things (like angle of attack, etc) being equal will the same lift be generated as the original wing?

Answer 1

"The wing lift formula shows that lift of a wing is proportional to its area".

That's absolutely true. However a wing generates both lift and drag. Drag is of two natures: Parasitic drag and lift-induced drag. The sum of all drag is the total drag:

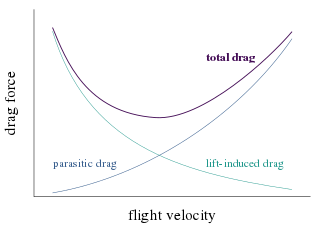
^{Source: Wikipedia}

Induced drag decreases with speed, and has its origin in wing tip vortices. Vortices actually increase the velocity of the downwash, and changes the effective angle of attack. Which in turn changes the direction of the aerodynamic force, creating a force opposing to the direction of flight:

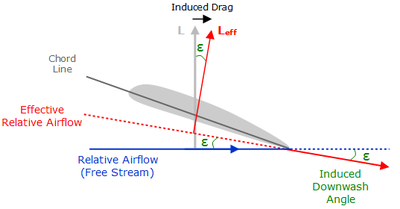
^{Induced drag, source: Wikipedia}

Induced drag being due to tip vortices, if we can make vortices less efficient, then we also reduce induced drag. The way to do that is to have long wings, so the the downwash from the vortices affects only a part of the wing:

^{Tip vortices, source: Wikipedia}

Research demonstrated that the induced drag is dependent on the wing aspect ratio, not only on the wing span. This can be understood easily: The quantity of air in a tip vortex is larger when the chord is large.

So the answer to your question is: Yes lift is proportional to the wing area, but the lift/drag ratio is proportional to the wing aspect ratio. That's why longer wings are preferred, they minimize energy lost in fighting drag.

---

"Why are most conventional wings shaped the same (swept back rectangles)?"

Longer wings are better for fuel efficiency, but we have other problems in the designer queue, and some can be solved by selecting the wing planform, e.g.:

• We want to prevent the stall to be abrupt (at the expense of creating less lift though):
  
  

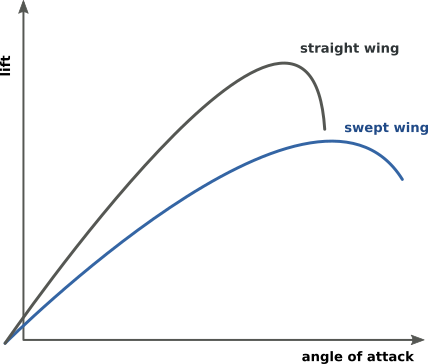
• We want to delay the creation of a local supersonic flow in supercritical wings used in commercial airliners. When flying at M 0.8, airflow is accelerated to produce lift, some areas of the wing reach supersonic speed (Mach > 1). The associated shock wave creates an additional drag. By sweeping the wing we add a spanwise component to the airflow which decreases the chordwise velocity, so the shock wave appears only to a higher aircraft airspeed:
  
  

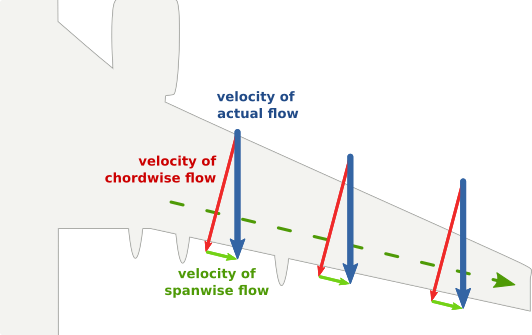
On the other hand, the sweptback wing tends to stall at the tip first, which is not good at all, as when a stall appears we need ailerons to prevent it from worsening, and ailerons are located at the tips to increase their effectiveness. So swept wings are also twisted so that the tip angle of attack is smaller than the root one, forcing the stall to start at wing root.

---

Wings planforms are multiple, each type of wing, or sometimes subcomponent, can improve a particular issue (possibly creating another that will be less important for the designer). See this very interesting article on Wikipedia: Wing configuration

Answer 2

A higher aspect ratio (given the same wing area) means more wing span and less lift-dependent drag. At the same angle of attack, higher aspect ratio also means more lift (within limits).

Lift is produced by deflecting the oncoming stream of air downwards. The more air can be affected, the more efficient lift production becomes. Maybe you want to read a few answers around here if you want to learn more.

What you describe is a slender body wing. Most of the deflecting is done by the first few percent of this wing's chord, and the remainder will not be able to increase lift more, working on the already deflected air. Consequently, the same area of wing will create less lift force, at least in subsonic flow. Take the XB-70, for example: Its maximum wing loading (mass divided by area) was 420 kg/m². The swing-wing B-1, which could stretch its wings out for slow speed flight, has a maximum wing loading of 1190 kg/m², however, the calculation method of its wing area does not include the large delta section ahead of the main wing. Still, a sizeable difference remains and shows that the same wing area can create more lift when stretched out.

Answer 3

The "wing lift formula" that you quoted is a simple approximation and is not used for the detailed design of wings.

Practical reasons why conventional wings have the shape they do depend on many design considerations, including the mission of the aircraft. The answers you have received address some of those issues.

Be aware though, that there are many misconceptions in wing design. One common misconception is that: "Induced drag being due to tip vortices, if we can make vortices less efficient, then we also reduce induced drag."

That is a great over-simplification. Induced drag depends on the shape of the entire wing, not just the aspect ratio and the tip vortices.

Former Boeing Technical Fellow Doug Maclean discusses this misconception in particular, and many others in his book: Understanding Aerodynamics - Arguing from the Real Physics.

You might get a lot out Maclean's talk:

Common Misconceptions in Aerodynamics