During design, chord length will be the result of wing span and wing area, because those two are what the designer wants to set. To be more precise, he tries to set a certain wing loading, trying to minimize wing area (and weight) such that the wing creates just enough lift for the estimated aircraft mass in all design cases. Take-off, maximum altitude and minimum turn radius are the classics which drive minimum wing area.
Now I will focus on aspect ratio, because Jan has already answered the rest with his excellent answer. The higher the aspect ratio, the less the wing tips will influence the airflow around the wing. This means that the wing creates more lift for a given angle of attack, but also that the stall angle of attack is lower. The ratio of wing span to aircraft mass (called span loading) is the driving force for induced drag. However, wing span and aspect ratio will also drive wing structural mass, so you need to find a sound compromise between low induced drag and low wing mass.
What is induced drag? It is the consequence of creating lift over a limited span. The wing creates lift by deflecting air downwards. This happens gradually over the wing's chord, and creates a reaction force orthogonally to the local speed of air. This means the reaction force is pointing up- and slightly backwards. This backwards component is induced drag! The wider the wing is, the more air can be used for lift creation, hence less deflection is needed. Consequently, the backwards tilt of the reaction force is smaller, resulting in less induced drag for the same lift.
If you fly fast, there is a lot of air mass streaming past the wing per unit of time, so you need to deflect the air only slightly. Your induced drag is small. That is why induced drag changes inversely with air speed.
Now you know that for high speed at high density, induced drag is not important. If you design an attack aircraft which has to fly at low altitude, a low aspect ratio will help: The lift increase due to gusts is smaller than with a high aspect ratio wing, and the induced drag is manageable.
How the lift curve slope changes with aspect ratio in subsonic flow is shown in the simple plot below. For a slender body (aspect ratio $\approx$ 0), the gradient of the lift coefficient $c_L$ over angle of attack $\alpha$ is $c_{L\alpha} = \frac{\pi \cdot AR}{2}$. Please note that the red line is only valid for AR = 0! Then the lift curve slope increases up to $c_{L\alpha} = 2\cdot\pi$ for $AR = \infty$ (and zero airfoil thickness and no friction effect), as shown by the blue line.
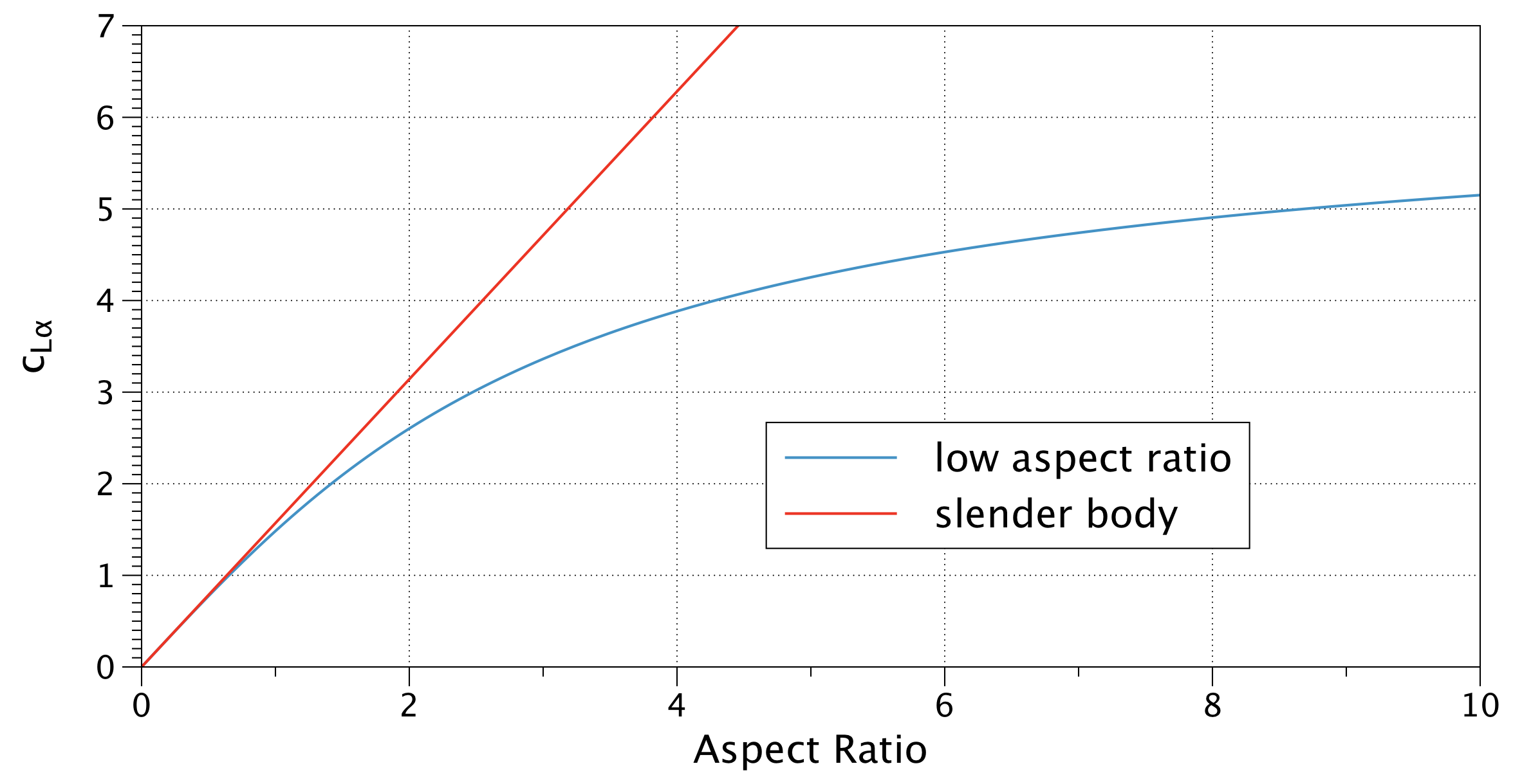
There are more influences, however. Dihedral means that the lift is tilted inwards, and the part which counteracts weight is only growing with the cosine of the dihedral angle $\nu$. Same goes for sweep: Sweeping means that the wing only sees a reduced angle of attack change. If you want to capture all effects, a plot will not suffice. Here is a table with formulas for most cases:
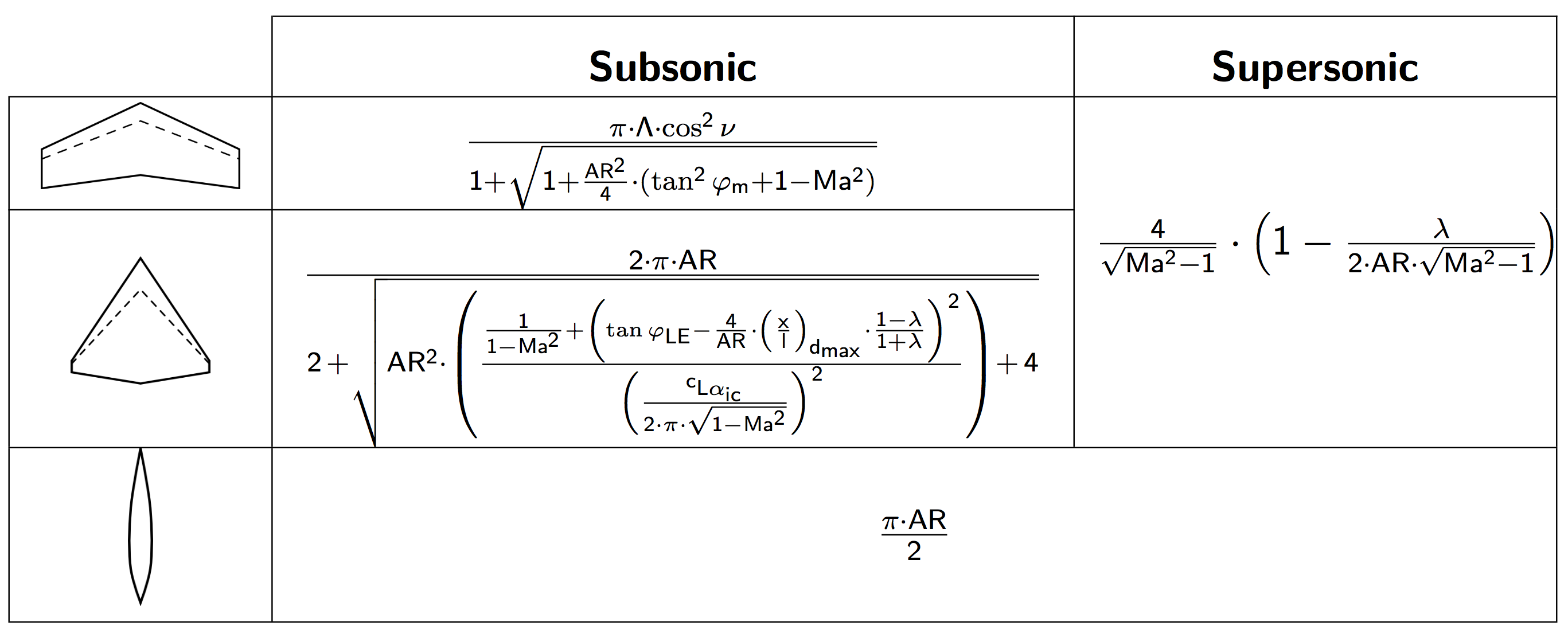
Nomenclature:
$c_{L\alpha} \:\:$ lift coefficient gradient over angle of attack
$c_{L\alpha\:ic} \:$ lift coefficient gradient over angle of attack in incompressible flow
$\pi \:\:\:\:\:$ 3.14159$\dots$
$AR \:\:$ aspect ratio of the wing
$\nu \:\:\:\:\:$ the wing's dihedral angle
$\varphi_m \:\:$ sweep angle of wing at mid chord
$\varphi_{LE} \:$ sweep angle of wing at leading edge
$\lambda \:\:\:\:\:$ taper ratio (ratio of tip chord to root chord)
$(\frac{x}{l})_{d\:max} \:$ chordwise position of maximum airfoil thickness
$Ma \:\:$ Mach number
Another consideration is wing volume: In most aircraft, the wing will hold most of the fuel, and a long-range aircraft needs big tanks. Sometimes, only choosing a lower aspect ratio will give enough wing volume for the required range. In order to keep induced drag constant, the span will be kept the same, so wing area increases with chord length. This has the added benefit of more lift, so less complex high-lift devices are needed. Since the structural mass of such a low aspect ratio wing with simple flaps is relatively low, the only disadvantage is the higher friction drag of this larger wing.
If maneuverability is important, wing span needs to be as small as possible. This reduces inertial moments and roll damping, so the aircraft can accelerate faster into a rolling motion and will reach a higher roll rate. This is extremely important in dogfights when the one who points his radar, gun and rockets at the adversary first will win. Here the chord is chosen for sufficient wing area at the minimum practical wing span.
Now we need to talk about viscous effects. Friction between air molecules and between air and wing. The ratio of inertial to viscous forces is expressed by the Reynolds number, and generally a higher Reynolds number means your friction effects go down, translating into less friction drag and a higher stall angle of attack. Especially for model airplanes, but also for gliders, it is sometimes better to reduce aspect ratio in order to gain absolute wing chord. The Reynolds number increases linearly with wing chord, and this can also be a consideration for choosing wing chord.
A normal wing has positive camber, and this means that the center of pressure moves forward with increasing angle of attack. Moment-wise, this means that the wing will create a stronger pitch-up moment when it pitches up. This makes the wing by itself unstable, and you need a tail surface to regain stability. Making wing chord longer will increase the unstable influence of the wing relative to the unchanged tail surface. This is why I said that increasing chord will decrease stability. During design, you watch for a size called tail volume. This is the area of the horizontal tail surface, multiplied with it's lever arm expressed as a multiple of the wing's reference chord. If you keep this constant while changing wing chord, your stability stays the same. But then you change your pitch damping, because this is affected by the square of the lever arm. Digging deeper will uncover more consequences, so I better stop here for today.


