Propeller efficiency is mentioned a lot here on Aviation SE, but lacks a good explanation. Here we go:
A propeller accelerates the air of density $\rho$ which is flowing through the propeller disc of diameter $d_P$. This can be idealized as a stream tube going through the propeller disc. This stream tube starts with air at ambient speed and a wide diameter d$_0$. As the suction ahead of the propeller accelerates the air in the streamtube, it starts to speed up and the stream tube contracts. In the propeller plane we witness a jump in pressure p: Here, energy is added to the flow, so Bernoulli does not apply momentarily. Past the propeller the air in the stream tube accelerates further and the tube contracts to the diameter d$_1$ when the pressure inside has dropped to the ambient pressure (dashed line in the pressure graph below):

The air speed ahead is $v_0 = v_{\infty}$ and the air speed aft of the propeller is $v_1 = v_0 + \Delta v$. The propeller effects a pressure change which sucks in the air ahead of it and pushes it out. Since the mass flow must be equal ahead and behind the propeller, the stream tube diameter is bigger ahead of the propeller and smaller downstream. In reality, there is no neat boundary between the air flowing through the propeller and that surrounding it, but for computing thrust this simplification works well if the airspeed is identical across the cross section of the propeller disc.
The efficiency $\eta$ of thrust creation is the work done on the mass flow through the propeller $W = m\cdot\Delta v\cdot v_0$ relative to the impulse change of the air $\Delta I = m\cdot\frac{v_1^2 - v_2^2}{2}$:
$$\eta^{opt} = \frac{2\cdot v_0}{v_1 + v_0} = \frac{v_{\infty}}{v_{\infty} + \frac{\Delta v}{2}} = \frac{1}{1 + \frac{\Delta v}{2\cdot v_{\infty}}}$$
This equation assumes that air is uniformly accelerated straight backwards. To be more precise, you need to add the swirl losses, since the air receives a rotational component $\omega$ from the propeller, spinning with the angular velocity $\Omega$, as well:
$$\eta^{opt}_{Prop} = \frac{1 - \frac{\Delta v\cdot \left({v_{\infty} + \frac{\Delta v}{2}}\right)}{d_P^2\cdot\Omega^2}}{1 + \frac{\Delta v}{2\cdot v_{\infty}}}$$
Still, we have not included friction losses yet and our prop spinner and engine nacelle are also not included. Now we must enter into a definition what propeller thrust is: Is it just the lift acting on the propeller blades in forward direction, or is it the remaining forward force after the additional drag of the airplane components in the slipstream of the propeller has been subtracted?
To avoid lengthy computations, charts can be used where the efficiency is plotted over a range of parameters.
To cut the discussion short: Generally it is safe to assume a top propeller efficiency of 0.85 (85%) with big, slowly spinning propellers (1000 to 1700 RPM). If the twist distribution along the blade does not match the local angle of attack distribution (say, if the propeller is optimized for high speed, but operated at slow airspeed, like during take-off), efficiency can easily drop to 0.7 (70%). Things become worse if the pitch of the blades is fixed. See below for a typical example of a variable pitch propeller. Each of the curves is for a different pitch setting, the x axis shows the advance ratio (the ratio between airspeed and circumferential speed; here off by a factor of 1/$\pi$), while the y axis shows the efficiency.
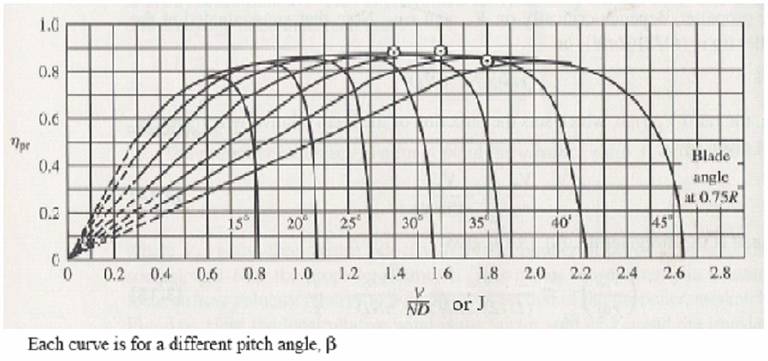
Efficiency chart of a variable pitch propeller. Source: McCormick B.W. Aerodynamics, Aeronautics & Flight Mechanics. John Wiley & Sons, Inc., 1979.
What can be seen from the equations above is that it is more efficient to accelerate lots of air a little than to accelerate a little air by a lot. This means that small propellers on ungeared engines, spinning at high RPM, are at a distinct disadvantage; this is why Roskam assumes only 70% for them and only 80% for GA propellers.
The 90% you name has to my knowledge only been scratched at by some very efficient (slow, large, contra-rotating) propellers operating under ideal conditions. To play it safe, I would choose a slightly smaller number for them.


