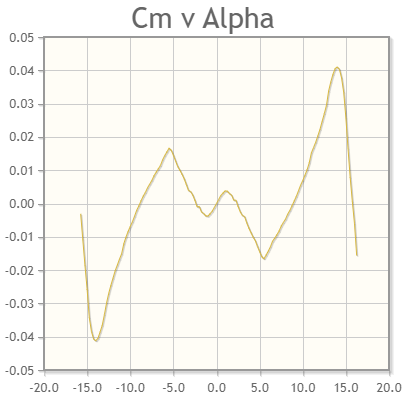
As I understand it, the pitching moment coefficient of a symmetric aerofoil should be zero, under potential flow assumptions. Obviously, approaching stall and post-stall, these assumptions are invalid and the pitching moment coefficient would no longer be zero.
However, looking at this graph of pitching moment coefficient against angle of attack, for a NACA0009 aerofoil at Re = 1e+6, it seems like Cm does actually vary considerably at low and moderate angle of attack.
Why?
Under incompressible potential flow and at small angles of attack, one of the most celebrated results of Thin Airfoil Theory is that all airfoils have their aerodynamic centre at 1/4 chord; and for symmetrical airfoils, the centre of pressure coincides exactly with aerodynamic centre.
If you look at the plot in the OP, the variation of Cm between -3 and 3 deg are relatively small, indicating that the linearized theory agrees well with the nonlinearized simulation.
Beyond 3 deg, however, the viscous effects present in the simulation but absent from Thin Airfoil Theory begin to alter the results. This manifests primarily in the boundary layer, which alters the apparent shape of the airfoil, thereby making it non-symmetrical. The next graph shows the XFOIL simulation at 7deg AOA; notice that the boundary layer at upper surface thickens near the trailing edge.
