How to distinguish the induced drag of a lift-producing wing from its pressure drag?

Question

Quote John Anderson Fundamentals of Aerodynamics:

"The three-dimensional flow simply alters the pressure distribution on the finite wingin such a fashion that a net pressure imbalance exists in the direction of V∞ (i.e., drag is created). In this sense, induced drag is a type of “pressure drag."

Wing at 15°AoA flying in the sky and producing lift same as wing weight...

AXIOM: Fluid can exert forces at object only in two ways: pressure (normal force) and viscosity(tangential force)

Integration of all tangentional forces at wing ,gives resultant force.Component in backward direction-parallel with freestrem is SKIN FRICTION DRAG

Integration of static pressure over entire wing,gives resultant pressure force.Component of that force in backward direction-parallel with freestrem is PRESSURE DRAG.

What is than INDUCED DRAG, how distinguish induced drag(which is pressure drag) from pressure drag when wing produce lift?

Answer 1

There are multiple ways to decompose lift and drag forces, and they are unfortunately not compatible with each other.

If you know the flow field (for example because you ran a CFD simulation), then to compute lift and drag, you need to integrate:

• pressure forces (i.e. local pressure times surface normal, over area)
• viscous forces (local viscous stress times shear direction, over area) shear stress is tangential to the local surface, but because not all surfaces are tangential to the flight direction, this influences both lift and drag (though usually a lot more to drag).

That gives you two force vectors, and after you added them up, you can then decompose them into one component which is parallel to the inflow direction (drag), and one which is normal to it (lift). (let's forget spanwise forces for now...). Looking at drag, you can then of course see which part comes from the pressure forces and which comes from the viscous forces.

Next, induced drag: This is actually a somewhat theoretical definition, and most people today speak about "lift-dependent" drag. This means: How much more drag is the airfoil producing because it produces lift? Assuming an uncambered airfoil, the lowest drag is at AoA=0°, when lift is also zero, so all additional drag we're getting at AoA=15° is lift-dependent. Assuming a cambered airfoil, the lowest drag is actually not at zero lift, and also not at AoA=0°, so at lowest drag, it's actually producing some lift -- so ... negative lift-dependent drag! Just look at these drag polars: 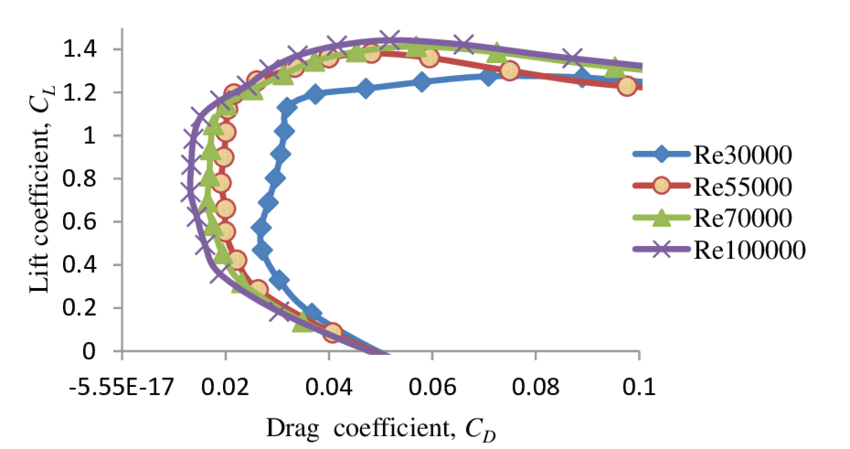Does that mean we have negative induced drag? Our definition is already becoming difficult to use. So let's keep the airfoil uncambered for now, meaning that the lowest drag is also at AoA=0, where we produce no lift.

So, under these circumstances, what happens to drag when we increase AoA? Of course, pressure on the upper side of the wing reduces, and it increases on the lower side. This means we're getting a pressure force which is pointing mostly upwards (lift) but also somewhat backwards (drag). But we're accelerating the flow on the upper side, which increases friction there. We're decelerating on the lower side, but that effect is a bit smaller. This means we're getting some additional friction drag. But that's not all! Because of the additional friction on the upper side, the boundary layer grows faster than it would otherwise, changing the streamlines, which in turn changes the pressure distribution, and causes additional pressure drag. This means: If we switched friction off now, we'd actually reduce pressure drag, too!

So, really, we can't point at the change in pressure drag and call it induced drag.

Now, if we make some more simplifying assumptions -- the kind that people used to make all the time when they were still using pencil and paper to design airplanes -- that's when things finally start to add up. This means we're assuming simple potential flow, and maybe we're adding some estimate of viscous drag based on flight speed and surface area, which is not affected by pressure distribution. In that case, we would have no pressure drag on our symmetric airfoil at AoA=0°, and all the pressure drag we're getting at AoA=15° is purely because the pressure on the airoil is pushing normal to the surface, the upper side is also facing backwards to some extent, because it's at incidence to the flow. Now, all the pressure drag is indeed due to lift, and viscous drag is not affected by lift.

So, until now I was talking about "lift-dependent" drag. But what about "induced" drag? Even the Wikipedia article on induced drag doesn't make a difference between lift-dependent and induced drag, so how big can it be? Fairly large, actually. The most common definition for induced drag is the drag generated because the wing produces trailing vortices. So all the kinetic energy in the wing-tip vortices (but also in the vortex sheet behind the wing wherever the lift is changing in spanwise direction) needs to come from somewhere, and that's called induced drag. At least in simplified physics, that is indeed completely pressure drag -- but it does not necessarily explain all pressure drag. Imagine for example an infinite wing. No change in lift distribution, no trailing vortices, but it must have some pressure drag! Mathematically, this can be solved by assuming that when the wing accelerated or increased AoA, it generated a parallel vortex which it left behind, and keeps feeding via two imaginary wing-tip vortices at infinity. But if you measure a 2D profile in a wind tunnel or simulate one with modern CFD methods, the lift-dependent part of drag is much larger than that, because the theoretical induced drag is pretty small next to all the real effects which happen on top of it.

Now, if you take one more step towards reality, and include cambered airfoils, viscosity, boundary layer displacement, and if you're going fast enough also compression shocks (which produce "wave drag", which another factor influencing viscous and pressure drag...) -- that's when "induced drag" becomes fairly theoretical.

So why on earth does anyone still use it? Precisely because it is simple to compute in simple physics models, where you ignore a lot of real effects. That's when it still does tell you what the lowest achievable lift-dependent drag for your wing shape would be, if all those nasty interactions between pressure field and boundary layer, separations, shock waves and other complications did not exist. This means: Induced drag is a useful construct to explain why lift always produces drag, why long slender wings can be more efficient at producing lift, and how much more efficient. But in a real flow, there's not really a way to extract it separately.

Footnote: Of course there are methods to at least approximately extract the different drag components. The best-known tool to do this is Onera's FFD tool (which only few people outside Onera get to use...). I did not find the original paper quickly, but here is the extension to unsteady flow. You can see the math becomes pretty complicated very quickly. You can also see that they provide a lot of drag components, but a closer look shows that although they include induced drag, and a lot of other components, they're not all adding up to total drag -- that's because there are lots of ways to decompose drag, and most of them don't neatly align.

Answer 2

This is good one. Doug Mclean:

Let’s first put induced drag in perspective by looking at drag in general. Drag is just the flight-direction component of the total aerodynamic force, excluding engine thrust. (For purposes of this discussion, we’ll assume drag and thrust can be cleanly separated, ignoring some serious theoretical difficulties.) The air acting on each local element of the airplane’s external surface makes a contribution to the force that can be resolved into a component parallel to the local surface (shear force) and a component perpendicular to the surface (pressure force). When these two components are resolved in the flight direction and integrated over the entire external surface, the resulting forces are generally referred to as the "skin-friction" drag and the pressure drag. The skin-friction drag is entirely a result of viscous effects (viscosity and turbulence) in the boundary layers on the airplane’s surfaces. The pressure drag is a result of a more complicated combination of flow mechanisms, including viscous effects, shocks, and the global effects of lift. Given enough data defining the distribution of forces on the surface, resolving the drag into a skinfriction part and a pressure part is straightforward, since it involves simply resolving a vector into components. Dividing the drag into viscous drag, shock drag, and induced drag according to the mechanisms responsible isn’t so simple.

We’d like to define induced drag as the part of the drag due to the global effects of lift. We’ve already seen that the global effects of lift contribute to the pressure drag, but that the total pressure drag also contains contributions from other flow mechanisms. How do we define how much of the pressure drag is induced drag? There is nothing about the distribution of the forces exerted on the surface that will tell us how much of the drag was caused by which flow mechanism. And it turns out that looking at the flowfield doesn’t yield a rigorous definition either. Because the different flow mechanisms overlap and interact, their effects do not add in a simple linear way to the total pressure drag, and an exact decomposition of the pressure drag into component parts IS NOT POSSIBLE. However, for practical purposes, it is possible to make an approximate decomposition, based on idealized, approximate theories regarding what goes on in the flowfield. For example, if the flow in the neighborhood of a shock is known, the shock’s contribution to the drag can be estimated based on the Oswatitsch formula. Likewise, if the spanwise distribution of lift is known on the lifting surfaces, the induced drag can be estimated using Trefftz-plane theory, which is based on an idealized model of the flowfield associated with the given spanloading. So we must keep in mind that the idea that drag can be decomposed into different “components,” according to the flow mechanisms responsible, is an idealization. It is a useful one, however, and in practice, predictions of drag increments based on these idealized models have proved to be reasonably accurate.

Now let’s look at how the induced drag is distinguished from the other pressuredrag components, physically speaking. All forms of drag manifest themselves in the flowfield in two main ways. First, conservation of momentum requires that the drag force alters the balance of momentum and pressure. Second, conservation of energy requires that the work done against the drag force shows up as an increase in the combined heat energy and kinetic energy. (Note that while both of these relationships can be correctly expressed in any reference frame, the work/energy relationship is most clearly understood in a reference frame fixed to the air mass rather than the airplane, since that is the frame in which the work done relates most directly to the energy expended by the propulsion system.) With viscous drag and shock drag, the dissipation of energy into heat is immediate, and very little kinetic energy is involved. Induced drag is unique in that nearly all of the energy added to the flow shows up initially as kinetic energy and is dissipated into heat only very gradually over a long distance downstream.

The kinetic energy produced by induced drag is associated with a large-scale air motion caused by lift forces, mostly on the wing. In general terms, the motion is mostly perpendicular to the direction of flight and is characterized by downward flow in the area between the wingtips and upward flow outboard of the tips, as shown in Figure 3.1. Note that these lift-induced velocities are not concentrated closely just around the wing itself or the wingtips, but are spread fairly diffusely over a wide area of the flowfield.

While the air more than about one wingspan ahead of the wing is essentially undisturbed, the general flow pattern of Figure 3.1 reaches practically full strength at a distance of about one wingspan behind the wing and generally persists over long distances downstream. At the location of the wing itself, the flow pattern has reached roughly half of its maximum strength, and the wing is flying through air that is already moving generally downward between the wingtips. Thus the wing can be thought of as flying in a downdraft of its own making. Because of the apparent downdraft, or “downwash,” the total apparent lift vector is tilted backward slightly. It is the backward component of the apparent lift that is felt as induced drag. When we look at the force/momentum balance, the induced drag shows up in the flowfield primarily as reduced pressure downstream of the wing.

Figure 3.1