11
$\begingroup$

I know that the Wright brothers started to use a catapult in September 1904 to help their Flyer II take off easily even when no headwind was available.

Assuming the propellers did not turn, or they were not even mounted on their shafts, how far away the catapult could have thrown Flyer II together with its engine and pilot? 1m, 5m, 10m, 25m, 100m or more? Even a rough evaluation, based for example on an experiment with a scale model, would be good for me.

(Note: I am not interested in a pure ballistic evaluation, considering the plane the equivalent of canon ball of mass, m, that is accelerated to the speed, v, and launched at an angle alpha.)

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ I'm not sure that this experiment has been done with a scale model, are you actually asking that somebody build an accurate scale model and attempt to replicate this for your answer? Otherwise a purely ballistic answer or mathematical one is all you can hope for... $\endgroup$
    – Ron Beyer
    Commented Mar 13, 2017 at 2:41
  • 1
    $\begingroup$ The catapult used 1400lbs of weights falling from a 20-foot tower for propulsion. Maybe somebody can do the math and figure out how much energy that imparts vs the performance of the Flyer $\endgroup$
    – TomMcW
    Commented Mar 13, 2017 at 3:04
  • 6
    $\begingroup$ It appears that a Flyer II, with the engine stopped and accelerated only by that falling weight, could not have flown at all because the energy communicated by the weight to the plane is about the same as that required by the 925 lbs Flyer II to reach the take off speed (30 mph). --- E_weight=(1400 pound) * (20 ft) * (9.81 (m / (s^2))) = 37 975.87 joules --- E_plane_take_off=(925 pound * ((30 mph)^2)) / 2 = 37 732.25 joules. The moment the take off speed is attained, the weight stops, the aerodynamic friction diminishes the speed and the plane remains below the required 30 mph flight speed. $\endgroup$
    – Robert Werner
    Commented Mar 13, 2017 at 5:45
  • $\begingroup$ "thrown" is not the right word - it merely pulled the plane down a track for a distance. If the plane didn't get enough airspeed from the pull and the headwind, it wouldn't take off when the elevator was manipulated. $\endgroup$
    – CrossRoads
    Commented Jun 6, 2018 at 19:15

2 Answers 2

Reset to default
11
$\begingroup$

The catapult cannot launch an unpowered Flyer II at all.

Robert's comment reaches the right conclusion using potential to kinetic energy, though his frictionless answer up to takeoff overestimates available energy and depends on aero drag not to take flight. The corrections are:

  • All the potential energy from the weight is not transferred to the flyer. The weight builds kinetic energy that is dissipated as it slams into the ground. Its velocity is one third that of the flyer.
  • Though the tower is 20ft tall, the weight only falls 16ft(5m). The rope and weight take up space.

Setting potential energy of the weight equal to kinetic energy of weight and plane:

$$ M_w\cdot H_w\cdot g=\frac{1}{2}M_f\cdot V_f^2+\frac{1}{2}M_w\cdot V_w^2$$ $$635kg\cdot 5m\cdot 9.8\frac{m}{s^2}=\frac{1}{2}\cdot 408kg\cdot V_f^2+\frac{1}{2}\cdot 635kg\left(\frac{V_f}{3}\right)^2$$ $$130\frac{m^2}{s^2}=V_f^2$$ $$V_f=11.4\frac{m}{s}=25mph$$ Since the Flyer II needed 28-30mph to become airborne, the catapult cannot launch the unpowered flyer by itself, even excluding friction and drag.

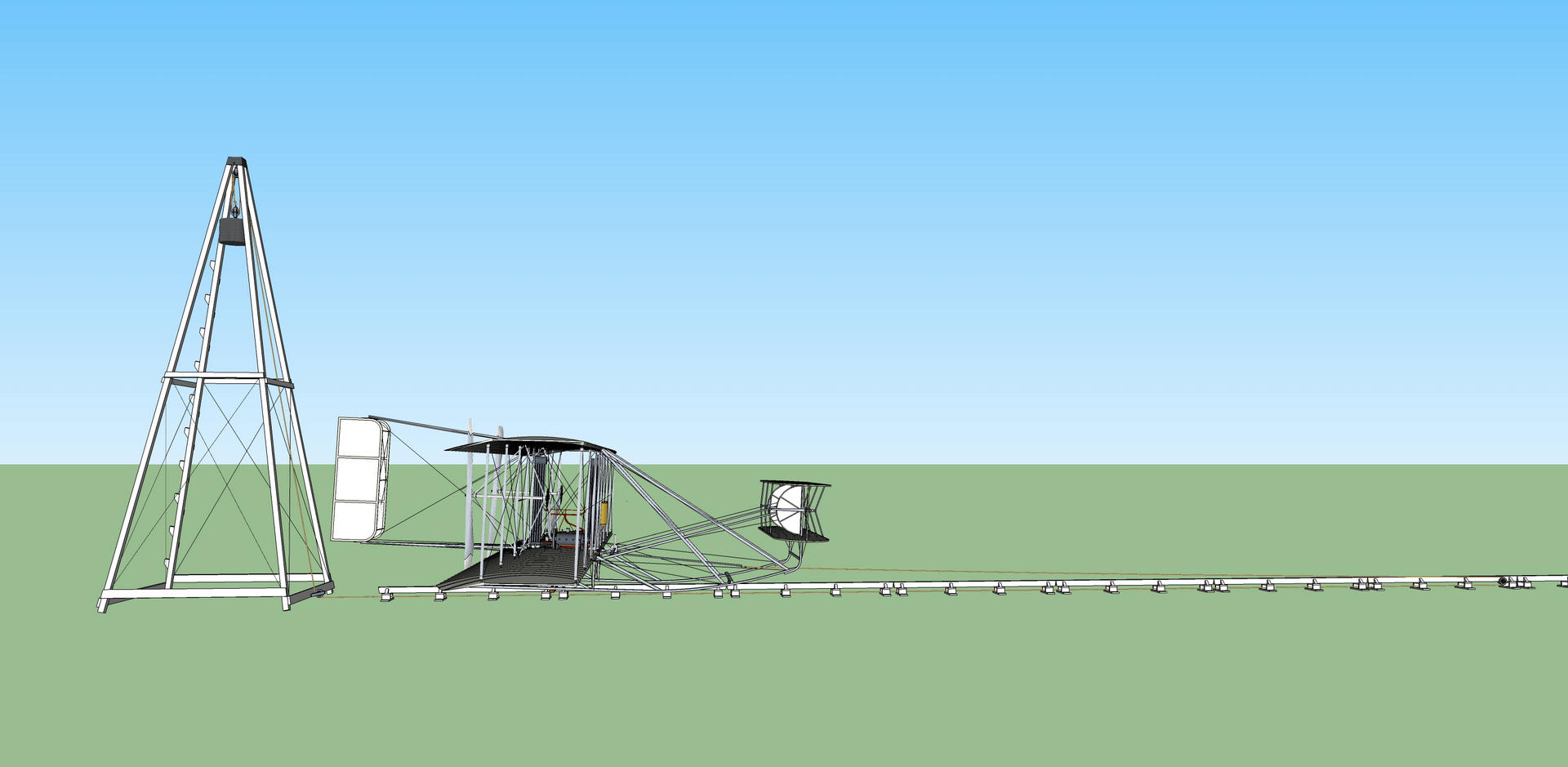
enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ The fact that the Flyer required 28-30 mph to become airborne just means that it required this speed for the lift to balance its weight. A Flyer traveling at 25 mph would still presumably have a substantial amount of lift (just less than its weight), and would fly further—perhaps much further—than a simple ballistic trajectory. $\endgroup$
    – Michael Seifert
    Commented Nov 6, 2019 at 0:43
  • $\begingroup$ @MichaelSeifert, the rail was neither sloping up nor elevated, so the Flyer traveling at 25 mph was still on the ground (ok, maybe a foot above it as the rail has some thickness), so it couldn't fly anywhere without climbing, and it couldn't climb at that speed. $\endgroup$
    – Jan Hudec
    Commented Nov 6, 2019 at 6:50
0
$\begingroup$

The Wright catapult was powered by a 635 kg weight, dropped 5 meters.

It's potential energy, before dropping, mgh = 635 kg × 9.8 m/s^2 × 5 meters = 31115 Joules.

The 408 kg Flyer II was connected to the drop weight with a compound pulley that moved it 15 meters when the weight dropped 5 meters.

Dropping the weight converted potential energy to kinetic energy as follows:

mgh × 2 = drop weight mass (v)^2 + launch mass (3v)^2

drop weight speed = v launch speed = 3v

The minimum flying speed was around 30 mph or 14.2 m/s

The catapult was mathematically evaluated with various launch masses, without friction or drag factors, using no gearing and 3x gearing for comparison: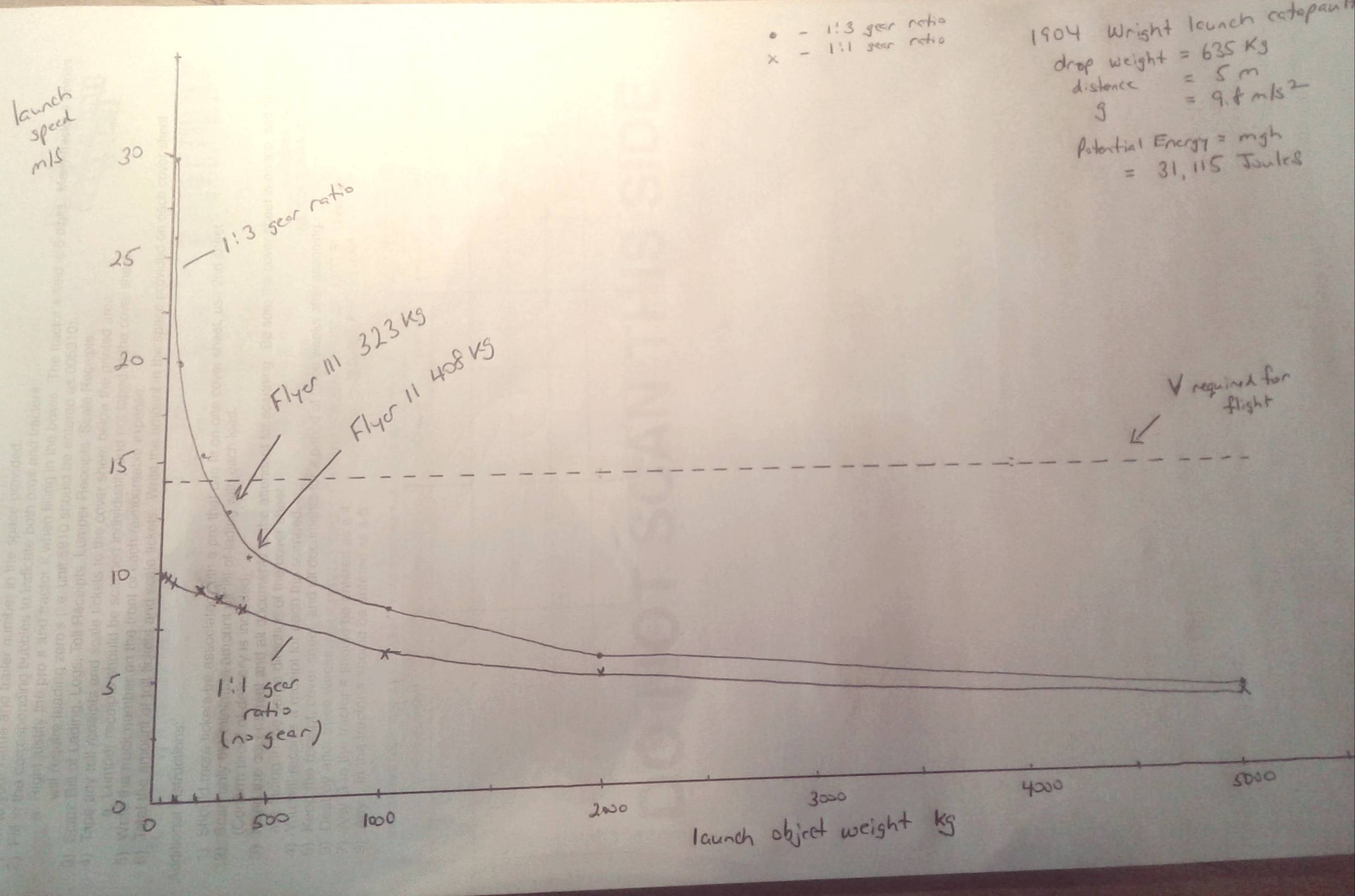enter image description here

Although the 1:3 gearing was a significant improvement in the catapult design, the 408 kg Flyer II was too heavy to reach launch speed, with a theoretical 11.4 m/s.

The much improved Flyer III, coming in at a svelte 323 kg, would come closer at 12.6 m/s.

Still too slow, with obvious solutions utilizing increased drop height, more drop weight, and still lighter aircraft design, why would the the Wrights hold below launch speed?

The answer may be in the other aspect of their development, the internal combustion engine. Holding catapult launch speed down may have given them a crucial abort option, with the plane dropping to the ground a few feet from the rails with little or no damage.

With a more reliable engine, a more powerful catapult may have been more desirable. With sufficient runway length, it is not needed.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .