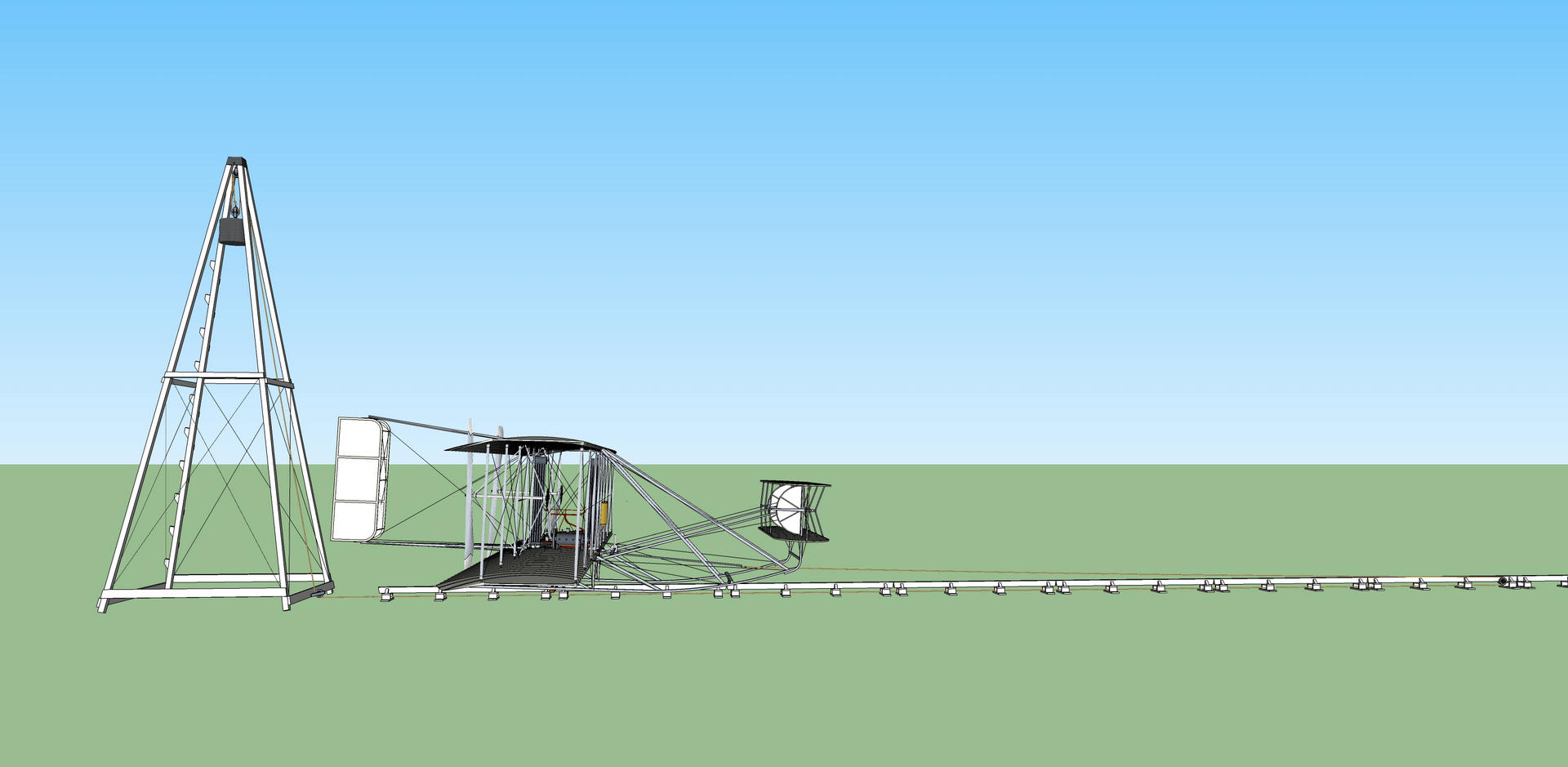
The catapult cannot launch an unpowered Flyer II at all.
Robert's comment reaches the right conclusion using potential to kinetic energy, though his frictionless answer up to takeoff overestimates available energy and depends on aero drag not to take flight. The corrections are:
- All the potential energy from the weight is not transferred to the flyer. The weight builds kinetic energy that is dissipated as it slams into the ground. Its velocity is one third that of the flyer.
- Though the tower is 20ft tall, the weight only falls 16ft(5m). The rope and weight take up space.
Setting potential energy of the weight equal to kinetic energy of weight and plane:
$$ Mw*Hw*g=1/2Mf*Vf^2+1/2Mw*Vw^2$$$$ M_w\cdot H_w\cdot g=\frac{1}{2}M_f\cdot V_f^2+\frac{1}{2}M_w\cdot V_w^2$$ $$635kg*5m*9.8m/sec^2=1/2*408kg*Vf^2+1/2*635kg(Vf/3)^2$$$$635kg\cdot 5m\cdot 9.8\frac{m}{s^2}=\frac{1}{2}\cdot 408kg\cdot V_f^2+\frac{1}{2}\cdot 635kg\left(\frac{V_f}{3}\right)^2$$ $$130m^2/sec^2=Vf^2$$$$130\frac{m^2}{s^2}=V_f^2$$ $$Vf=11.4m/sec=25mph$$$$V_f=11.4\frac{m}{s}=25mph$$ Since the Flyer II needed 28-30mph to become airborne, the catapult cannot launch the unpowered flyer by itself, even excluding friction and drag.