While flying between 2 points 120 nautical miles apart, after about 40 nautical miles we discover we are 5 nautical miles off to the right of the track.
What heading correction is necessary in order to reach the destination? How do you calculate the correction using a circular flight computer?
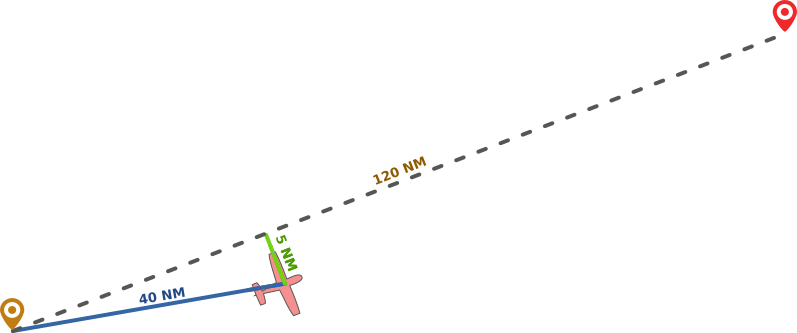
1 - First I calculate the track deviation angle:
To find the track angle:
outer scale: distance off track (NM) in this case 5 NM
divided by
inner scale: distance already travelled(NM) in this case 40 NM
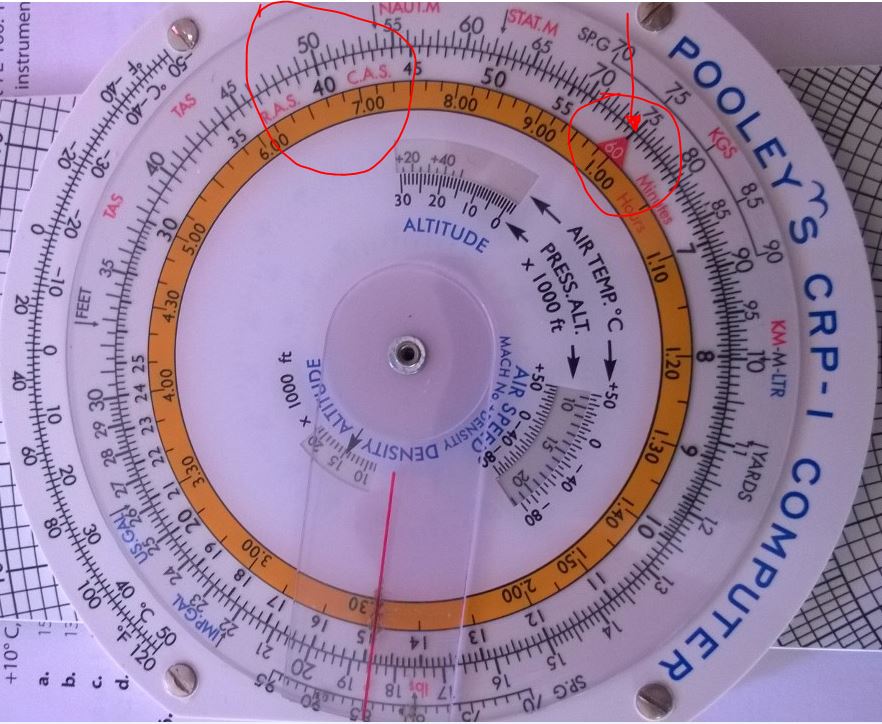
To do this I divide 5NM per 40NM as per the picture below, the division is within the red circle and the result is pointed by the red triangle that says '60' on the other red circle with the arrow pointing to it.
the result is 7.5 as you can see on the picture below.
2 - Then I calculate the closing angle for the remainder of the journer:
To find the closing angle:
outer scale: distance off track (NM) in this case 5 NM
divided by
inner scale: distance to go (NM) in this case 80 NM
The remainder of the journey is (120NM - 40NM) = 80NM The current deviation which is 5 NM.
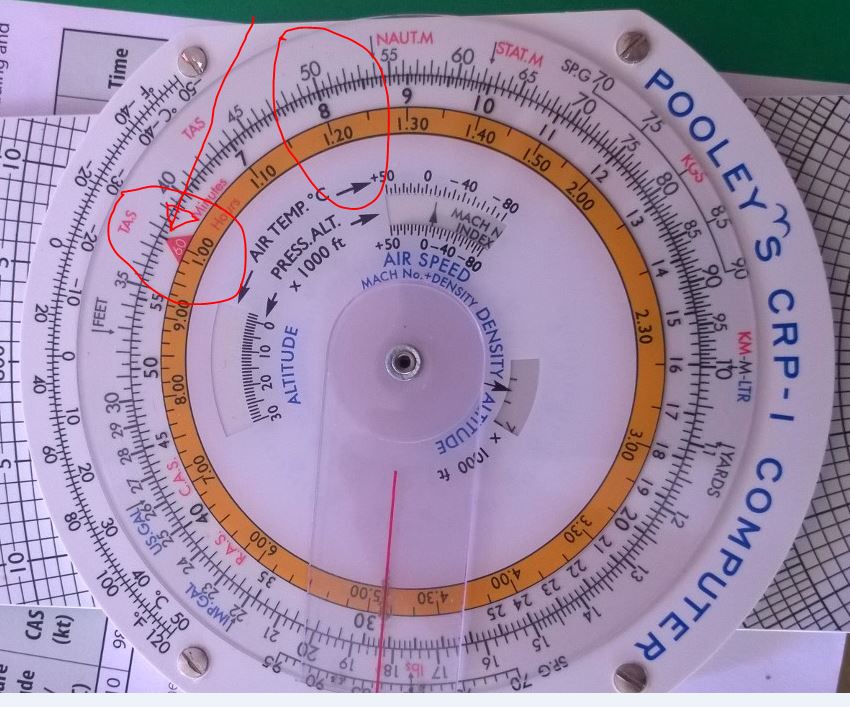
To do this I divide 5NM per 80NM as per the picture below, the division is within the red circle and the result is pointed by the red triangle that says '60' on the other red circle with the arrow pointing to it.
the result is 3.77 rounded down to 3.7 as you can see on the picture below.
3 - Then I calculate the total heading correction:
total heading correction to reach the destination = track error + closing angle
that is:
7.7 + 3.7 = 11.2 degrees
so the heading must be corrected to 11.2 degrees left.
