Why do shockwaves extend past the body that created them? As seen in this photo, the shock doesn’t stop in the air the plane is effecting, but continues on. I always assumed it was high pressure air from the shock extending out, but now I’m not too sure.
Pretty pictures
You aren't going to believe this answer, but it is true!
Think about a simple 2D diamond airfoil. We will think of this as an inviscid problem for now.
The flow in region 1 is all parallel. Start by considering the streamline just above the centerline. When that streamline encounters the airfoil, it must turn by angle $\epsilon$. This abrupt turning is what causes the shock.
Now consider the 'next' streamline. In region (1), it must be parallel to the first streamline -- parallel to the centerline. This streamline never encounters the airfoil. Instead, it encounters the inner streamline when that streamline has been forced to turn. The inside streamline turns, so the next streamline must turn.
Consequently, all the flow in region (1) is parallel. Also, all the flow in region (2) is also parallel. The shock exists to abruptly turn the flow by the angle $\epsilon$ -- it also causes a pressure jump and other changes in properties.
If this was all there is to it, region (1), the shock, and region (2) would go on to infinity!
Every streamline needs to turn because the streamline 'inside' of it turned. Every streamline acts like the rigid wall along the centerline and airfoil surface.
Next, lets consider some things that make this break down a bit.
Between regions (2) and (3) is an expansion fan. The fan acts to turn the flow the other way (convex corner) and it accelerates the flow. Although the streamline at the body will turn abruptly, the streamlines further out in the expansion fan will turn gradually.
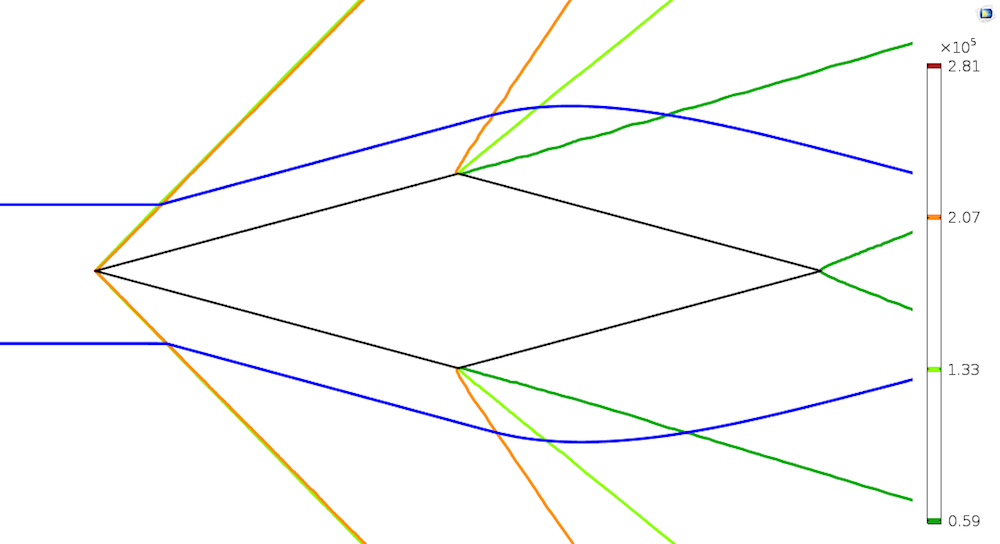
Notice that the angle of the 'start' of the expansion fan is such that it will eventually intersect with the oblique shock.
This is where things get really interesting.
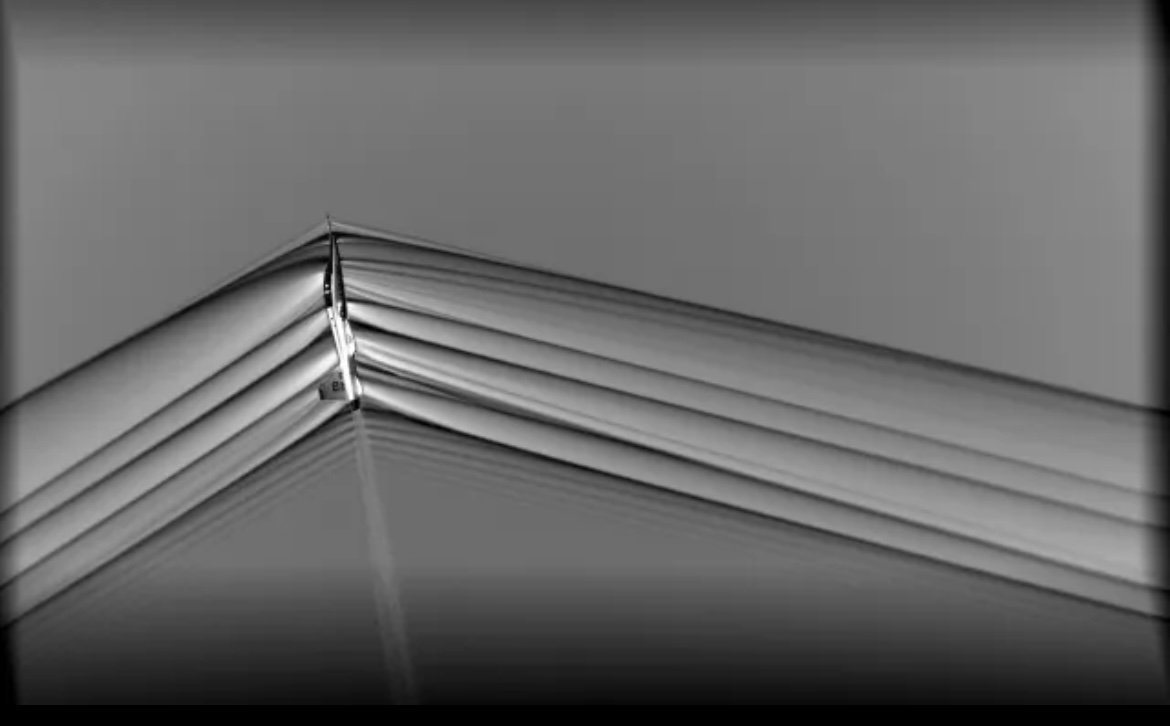
Notice that most analysis, drawings, and other depictions stop with the very nearby vicinity of the flow like the first image.
When the expansion fan meets the oblique shock, things get interesting. Recall that if the shock turns the flow 'up', the fan turns the flow 'down'. When the meet, it has the effect of allowing the 'next' streamline to turn a little less. Instead of turning $\epsilon$, it turns just a tiny bit less, and the next streamline turns less, and less for each one out. This creates a region with a curved shockwave. Each shockwave further out is a little weaker because it doesn't have to turn quite as much as the one before it.
Another bit of reality is that airplanes are 3D, not 2D. So, airplanes behave more like the cone shock equations not the wedge shock relations.
We can think of conical flow as being axis-symmetric. A streamline in 2D is really a cylindrical stream surface in 3D axis-symmetric flow. However, when a flow turns 'out' from the centerline, the area available to it increases. This is the fundamental reason why cone shocks are different from wedge shocks.
We call this a 3D relieving effect. Streamlines further from the body have more room to move to. This means that a 3D shock is really very different from a 2D shock.
