1
$\begingroup$

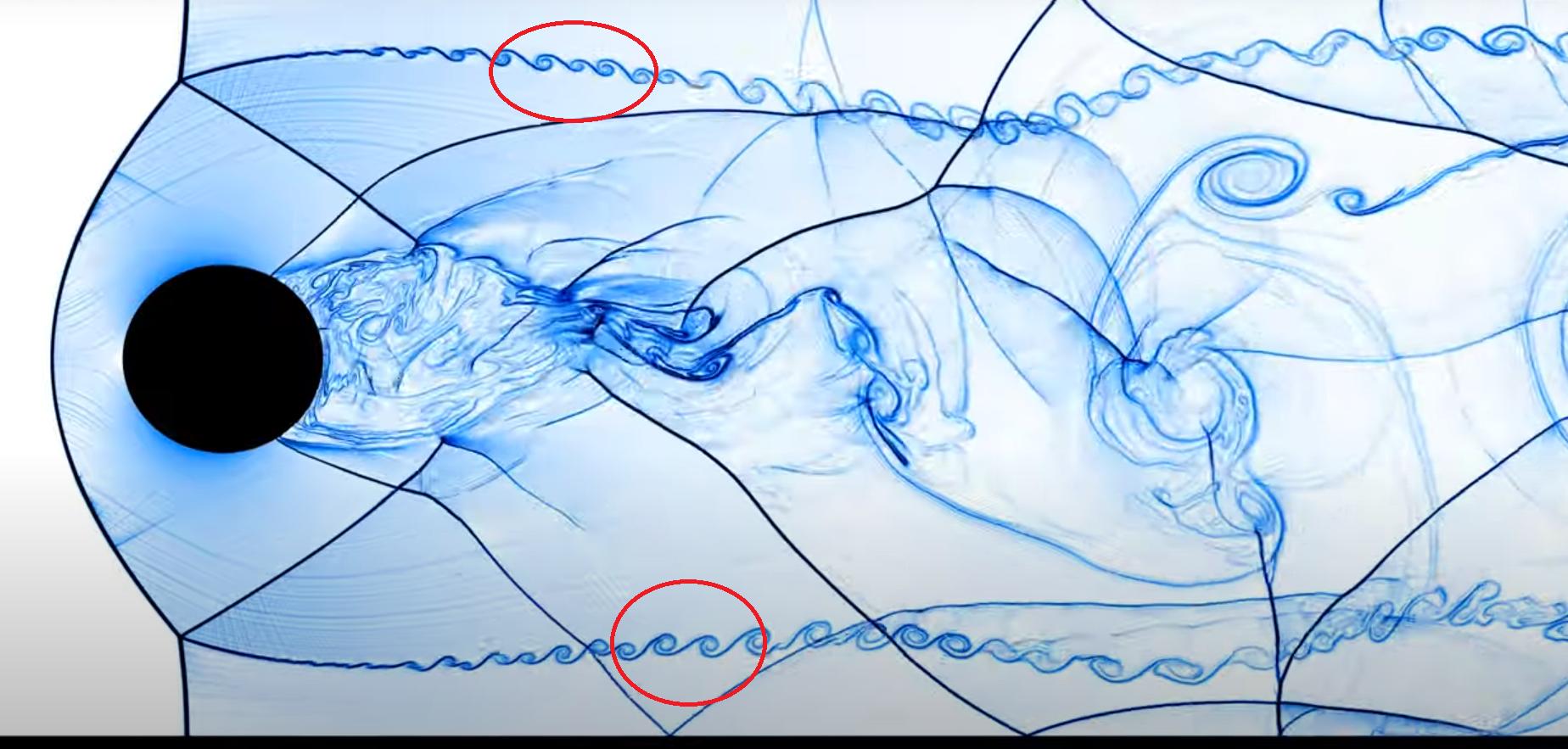
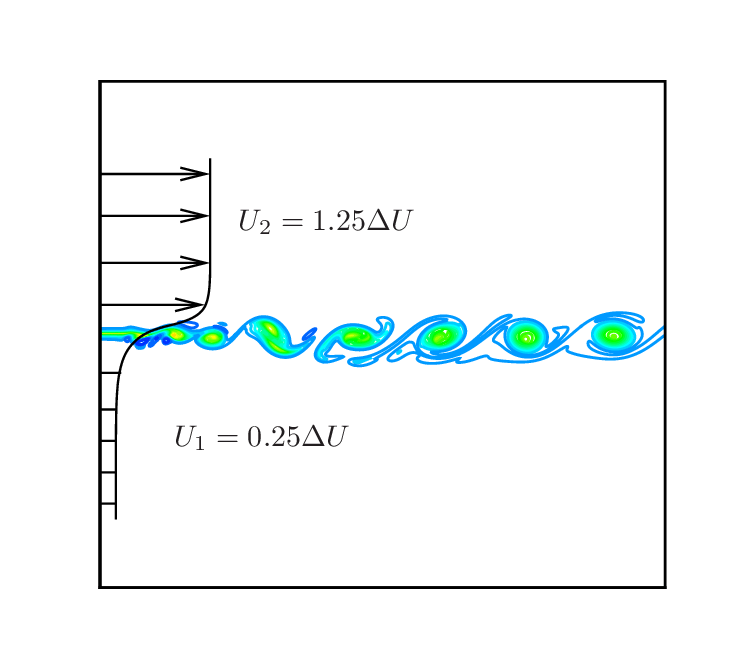
What are these little vortex patterns from on the slip line of these shocks? At first I thought it was vortex shedding, but it looks a tiny bit different to me (than vortex shedding).

You can see the slip line bending towards the middle (slightly), so would that have something to do with the creation of the vortices? Thanks. (This picture was at Mach 3)

Link to original video.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

This flow pattern is typical for shear layers. The flow speed jumps across the layer and mixing causes those regular eddies to form. See here for more.

shear layer simulation

Graphical representation of a shear layer simulation (source)

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ Is this a viscous phenomenon? $\endgroup$
    – sophit
    Commented Mar 9 at 19:19
  • $\begingroup$ @sophit good question; I would think it is, not sure. $\endgroup$
    – Wyatt
    Commented Mar 9 at 20:15
  • 1
    $\begingroup$ Everything to do with a shear layer is viscous phenomena. This is a transition -- the shear layer is at a Reynolds number where it starts laminar (to the left) but some eddies are forming -- but it isn't quite enough to go fully turbulent. $\endgroup$
    – Rob McDonald
    Commented Mar 10 at 6:45
  • $\begingroup$ @RobMcDonald: I was almost certain but then how does this match with the claim from the owner of the simulation claiming to have used "compressible Euler equations (meaning no viscosity)"? $\endgroup$
    – sophit
    Commented Mar 10 at 9:22
  • 1
    $\begingroup$ I can't go into depth (it has been more than 20 years since I took a CFD course), but there is a numerical phenomena called 'Artificial Viscosity' where the error in the way the equations are solved can manifest itself in the same way a viscous term would appear in the equations. Sometimes this is important -- it is what causes an Euler code to separate at the trailing edge of an airfoil without an explicit Kutta condition. Choosing a method that is either even or odd (order of dominant error term) will result in a method that has either dissipation and dispersion characteristics. $\endgroup$
    – Rob McDonald
    Commented Mar 10 at 19:25

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .