Did you try googling 'lambda shock'? I suggest you do an image search, there are a lot of explanations out there.
Here is an interferometry image. Not as pretty as a shadowgraph or Schliren, but still very informative. If you google around, you will find many more -- but often of even more complex phenemona.
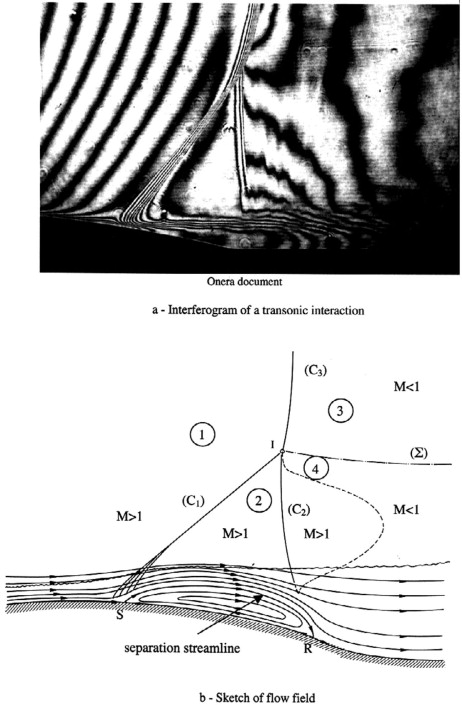
Region 1 is upstream of the shock (C1).
Shock C1 causes an adverse pressure gradient that causes a flow separation bubble. Flow separates at S, but reattaches at R.
Inside the bubble (the streamline connecting S to R), there is a recirculation zone. Flow outside the bubble keeps going.
A second shock (C2) forms on the downstream side of the bubble.
These shocks meet at point 1, and a combined shock (C3) extends to the freestream.
The surface $\Sigma$ is a slip line between zones 3 and 4. Velocities will be parallel across that line. Pressures will be equal across the slip line. Temperature, density, entropy, etc. will be different across the slip line.
You should check out An Album of Fluid Motion. Image 246 for example.
Also, here is a great video (from a computation) of flow in a shock tube.
Initially, flow is everywhere at rest in a section of tube. The top of the tube is far above us (we are zoomed in on the bottom wall). The right-side of the tube is closed. To our left, there is a membrane separating a region with 100x the pressure. That membrane is popped and the shock starts propagating down the tube (to the right).
When the shock passes a location, it induces a velocity to the right.
Eventually, the shock hits the wall at the right of the tube, it reflects back to the left.
Now, instead of entering a region of stationary air, the shock is encountering a flow. Since the bottom wall of the tube is there, the flow has built a boundary layer along the bottom wall.
The shock causes the boundary layer to separate, which forces some turning -- forming an oblique shock on the bottom wall that forms a lambda structure.

