I was just brushing up on basic aerodynamics and I have something I want to clarify about flying in the region of reverse command. I went up in my 172 the other day and noticed the following while practicing slow flight:
- I'm on straight and level flight, and I enter slow flight (dirty) the way I usually do. I pull the power back and then I raise the nose to a level attitude slightly to bleed off some airspeed while maintaining the same altitude. When I entered the back side of the power curve, I pitched for an airspeed that was significantly above Vso, but below L/D max, which in this case happened to be 55-56 Knots. However, this time I didn't add any power and I just pitched for 55 knots to see what would happen. The airplane started descending (sinking), which is really what I expected it to do.
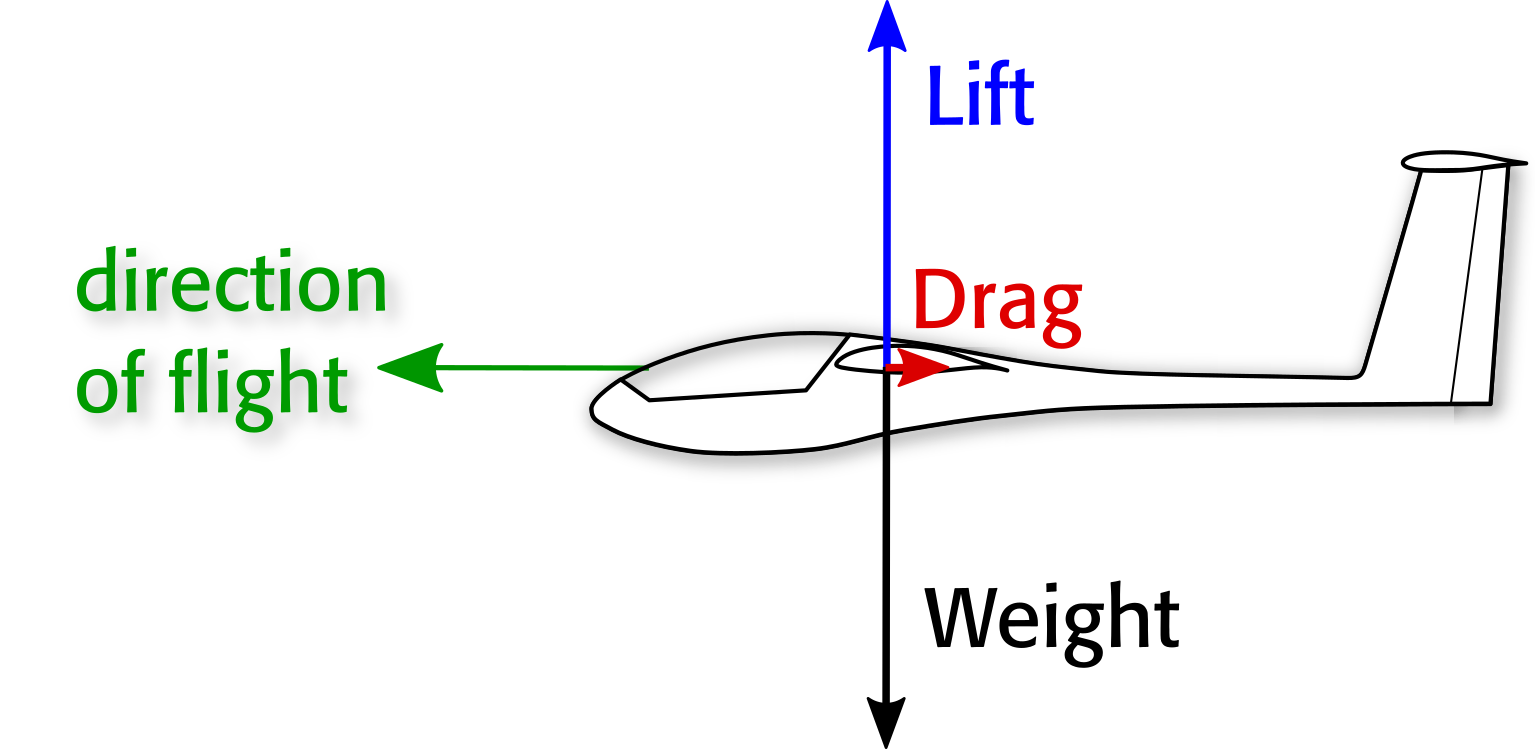
My limited understanding of why this happens is that total drag (mostly induced drag at high AOA) exceeds thrust available and the airplane develops a sink to try to regain equilibrium. Is this correct? Can someone with more knowledge explain why this happens? Maybe I'm overthinking it... I just know that high drag at low airspeeds typically results in a high sink rate. I just can't explain exactly why. Some people have said that weight exceeds lift, but I'm pretty sure this isn't the case as the airplane is not stalled.