How does turn radius change with velocity between Vm and Vs, as in a 180 degree turn in a canyon?

Question

I just heard a briefing on mountain flying, and it was stated that the first thing, or the most important thing to do in the event you are unfortunate enough to find yourself in a blind canyon with no safe way out straight ahead, [ and you need to turn around and go back the way you got in, is to pull power.

To address comments made in some of the answers below, I am asking about a situation in a General Aviation (GA) aircraft, where you do NOT have sufficient airspeed/aircraft performance to "go over the top" i.e., perform an Immelmann/Cuban Eight or Hammerhead.

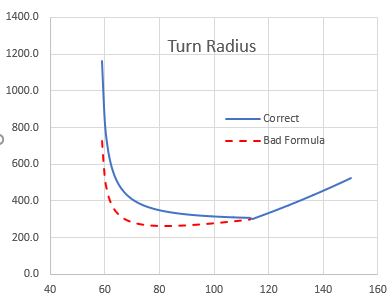
It was stated that this is the recommended approach because the turning radius of the aircraft is dependent on velocity and therefore that the slower you are going the tighter the turn, and the less distance it will take to turn around. (I suspect this might be based on a simplistic reading of the Turn Radius formula $R= V^2 / G$, without considering that below Maneuvering Speed ($V_M$), $G$ also varies with $V^2$).

NOTE. By G, I mean the aircraft G, or Load Factor (engineers use the letter N), which is equal, generally, to Lift divided by aircraft Weight, and specifically, in this issue, the radial G, or that horizontal component of the total aircraft G which is turning the aircraft, and not just keeping it in the air.

I have always thought just the opposite, (about the power setting), for a variety of reasons, and decided to do the physics/aerodynamics analysis and see what that predicted.

Without going through the Math, what I ended up with was that the turn radius in a maximum performance turn, (AOA at $C_{Lmax}$), would be

$$R \cong \frac{V^2}{\sqrt{V^2-V_s^2}}$$

where:

1. $R$ .... Turn radius
2. $V$.... Aircraft true air speed
3. $V_S$... Stall speed (TAS)

This is based on the assumption that we are below maneuvering speed ($V_M$, or what we called $V_c$ (Corner Velocity) in the USAF), so we are limited by stall AOA and not placard G-Limits, and that we need to maintain level flight or at least a controllable rate of descent. i.e., we need to maintain a bank angle no greater than that which would generate a vertical component of Lift sufficient to keep the nose from dropping any further. Therefore, the slower and closer to stall we get, the less angle of bank we can hold (and the less of our lift is actually turning the aircraft). As I remember this from the Air Force, turn radius is constant below $V_M$, and therefore, the most critical aspect of this issue is preventing stall, loss of control, and spinning into the ground. plus, keeping the airspeed as high as possible (below $V_m$) allows us to use the highest possible bank angle, where we will get the greatest possible horizontal component of wing lift to turn us around.

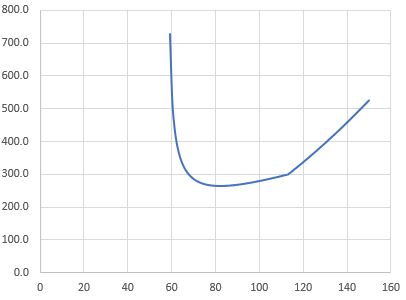
When I graph the above equation, I get what I expected, that below $V_M$, the turn radius is more or less constant, except that the closer to stall you get, the bigger it gets (which makes sense, at stall, you cannot turn at all!) For an aircraft with a stall speed of about 59K, The graph looked like the below: Vertical is turn radius (ft) and horizontal is true airspeed):

The kink in the graph at about 112Kts is because I assumed a $3.8 G$ aircraft, so $V_M$ would be $V_s \sqrt{3.8}$ or about 112 Kts. After that we are limited by placard G, and not AOA, and Turn Radius is just $V^2/G$.

So, if you notice, contrary to my expectations, the turn radius is not roughly constant below $V_M$, or, considering bank angle, gradually increasing the entire way as you slow from $V_M$ to $V_S$. No, it gradually decreases at first, (although not by a lot), until reaching a minimum about 25 kts above $V_s$, and then does as I expected, it rises asymptotically to the Stall speed line.

So, my question is, why, from a physical perspective does the turn radius first decrease as we slow from $V_M$ to $V_S$? Is there a physical explanation for this phenomena?

This does not affect the overall conclusion, (it still absolutely makes sense to add power to minimize airspeed bleed off and stay as far from the stall as possible, rather than intentionally reduce power, slow down and risk stalling), but if I am going to explain this, then I should be able to explain why the curve behaves as it does from a pilot perspective.

Answer 1

NOTE:. I have resolved my question. I had made a mistake in the algebraic derivation of the Formula I was using to graph this. I have included both the old incorrect formula and the new corrected one, and displayed on the graph the curves resulting from each one.

Without going through the Math, I noticed that my equation was wrong:

WRONG FORMULA: $$R \cong \frac{V^2}{\sqrt{V^2-V_s^2}}$$ CORRECT FORMULA: $$R \cong \frac{V^2 V_s^2}{g (\sqrt{V^4-V_s^4})}$$

where:

1. $R$ .... Turn radius
2. $V$.... Aircraft true air speed
3. $V_S$... Stall speed (TAS)
4. $g$ .... 32.2 $ft/sec^2$

Once I modified the formula properly, the curve indicates exactly what you would expect, so my question is now moot.

Derivation:
Staring with the standard Turn radius formula:

1. $$R \cong \frac{V^2}{G_R}$$

where $G_R$ is the Turning Lift (the horizontal component of the Lift vector), divided by the weight, referred to as Radial G, the G that is actually turning the aircraft and not just holding it up in level flight.

Since the Aircraft Load Factor or total G ($G_T$), radial G ($G_R$) , and the 1 G (god’s G) that is holding the aircraft up in the air form a 90 degree right triangle depicted in the diagram above, they must conform to Pythagoras Theorem that says that in a 90 degree triangle the square of the hypotenuse must be equal to the sum of the squares of the other two sides. so,

$G_R^2 + {1g}^2 = G_T^2$

$G_R = \sqrt{G_T^2 - {1g}^2}$

Back to turn radius formula, substituting expression for $G_R$,

2. $$R \cong \frac{V^2}{\sqrt{G_T^2 - {1g}^2}}$$

Now total G ($G_T$) - assuming we establish the maximum available G at whatever airspeed we are at, is simply the maximum available Lift ($L = C_{Lmax} ρ V^2 S$), divided by the aircraft weight

$G_T = g\frac{C_{Lmax} ρ V^2 S}{W} $

Substituting this into our equation gives us

3. $$R \cong \frac{V^2}{\sqrt{{g^2\frac{(C_{Lmax} ρ V^2 S)^2}{W^2}} - {1g}^2}}$$

Now here's the trick, The maximum available lift at stall speed $V_S$ is, by definition, equal to the aircraft weight, so

$W = C_{Lmax} ρ V_S^2 S$

Substituting this, and simplifying,

4. $$R \cong \frac{V^2}{g\sqrt{{\frac{(C_{Lmax} ρ V^2 S)^2}{(C_{Lmax} ρ V_S^2 S)^2}} - 1}}$$

and cancelling,

5. $$R \cong \frac{V^2}{g\sqrt{{(\frac{V^2}{V_S^2})^2} - 1}}$$

Simplifying,

6. $$R \cong \frac{V^2}{g\sqrt{{\frac{V^4}{V_S^4}} - \frac{V_S^4}{V_S^4}}}$$

and finally,

7. $$R \cong \frac{V^2 V_s^2}{g \sqrt{V^4-V_s^4}}$$

Answer 2

The advice to pull power before turning is only good if you cannot climb. What I learned is to pull up and then do the turn at low speed, but a higher altitude. In normal canyons, this should also give you more maneuvering space.

When flying gliders in the Alps, you learn the "Bayernkurve" (Bavarian turn). This is almost a hammerhead turn, but not flown vertically like a real hammerhead, but rather with the steepest climb angle possible. Flying in a steep climb will lower the load factor and help to make the turn tighter.

Not only should speed be as low as the load factor allows, you should fly at the highest lift coefficient possible. This means to use all lift enhancing devices at your disposal, which in a climb includes thrust. Flying with full flaps increases drag, which is another reason not to cut thrust.

Now to the formula for the smallest turn radius. This assumes quasi-stationary conditions, so no climb and no inertial effects are included. But even with those restrictions, it shows what the limiting parameters are. We start with the lift equation which now includes the centripetal force to fly a turn with the radius $R$: $$L = \frac{\rho}{2}\cdot v^2\cdot S_{ref}\cdot c_L = \sqrt{(m\cdot g)^2+\left(m\cdot\frac{v^2}{R}\right)^2}$$ To arrive at a formula for the radius, we need to isolate it on one side: $$R^2 = \frac{m^2}{\left(\frac{\rho}{2}\cdot S_{ref}\cdot c_L\right)^2-\left(\frac{m\cdot g}{v^2}\right)^2}$$ To simplify this, we can express the speed $v$ by the lift coefficient like this: $$c_L = \frac{m\cdot n_z\cdot g}{\frac{\rho}{2}\cdot v^2\cdot S_{ref}} \implies v^2 = \frac{m\cdot n_z\cdot g}{\frac{\rho}{2}\cdot S_{ref}\cdot c_L}$$ in order to arrive at $$R^2 = \frac{m^2}{\left(\frac{\rho}{2}\cdot S_{ref}\cdot c_L\right)^2\cdot\left(1 - \frac{1}{n_z^2}\right)}$$ The only variable parameters for a given airplane and height are (besides the radius) the lift coefficient and the load factor. In order to minimize the radius, the parts in the denominator on the right side need to be maximized: $$R_{min} = \sqrt{\frac{m^2}{\left(\frac{\rho}{2}\cdot S_{ref}\cdot c_{L_{max}}\right)^2\cdot\left(1 - \frac{1}{n_{z_{\:max}}^2}\right)}} = \frac{m\cdot\frac{n_{z_{\: max}}}{\sqrt{n_{z_{\:max}}^2-1}}}{\frac{\rho}{2}\cdot S_{ref}\cdot c_{L_{max}}}$$ So what does this tell us? In order to fly a turn with the smallest possible radius your airplane needs to have a low wing loading $\frac{m}{S_{ref}}$, you need to fly low (high air density $\rho$) and at the highest possible load factor $n_z$ and lift coefficient $c_L$.

Depending on the maximum load factor, speed will still be high compared to that at slow level flight. How high can be seen in a turn rate diagram. It differs from your diagram, but not so much: Use the lines of equal radius (which are radiating away from the origin) as the basis of the Y-axis and distort the result such that those lines become parallel, and you will end up with your diagram.

Turn rate diagram (own work) for a small aircraft. The bold, coloured lines represent the maximum stationary load factor over speed for a given engine thrust at various thrust characteristics over speed. The fine coloured lines are lines of equal load factor and the fine straight, black lines radiating from the origin are those of equal turn radius. It can be seen that the minimum instationary turn radius is achieved at the combination of maximum load factor and minimum speed; however, due to the high load factor the speed is quite a bit higher than the minimum speed in level flight. Also, the available thrust is not sufficient for stationary flight, so this point will incur an energy loss which needs to be made up for by adding sink speed or bleeding off climb speed.

my question is, why, from a physical perspective does the turn radius first decrease as we slow from V$_M$ to V$_S$? Is there a physical explanation for this phenomenon?

As you approach the point of the smallest turn radius from high speed by slowing down, $c_L$ will increase and, being in the denominator, will let the radius shrink. Moving further to lower speeds, away from the point of smallest radius to lower speeds, means that both the lift coefficient $c_L$ and the load factor $n_z$ will decrease, so the term $\frac{n_{z_{\: max}}}{\sqrt{n_{z_{\:max}}^2-1}}$ in the numerator will grow while the denominator decreases. This means that with lower speed R will increase again. The turn radius should be smallest at manoeuvring speed, which you call V$_M$ but should more properly be called $\text{v}_A$. Since your equation expresses the maximum lift coefficient in terms of stall speed at 1g, let me modify my equation accordingly with an expression that is valid for the flight regime at maximum lift coefficient (left of the kink, so to speak): $$R = \frac{m\cdot\frac{n_z}{\sqrt{n_z^2-1}}\cdot g\cdot v^2}{\frac{\rho}{2}\cdot S_{ref}\cdot c_{L_{max}}\cdot g\cdot v^2} = \frac{v^2}{g\cdot\sqrt{\left(\frac{v^2}{v_{min}^2}\right)^2-1}} = \frac{v^2\cdot v^2_{min}}{g\cdot\sqrt{\left(v^4-v_{min}^4\right)}}$$ where $v_{min}$ is the stall speed in level flight. If you look at the units of the parameters on both sides, you will find that the equation above gives a length unit on both sides whereas that is only true for your corrected equation (which I can confirm as correct). There should be no decrease in turn radius as you move away from the kink.

Answer 3

Chandelle. If you are not familiar with the maneuver, it is a requirement for the Commercial license and is very useful in such a situation. It is a climbing 180 degree turn that exchanges airspeed for altitude while decelerating the aircraft down to a slower airspeed, and thus allowing for a tighter turn radius. Personally I would rather exchange that excess airspeed for altitude rather than pulling the power back, but no advice given here today is necessarily valid for the particular day you are flying, specific aircraft you are flying, weather, temperature, density altitude and severity of the situation you are in. Yeah, I know, that sounds like a legal disclaimer, but it is really the truth - a chandelle is awesome if you have the room to maneuver in the particular aircraft you are in. It might be fantastic in a STOL aircraft but perhaps you are in a Baron going 180 KIAS in a narrow canyon and have waited far too long to make your turnaround decision, so in the end, "the PIC is the sole authority for the safe operation of the aircraft". For what it's worth, practice chandelles to give yourself the skills you need should the need arise. https://en.wikipedia.org/wiki/Chandelle NOTE: What I am discussing is similar to that which is found under "CRUISE FLIGHT – BOX CANYON TURN" located at https://www.mountainflying.com/Pages/mountain-flying/box_canyon_turn.html The general idea is a climbing turn, into the wind, to reduce your progress toward the terrain by slowing your aircraft to increase turn radius while gaining altitude - and I wouldn't recommend slowing below Vx, but again, YMMV. If you think there is a better method, please respond with that method rather than suggesting "nah, that won't work", and remember, all things are variable - no one can give you a pre-planned suggestion here that will work on the day you are flying, in the aircraft you are flying, with the weather you are in, the density altitude, and the exact distance from the terrain and the airspeed at which you are operating. If you are already that far behind the aircraft in your planning, best of luck in any escape maneuver you perform.