I'm afraid that a thorough answer requires the contents of chapters 2 and 3 of Leishman, but here is an outline. The relationship between power and true airspeed from this answer:
Power equals torque times angular velocity of the rotor: $ P = Q * \Omega$. Most helicopters have constant speed rotors, so the relationship between torque and speed would be similar to the power curves.
Rotor blade length and pitch appear as follows. Power P and torque Q equations for momentum theory in the hover:
$$P = C_P \cdot ½ \rho A (\Omega R)^3$$
$$Q = C_Q \cdot ½ \rho A (\Omega R)^2 R$$
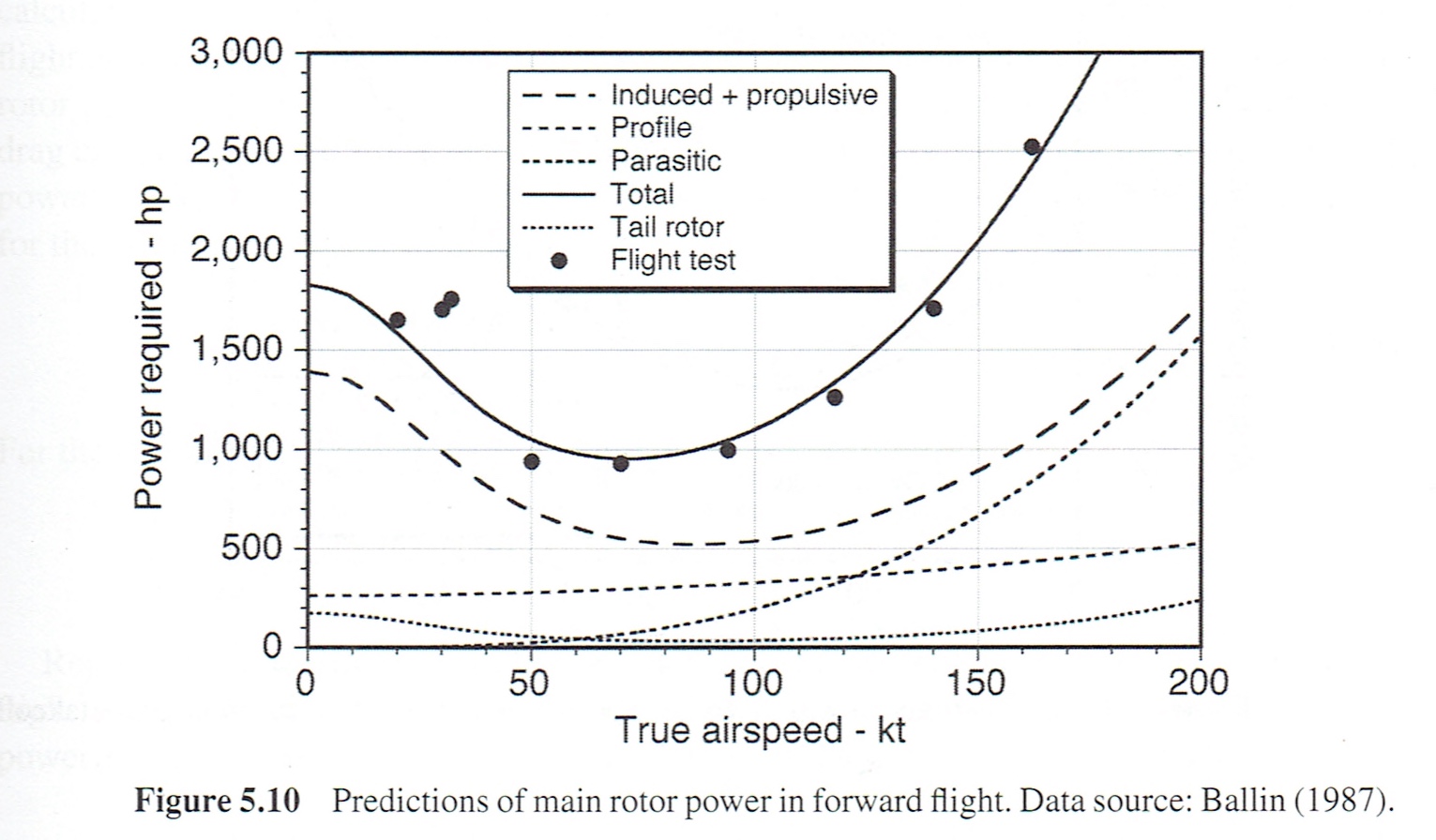
At forward speed the momentum based power becomes less due to lower induced power requirements. An inflow equation relates forward velocity of the helicopter with blade tip speed - too much scope for an answer here.
Blade length appears in the rotor disk area A. Torque and power are functions of the rotor solidity $\sigma$, defined as (total blade area)/(disk area). Note that $C_P$ is always the same value as $C_Q$
From Leishman:

..if the amount of lift a helicopter generates changes dependant on pitch, how much does the pitch change..
Thrust T of the rotor Again from Leishman:




