How can I find the minimum and maximum airspeeds for efficiency with a specific prop/engine combination?

Question

I'm operating a Cessna 180 with various wing and weight mods with a TCM IO550D engine and a McCauley Black Mac (D3A34C401/90DFA-2 3 blade (88 inch) seaplane prop. I operate Lean of Peak (LOP) for most ops, and accept some airspeed loss for the benefits of LOP. At some point of loss of airspeed, the resultant inefficiency of the power plant is counter to any fuel efficiency gained by LOP. I'm trying to understand where this airspeed intersects, at a given altitude.

A second part of the question, is what factors affect efficiency loss at top power settings in both cruise and for takeoff? For example, continuous, unlimited full rated 300 Hp is only available at WOT and 2700 RPM, but it is insufferably loud at that RPM. McCauley indicates the prop's max pull is at 2600 RPM. What am I gaining or losing by taking off, or flying with 2600 RPM vs 2700 RPM? Is there a useful chart for evaluating engine/prop combinations?

Answer 1

What you really need to do this analysis is a detailed propeller map and a detailed engine map. Then you can do some analysis to match them together and make your own combined map.

Piston engine maps are often included in POH or other aircraft documentation, you can probably find one for your IO550D fairly easily.

Prop maps are much harder to come by. However, you may be able to obtain one by contacting the company.

Although I use the word Map -- implying a piece of paper with a chart on it, it could also be provided as a table of numbers -- or these days as a computer program that performs interpolation between points of an internal table of data.

Edit to discuss chart linked to by OP in comments.

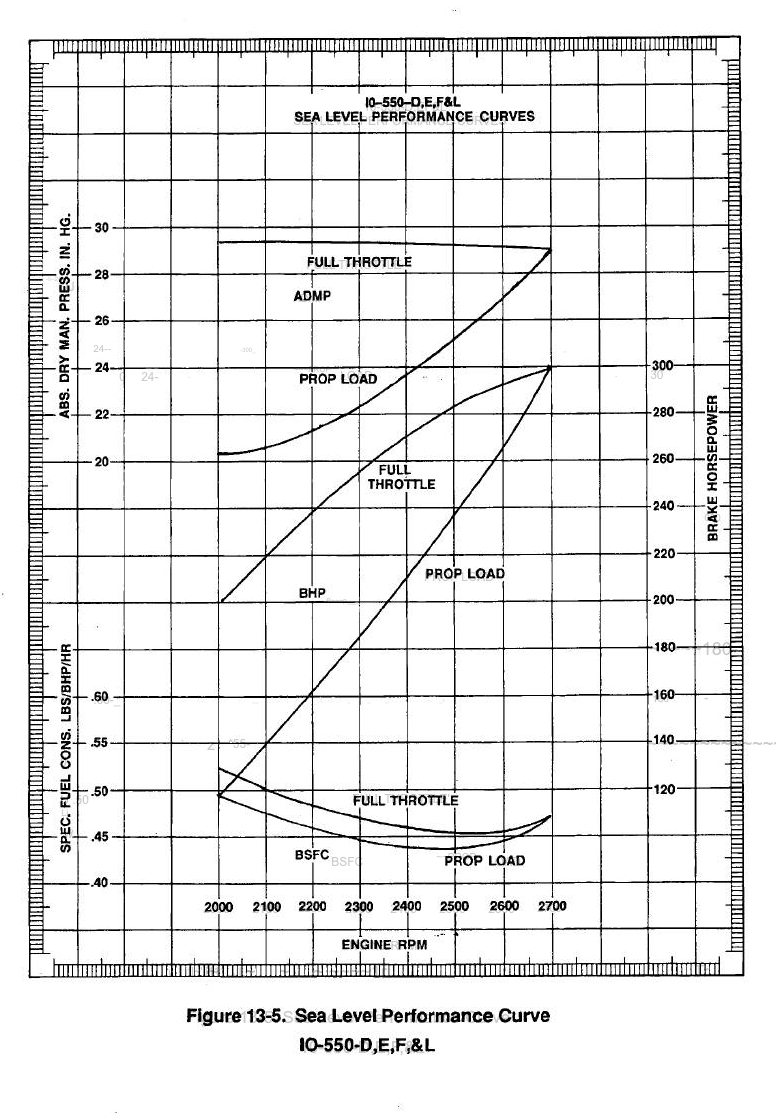
This is a simplified performance chart for this engine -- the important thing about this chart (that is not explicitly stated) is that it is for performance with a (particular) fixed pitch prop attached.

A different style of presentation would be used with a constant speed prop.

At the top of the page, the altitude is specified. This chart is also for a specific airspeed -- but unfortunately, that is not indicated anywhere I can see on this page.

The bottom two curves use the vertical scale at the lower left for BSFC. The middle two curves use the scale at the right for BHP. The top two curves use the scale at the upper left for manifold pressure.

All of these quantities are plotted as a function of RPM (the x-axis).

There are two curves because one represents the engine's performance at full throttle. The other represents the engine's performance with a fixed pitch propeller attached (at sea level and a particular airspeed).

The full throttle line represents the maximum operating capability of the engine -- in this case, it looks like it is mainly operating at about 29 inHg MP. If it were attached to a brake in a dynamometer, these are what the power and fuel flow curves would look like as RPM is varied.

However, the chosen fixed pitch propeller can't absorb 240 Hp at 2200 rpm. Instead, at 2200 rpm, it only absorbs 160 Hp with the engine at 23 inHg.

If the pilot advances the throttle (say to 200 Hp), the engine will produce more power than the prop can absorb, so there will be an excess torque applied to the shaft, and the propeller will accelerate to a faster rpm -- until 2350 rpm.

The prop is the load applied to the shaft -- the engine is the thing trying to drive the shaft. They must always match RPM (or the shaft is broken). The torque in and out must also balance -- knowing that you can accelerate / decelerate when out of balance. Just like with forces.

For a propeller, we have the advance ratio $J$, it is a function of the true airspeed ($V$), the engine speed ($n$) (in revolutions per time, not radians per time), and the propeller diameter ($D$).

$$J=V/(n\,D)$$

In many ways, the advance ratio is like the angle of attack of a wing.

For a fixed pitch prop, the power coefficient is a function of the advance ratio. This is like saying the drag coefficient is a function of the angle of attack.

$$C_P=f(J)$$

There is also a thrust coefficient -- but I'm ignoring that for now. The thrust coefficient would be like the lift coefficient in this analogy.

For a variable pitch prop, the power coefficient is also a function of the blade angle. But this chart is for a fixed pitch prop.

$$C_P=f(J, \beta)$$

The power coefficient is dimensionalized to shaft power like this

$$P_{shaft}=C_P\,\rho\,n^3\,D^5$$

This is entirely analogous to dimensionalizing the drag from the drag coefficient like this

$$D=C_D\,0.5\,\rho\,V^2\,S_{ref}$$

So, in this chart, propeller performance is for a fixed pitch prop at a single altitude (i.e. density $\rho$), and a single airspeed ($V$).

Starting at low throttle (2000 rpm), we are at some $J$, which means a particular power coefficient $C_P$, which is then dimensionalized to our $P_{shaft}=120$Hp.

Advancing the throttle, each point is for a different advance ratio $J$, which means a different power coefficient, and a different shaft power.

If we were to change the airspeed (say faster), the advance ratio would change (increase) at a given RPM, so the $C_P$ would change (decrease - I haven't shown a $C_P vs. J$, but trust me). And so the power absorbed by the prop would decrease. Since the pilot did not change the throttle, the engine's output power now exceeds the propeller's absorbed power and the prop will accelerate -- we've put the airplane in a dive and may over-speed the prop.

A different airspeed would be represented by a different set of prop load curves.

Likewise for a different altitude, or a different propeller.

Answer 2

Here is a minor addition which might help a little.

Knowing the prop diameter and the speed of sound, you can calculate the shaft RPM at which the prop tips go supersonic. When that happens (or actually a little before) the thrust flattens off and the noise generation goes way, way up- and the propulsive efficiency of the prop goes down. That is, you burn more avgas but you do not get more performance. This is probably the difference between 2600 and 2700 RPM in your example.

One simple way to demonstrate this would be to affix a stout spring gauge or load cell to the end of the fuselage, tie off the other end to something heavy, power up and measure the tension force (thrust) as a function of RPM with the prop set to fine pitch between 2500 and 2700 RPM.

Or alternatively, just wait for Peter Kaempf to get out of bed (mind the time zone difference!) and furnish his answer!

Answer 3

You may want to read this paper and examine how Norris and Bauer completed a flight performance assessment of a Luscombe 8E. The Luscombe would be an approximate 7/8 scale 180, just considering the comparison, generally. Their evaluation could serve as a guide for completing a similar assessment for your 180. The paper was originally referenced in an answer by Peter Kämpf, here.

What am I gaining or losing by taking off, or flying with 2600 RPM vs 2700 RPM?

Something you might want to consider on launch is propeller stall. Propeller whirl tests to determine thrust are accomplished on a static thrust dynamometer. Consequently, the maximum thrust at a specific rpm given by the manufacturer will be static thrust. The loss of thrust at a yet higher rpm could be due to the initial onset of propeller stall, or otherwise balancing in the constant speed adjustment to reduce blade angle of attack, resulting in a slight loss of blade lift, or thrust. Once in level flight approaching cruise, however, propeller stall or an otherwise reduction in thrust, under these mentioned rpm conditions, would not be foreseen as an issue.

You mention the sound is insufferably loud at 2700 rpm. This could be due to the propeller tip velocity exceeding the speed of sound. Just as this occurs, there is a slight but noticeable drop in propeller efficiency evidenced by a loss of thrust. Do the calculations suggested by Niels Nielson to determine the tip velocity of the propeller vs rpm. Keep in mind that in flight, the vector sum of airspeed and prop rotational tip speed determines when the prop tip will exceed the speed of sound.

As you have indicated you are operating your plane with various wing and weight modifications, you may have to assess the flight performance of your plane by using PIW or VIW methods. The acronyms stand for power independent of weight and velocity independent of weight. These methods are used to back-figure various aspects of flight performance by application of relatively simple aerodynamic equations for thrust, and related factors. You may want to read this paper by Connick regarding flight testing and assessment of the performance of a Piper J4A Cub Coupe. Their assessment provides an excellent example of the process in determining flight performance factors similar to those of your interest. As a place to start, which will not cost anything, you can investigate the application of these methods using data from your POH or that for any comparable aircraft. The most important aspects are related to power required vs power available. All other factors (or most of them) can be derived from these. Rob McDonald has given you an excellent start regarding your power plant. An assessment of your propeller will determine the useable (available) power. Here is an extract of an ongoing similar POH approach estimating factors for other Cessna aircraft.

Added in edit - As additional information regarding propeller analysis, David F Rogers of NAR Associates has provided several papers available at NAR-Associates.com, which discuss aspects of propeller efficiency for different flight conditions such as takeoff, climb, and cruise. These papers are quite insightful in the examination methods for propeller evaluation.