The angle of an oblique shock is determined by the Mach number before the shock and how much the flow turns.
Usually, this is illustrated by a ramp. A 10deg ramp means the shock turns 10 degrees.
This is an oblique shock chart, it illustrates all oblique shocks for a perfect gas.
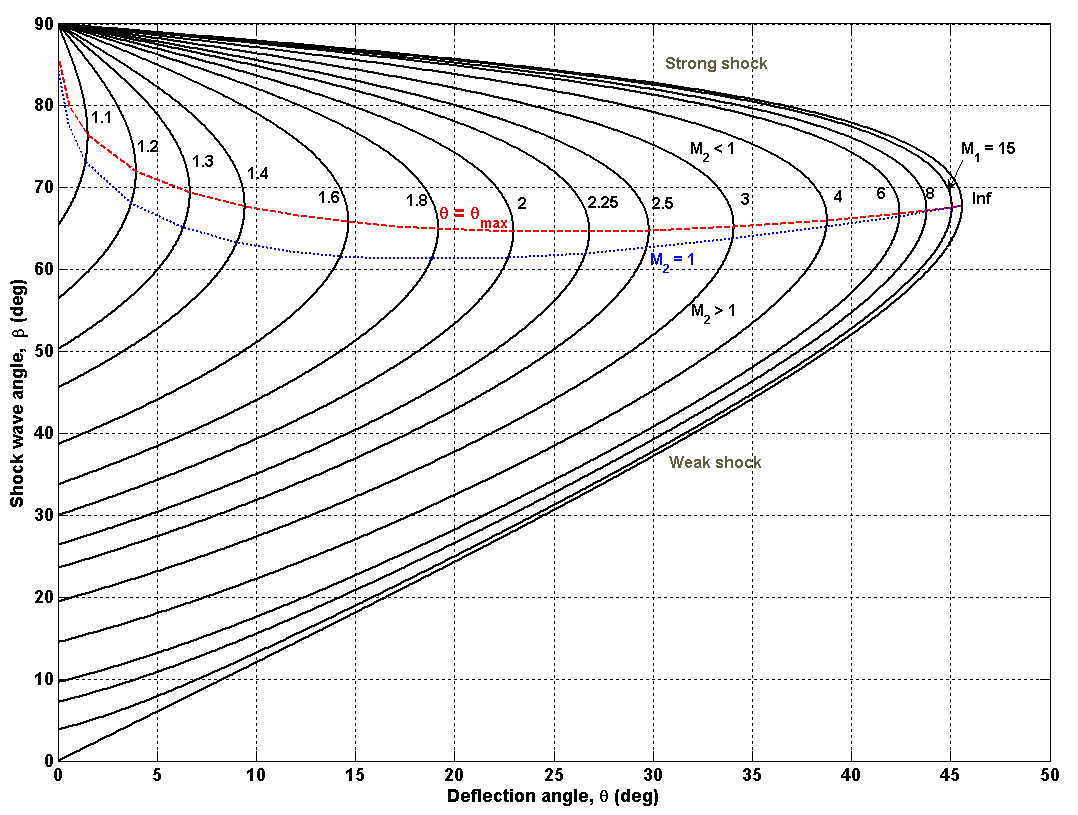
There are two solutions -- a strong shock and weak shock solution. The weak shock solution will have supersonic flow downstream of the shock. The strong shock solution will have subsonic flow downstream of the shock.
Nature generally prefers the weak shock solution unless it is forced into the strong shock solution.
So, a Mach 1.6 flow that must turn 10deg will have a shock wave angle of about 51 degrees.
This all makes sense when the upstream flow is uniform, and the downstream flow must turn the same amount.
However, imagine a situation where the flow had to turn 10deg, but the upstream flow wasn't uniform in Mach number -- lets assume it varied from 1.6 to 1.8.
Each individual streamline has its own oblique shock situation -- an intermediate streamline at Mach 1.7 that must turn 10 degrees will have a local shock angle of 47 degrees. The streamline at 1.75 that must turn 10 degrees will have a shock angle of 45.5 degrees.
Put all this together and you get a shock that is curved.
A curved shock results from a flow that has either 1) non-uniform Mach before the shock, and/or 2) the need to turn a non-uniform amount.
Edit: Stuff below about flow turning added.
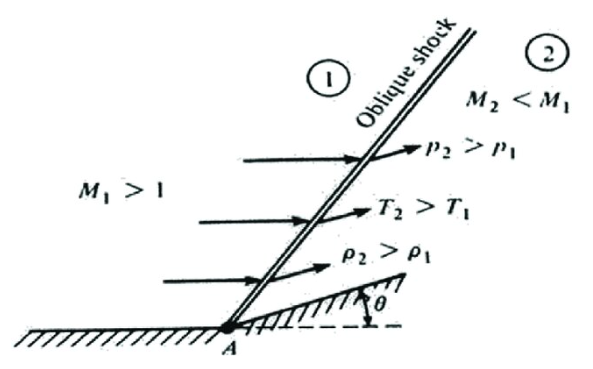
Here is a typical diagram used to explain oblique shocks. You will find this diagram and the shock chart above in any compressible flow textbook.
Region 1 is upstream of the shock. Region 2 is downstream.
Notice how the velocity vectors in region 1 are parallel to the wall at the bottom. They must be -- otherwise, the conservation of mass would be violated.
The wall bends at point A. It turns by angle $\theta$.
Notice how the velocity vectors in region 2 are parallel to the wall at the bottom. Once again, they must be -- otherwise, conservation of mass would be violated.
So, the sharp bend in the wall forces the flow to turn. This in turn forces the shock to exist. The strength of the shock -- and the shock angle are determined by the upstream Mach number and the turning angle.
The thing is, this $\theta$-$\beta$-$M$ (pronounced theta-beta-mach) relationship is true whether the wall is there or not. I.e. if you have a flow of a given Mach and a shock of angle $\beta$, then it will locally turn by angle $\theta$. Or, if you have a flow of a given Mach that turns by angle $\theta$ for whatever reason, the local shock angle will be $\beta$.
Edit: Adding lambda shock image to support comments.
The first shock of a lambda shock is the one that causes the separation.