If in a coordinated turn, the horizontal lift vector is equal to the Centrifugal force. Then how is the aircraft still turning?

Question

Image source

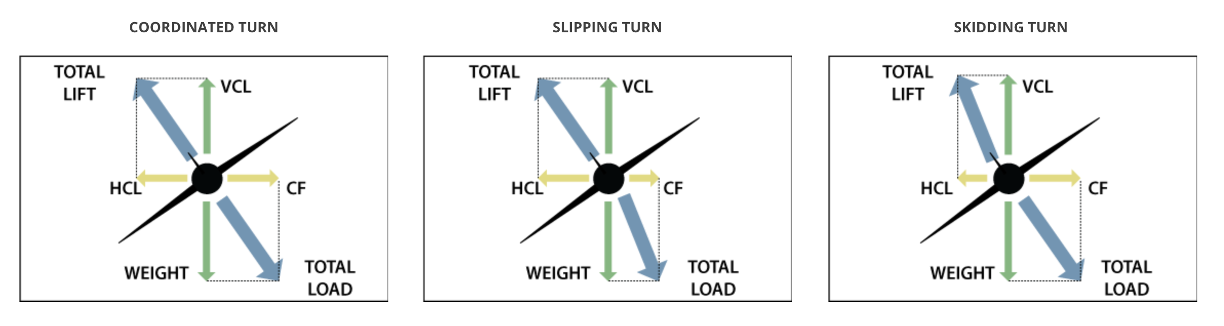
How does the Aircraft continue to turn when the both the Horizontal component of lift and the centrifugal force are equal?

Answer 1

How does the Aircraft continue to turn when both the Horizontal component of lift and the centrifugal force are equal?

Those pictures are not 100% correct.

To enter and keep a turn, a force toward the centre of the curve has to be created. In an airplane this is achieved by tilting the lift laterally, like in the following picture (source) where the airplane is turning left (as seen from the front):

The vertical component of the lift balances the weight out while the horizontal component keeps the airplane in a turn. This horizontal component is called centripetal force. The higher the centripetal force is, the steeper the turn is.

End of the story.

So what about the centrifugal force? Let's make an everyday comparison with what happen in car that accelerates. Due to the traction force the car gets accelerated forward. But what you experience as a driver/passenger is actually a backward force (aka inertia) pushing you against the seat. This is exactly the same as for our airplane: the centripetal force makes it bend leftward but what you experience is actually an opposite, rightward, centrifugal force. Those two forces have the same magnitude but opposite direction. Anyway is only the centripetal force that keeps the airplane in a turn. The centrifugal force is the inertia "felt" by the pilot/passenger and acting on all the non-lifting surfaces of the airplane.

Answer 2

The forces in the first pictures would be as shown if you are observing situation from reference frame connected to the turning airplane. Then, in this reference frame, the airplane is not moving and therefore neither turning. Other forces, gravity and interaction with air molecules remains in place, so in order to make the description consistent, you need to add centrifugal force.

If you observe the situation from ground, there is no centrifugal force (because this fictitious/inertial force appears as a consequence of applying laws of motion in rotating reference system) and horizontal component of lift makes observed trajectory circular.

Edit2: The later two images are not complete. Forces has to be always in balance if viewed from airplane's reference frame. They are missing part of aerodynamic force generated by side-wise motion through air, which is definition of slip and skid (you can call it drag or airframe-lift). See @quietflyer's answer for more details on this.

Answer 3

The centrifugal force is produced by the act of turning. If you weren't turning, it would be zero. If it were zero, you would have an imbalance of forces which would cause an acceleration that would cause you to turn.

Properly, the centrifugal force is not really a force, it is an acceleration.

$a=V^2/r$

Recall that we can write $F=m\,a$ where forces balance out accelerations. So, the horizontal component of lift needs to balance out (or it causes) the acceleration in the turn.

As @Martin said, the second two images are bogus and the source that generated them doesn't understand what is going on.

Answer 4

Centrifugal force is a fictitious force, i.e., it is not real, it is added by engineers only to make the F=ma equation work in what is called a non-inertial or accelerated frame of reference. They can also be referred to as Inertial or Psuedo Forces. It is the same concept as trying to do F=ma for motion in a room on a rotating space station that has artificial gravity due to the rotation. In that frame of reference, the cup of coffee on the table is not accelerating, it is motionless. To make the F-ma equation work, (to make the force F and the acceleration be zero we need to include a fictitious Centrifugal force to oppose and balance the centripetal force. Other forces which fall into this category include Coriolis force and Gravity.

That is exactly what is going on for the diagrams you are looking at for an aircraft in a steady state turn.

What is not addressed, (and rarely explained in basic flight training), is that Newton's rules of motion (F=ma for example), are only valid in an inertial or non-accelerated frame of reference. Unless you are in free-fall (experiencing zero acceleration), you are in a non-inertial or accelerated frame of reference, and some fictitious force must be added to your analysis to compensate for the acceleration of the frame of reference in which you are doing your analysis.

In addition, it must be remembered that everything is relative. Not just velocity. Most of us will agree that all velocities must be measured relative to some reference velocity. How fast is the earth moving? well, there is no correct answer unless you also specify "with respect to what"? What is not generally understood is that the same concept also applies to acceleration. Sitting in your chair in your living room, we are in fact in an accelerating frame of refence (as we feel acceleration), upwards. That is why Einstein's famous "thought experiment" is so illuminating. In it he explains that there is no way that someone can tell the difference between that situation, and being in a room in a spaceship in deep space that is accelerating with a rocket engine thrust at 32 feet per second squared. These two situations are not just similar, they are equivalent, (identical). In both cases you are in a non-inertial, accelerating frame of reference, and a fictitious force must be added to any analysis to compensate for the intrinsic acceleration of the frame of reference to make any F=ma analysis equation balance properly.

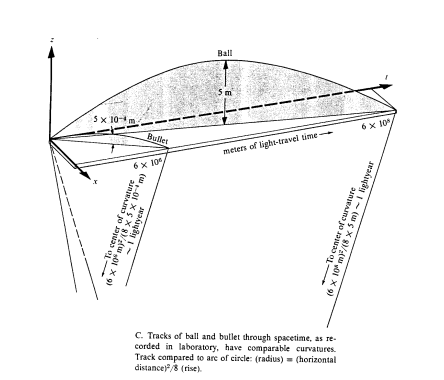
Heree is a graphic from Misner, Wheeler & Thorne's Gravitation that illustrates this:

Answer 5

Those diagrams, versions of which have been reproduced in many different flight training "ground school" materials, including some published by the FAA, are extremely misleading, and contain errors and omissions.

Slips and skids are not characterized by an "imbalance" between the pseudoforce called "centrifugal force", and the horizontal¹ component of the net aerodynamic force generated by the aircraft.

Rather, slips and skids can be said to be characterized by an imbalance between the pseudoforce called "centrifugal force", and the horizontal component of the wing's lift force. (This isn't the simplest or most intuitive definition of a slip or skid, but it is a valid one.)

Since the pseudoforce called "centrifugal force" is actually just a mirror image of the horizontal component of the net aerodynamic force generated by the aircraft, the above statement is really just an overly complicated way of saying that in a slip or skid, something other than the wing is generating an aerodynamic force which has a horizontal component.

That "something" is the fuselage. In a slip or skid, the airflow is striking the side of the fuselage, which generates a real aerodynamic sideforce, oriented perpendicular to the wing's lift vector. Because the fundamental defining characteristic of a slip or skid is that the nose of the aircraft is not aligned with the actual direction of travel through the airmass, in the yaw axis.

If the aerodynamic sideforce generated by the airflow striking the side of the fuselage were included in the diagrams attached to the question, the horizontal component of the net aerodynamic force generated by the aircraft would be exactly equal in magnitude (and opposite in direction) to the "centrifugal force" vector in every case.

If the vectors labelled "HCL" are supposed to represent the horizontal component of the net aerodynamic force generated by the aircraft, including the horizontal component of the aerodynamic sideforce generated by the airflow striking the side of the fuselage, then they are drawn incorrectly. For a given bank angle, the horizontal component of the net aerodynamic force generated by the aircraft is larger in a skidding turn than in a coordinated turn, and is smaller in a slipping turn than in a coordinated turn. If the vector labelled "total lift" is supposed to represent the total aerodynamic force generated by the aircraft, including the sideforce contribution from the airflow striking the side of the fuselage, then the coordinated turn is the only case where the "total lift" vector should be exactly "square" to the wingspan. In all cases the "centrifugal force" vector should be a mirror image of the vector representing the horizontal component of the net aerodynamic force generated by the aircraft.²

On the other hand, if the vectors labelled "HCL", "VCL", and "Total Lift" are only supposed to represent the horizontal, vertical, and total components of the wing's lift vector, then the diagrams become very confusing because the vector representing the aerodynamic sideforce generated by the airflow striking the side of the fuselage has been entirely omitted. In this case the vectors labelled "centrifugal force" should still appear as described in the above paragraph, but in the slipping and skidding cases, the vector labelled "HCL" will no longer be a mirror image of the vector labelled "centrifugal force". For a given bank angle, the wing's total lift vector, and therefore the horizontal and vertical components of the wing's lift vector, are all slightly smaller in a slipping turn than in a coordinated turn, because the aerodynamic sideforce generated by the airflow striking the side of the fuselage supports a small portion of the aircraft's weight.³ Similarly, in a skidding turn, the wing's total lift vector, and therefore the horizontal and vertical components of the wing's lift vector, are all slightly larger than in a coordinated turn, because the aerodynamic sideforce generated by the airflow striking the side of the fuselage contains an earthward component that must be opposed by the wing's lift vector. These differences should be apparent in the vectors labelled "VCL" and "Total Lift" as well as the vectors labelled "HCL", and the vector labelled "Total Lift" should be "square" to the wingspan in all three cases.

Obviously the diagrams would be greatly improved by changing to an airmass-based reference frame rather than an aircraft-based reference frame, so that the "centrifugal force" vector could be entirely discarded, and by also including by the aerodynamic sideforce vector generated by the air striking the side of the fuselage. There's no need to break things into horizontal and vertical components-- just show the wing's lift vector and the aerodynamic sideforce vector from the airflow striking the side of the fuselage. These two vectors are oriented perpendicular to each other. In a coordinated turn, there is no airflow striking the side of the fuselage, so the aerodynamic sideforce vector is zero, so the net aerodynamic force acts "straight up" in the aircraft's reference frame. In a slip or a skid, the aerodynamic sideforce vector is not zero, and so the net aerodynamic force does not act "straight up" in the aircraft's reference frame. End of story. (If desired, an "apparent load" vector could be added, which would always be the mirror image of the vector sum of the wing's lift vector and the aerodynamic sideforce vector from the airflow striking the side of the fuselage. Only in the case of the coordinated turn, where aerodynamic sideforce is zero, would the "apparent load" vector be exactly "square" to the wingspan. And now-- keeping in mind that the weight vector makes no contribution to the "apparent load" vector-- we understand why the inclinometer ball behaves as it does in slipping, skidding, and coordinated turns. And we've come to this understanding without ever invoking some sort of hypothetical "imbalance" between "centrifugal force" and some other force. It all boils down to the question of whether the airflow is, or is not, striking the side of the fuselage and generating an aerodynamic sideforce vector.)

This concept was also explored in this related, highly upvoted question-- What is missing from these diagrams of the forces in slips and skids?

But as to your specific question:

If in a coordinated turn, the horizontal lift vector is equal to the Centrifugal force. Then how is the aircraft still turning?

Because when we include the pseudoforce called "centrifugal force" in our vector diagrams, we are using an aircraft-based reference frame rather than an airmass-based reference frame or ground-based reference frame. Since the aircraft can't accelerate relative to itself, the net force in the aircraft-based reference frame will always be zero, whether the aircraft is turning or not. In the aircraft-based reference frame, the fact that the centrifugal force vector exists at all is actually evidence that the aircraft is turning. But for teaching purposes, using the aircraft-based reference frame (and therefore including the "centrifugal force" vector whenever the flight path is not linear) is arguably an inferior approach to using the airmass-based reference frame (and therefore not including any "centrifugal force" vector.)

Footnotes:

1. In this answer, when we talk about "horizontal", we mean as seen looking at the aircraft in a head-on view. We're not referring to fore-and-aft forces such as thrust and drag.

2. Note that in the diagrams attached to the question, the illustrator chose to make the "total load" vectors in the "skidding turn" and "coordinated turn" identical, and to make the "HCL" (horizontal component of lift) vectors in the "slipping turn" and "coordinated turn" identical. That's all kind of random and makes no sense.

3. For an extreme case, consider sustained linear "knife-edge" flight at a 90-degree bank angle-- here the aerodynamic sideforce from the airflow striking the side of the fuselage is doing all the work of supporting the aircraft's weight, and the wing's lift vector is zero.

Answer 6

I'm gonna go out on a limb and suggest that all of these answers seem to be answering a different question than "why does the airplane turn" when the bank is such that the acceleration in the airplane's rest frame points straight down (a coordinated turn). A ball on a string swung over your head moves in a circle without any aerodynamic forces. So there's something else going on.

Part of the problem is conflating turn = "move in a circle" with turn = yaw.

You can have a banked airplane where the wing lift vector points off to one side but the airplane doesn't turn. Every PPL candidate has to demonstrate a slip to landing, which is exactly that - the rudder input exactly opposes the yaw tendency created by the horizontal relative wind component.

What that highlights is that the yaw is caused by the side-force of the relative wind on the tail. The horizontal relative wind at the tail is caused by the horizontal lift component created by the bank. In a constant rate turn the rate of yaw must match the rate of turn, but that doesn't mean the airplane's nose has to be pointed along the tangent of the turning circle.

If the apparent "g" in the airplane frame is not straight down, that will cause a horizontal wind component on the tail which will yaw the plane. If the horizontal "g" points to the inside of the turn, the effective wind will yaw the nose to the inside of the turn & vice versa. That's why you can make a turn without using any rudder - the "g" to the inside will create enough yaw to keep the plane turning. But it's less aerodynamic than if you get all the yaw from having inside rudder input instead of depending on inside "g". So "coordinated turn" = "apparent g straight down".

Answer 7

Newton’s first law. If the forces are perfectly in balance then the aircraft will continue to turn at a constant rate.