From what I know the term bound vortex comes from potential flow theory and is a part of circulation around a wing.
I'll use the following diagram for illustration:
Intuitively, a hard surface resists fluid flow via shear stresses; these shear stresses tend to rotate fluid elements (i.e. vorticity). Therefore, we can regard a hard surface as filled with vortex sources.
In actual fact, only the boundary layer contains vorticity, and there is usually no fluid element rotation outside of it. Since the boundary layer does not affect lift [much] in attached flows at high Reynolds number, we can ignore it and call the entire flow irrotational. This leads to the concept of potential flow. Furthermore, we can model the hard surface as a vortex sheet. The vortex sheet is called singularities and does not affect the irrotational assumption because it is a boundary and not part of the flow itself.
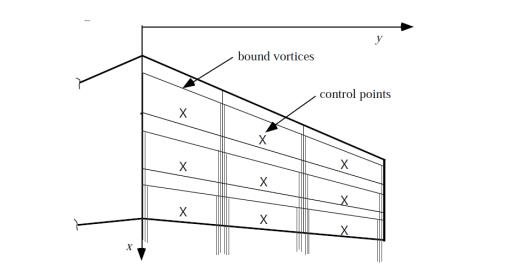
We can simplify the picture further. If the body is thin (like a wing), we can model the entire wing as a single vortex sheet (collapsing both upper and bottom surfaces into one). To simplify further, we can break the vortex sheet into individual vortex filaments running parallel to the wing (in the y-direction in the picture). These vortex filaments are called bound vortices. For high aspect ratio wings, we can simplify even further by considering the entire wing as a single filament, which leads to the Lifting Line Theory.
Since a vortex filament cannot end in a fluid, it must shed and end in the far field at infinity. This is a horseshoe vortex; the shed vortex filaments are called trailing vortices. A filament does not only shed at wingtips; in fact, it's shed everywhere along its length.
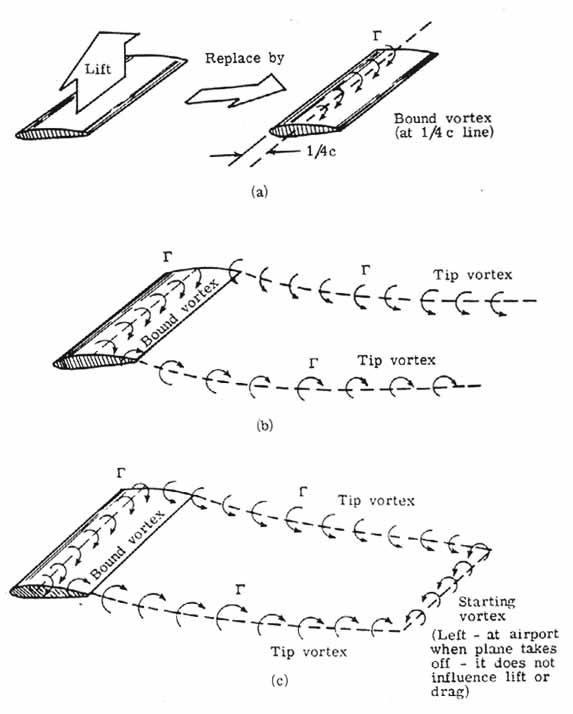
Image ref: https://history.nasa.gov/SP-367/f53.htm
Circulation is the total vorticity within a given closed loop. If you draw a closed loop from leading edge to trailing edge at a given span location, the total vorticity passing through this loop is the circulation at the given span location. Note that trailing vortices would be parallel to the said loop and will not partake in the circulation.
Lastly to complete the picture, acccording to Kutta-Joukowski Theorem, lift at unit span ($L'$) is directly proportional to the circulation ($\Gamma$) at the location:
$$L'=\rho_\infty V_\infty \Gamma$$
