Everywhere I look I'm only able to find information about airfoils.
How do I find out the location of the Aerodynamic centre of an arbitrary aircraft irrespective of its type or shape?
Everywhere I look I'm only able to find information about airfoils.
How do I find out the location of the Aerodynamic centre of an arbitrary aircraft irrespective of its type or shape?
What you refer to as the aerodynamic centre is also called the neutral point, the point where pitch moments do not change at all angles of attack with attached airflow.
If you ask for an unswept body of high aspect ratio, the answer would be easy: At the quarter chord point in subsonic flow and at the half chord point in supersonic flow. Unfortunately, the neutral point shifts forward with decreasing aspect ratio until it sits right at the leading point (not edge) of a slender body, a body with infinitesimally small aspect ratio. It shifts slightly backwards with positive sweep, so you normally have to calculate correction factors which depend on
If you don't have good wind tunnel data or a validated CFD model, you would use a collection of formulas and diagrams like DATCOM (see this link for a computerized version) to approximate a solution. Sorry, but I cannot give you a simple formula which would work out of the box.
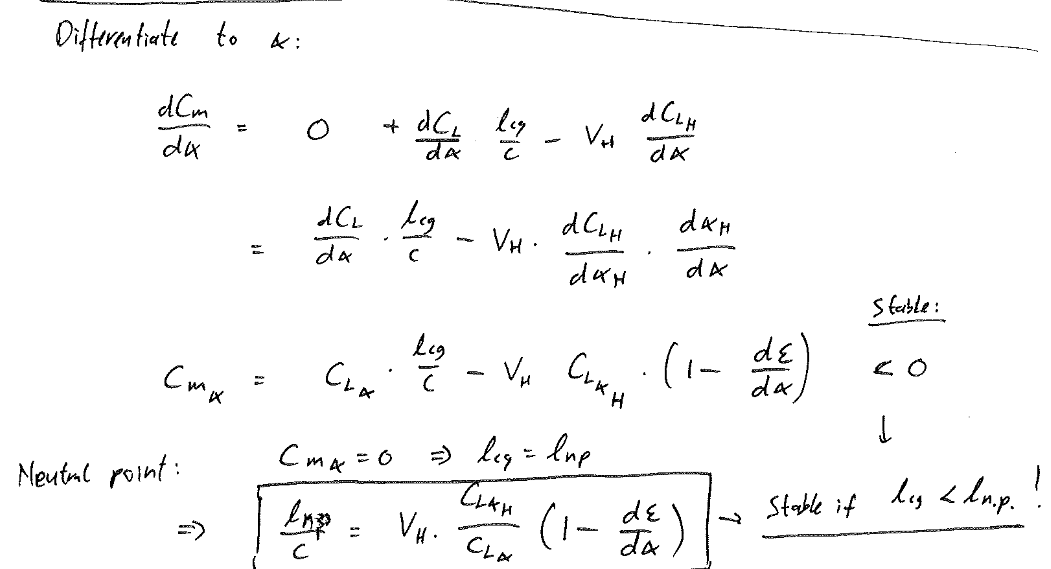
Can't you use the stability derivation?
Differentiate to $\alpha$
$$ \begin{eqnarray} \frac{\text{d}C_m}{\text{d}\alpha} &=& 0 + \frac{\text{d}C_L}{\text{d}\alpha} \frac{l_{cg}}{c} - V_H\frac{\text{d}C_{L_H} } {\text{d}\alpha} \\ & &\\ &=& \frac{\text{d}C_L}{\text{d}\alpha} \frac{l_{cg}}{c} - V_H \frac{\text{d}C_{L_H} } {\text{d}\alpha_H} \frac{\text{d}\alpha_H } {\text{d}\alpha} \end{eqnarray}$$
Resulting in:
$$ C_{m_\alpha} = C_{L_\alpha} \frac{l_{cg}}{c} - V_H C_{L_{H_\alpha}} \left( 1-\frac{\text{d}\epsilon}{\text{d}\alpha} \right)
$$