Are wings any more efficient at creating lift, versus orienting the engine's thrust downwards?

Question

As I understood from this article, wings on an airplane basically create lift by pushing air down.

Maintaining the lift force expends energy because air needs to be continually accelerated downwards. In the "energy budget" of the airplane, this energy cost appears in the form of extra drag due to the wing, which must be compensated by extra engine thrust.

It seems to me then that the power required to keep an airplane at a constant altitude using a wing is exactly equivalent to the power that would be needed if we would "simply" use a downwards-pointing fan instead of the wing.

Now, some posts on this website compare VTOL aircraft to airliners of similar size, and state that VTOL requires much more thrust (e.g. here - I would post more links but I'm not allowed to). But according to the reasoning above, shouldn't it require exactly the same amount of power to put a plane in the air, whether using a wing or directing the engine thrust downwards?

Please disregard, for a moment, the technical difficulties and safety issues. They are discussed in other questions related to gimbal thrust and VTOL aircraft. My question has to do with energy expenditure and power.

Answer 1

Interesting question. Purely empirically, it is the lift-to-drag ratio you are looking for. If you take this value as given for any particular aircraft, you have a direct answer for how much more effective wings are. It is the ratio of the lift to the total drag. The engine only needs to overcome the drag.

With L/D equal to unity you would need the same thrust as for the vertical take off. But even quite "bad" fixed wing aircraft would have L/D about 5. Gliders or similar aircraft built with a strong focus on aerodynamics can have an L/D of 50 or more (at least in some narrow range of airspeeds).

So yes, wings are more efficient. About one order of magnitude as a rule of thumb for common aircraft and optimal airspeed.

Why your reasoning with air pushed down is incorrect is more tricky to explain. I'll start with the assumption that, as air passes an airfoil, its speed relative to the airfoil is unaltered, and only the direction changes. (I know air slows down at least because of friction etc., but these are, at least theoretically, avoidable things not related directly to creating the lift. If there is something intrinsically related to lift which makes airflow change not only direction but speed too, then someone will hopefully correct me here.)

See the image. Airmass moving initially towards the airfoil with velocity $\vec{v_0}$ is deflected down by angle $\alpha$. Therefore the change in the velocity is $\vec{\Delta v}$. This change can be divided into horizontal and vertical components. To hold the aircraft in the air, the vertical component has to be equivalent to the aircraft's weight divided by the mass flow rate over the wing. The vertical component is related to the horizontal by $$ \Delta v_{\rm horiz}=\Delta v_{\rm vert} \cdot \tan{\alpha\over 2}. $$

So, from this simplistic view, the drag would be $\tan\alpha/2$ times the lift. Higher mass flow rate over the wing (longer wings, higher airspeed) makes it possible to keep the same lift with lower deflection ($\alpha$), thus less drag due to the generated lift.

---

Additional comment: how is it related to power and energy

The answer above is focused on how wings decrease necessary engine thrust, but original question could be interpreted in terms of energy efficiency too. I'll try to add some comments on this part.

• simple example – rocket engine: not too typical for aircraft, but simple one. Rocket consumes same amount of fuel per second in order to generate a unit of thurst regardless of its size and regardless if it is pointing upward (and is static with respect to air) or forward (and moving through the air). You need to burn proportionally more fuel per second to generate higher thrust. So, for the rocket propulsion, you will save fuel in the same ratio as the necessary thrust decreases.

• Propeller or jet engines are more complicate as their thrust and fuel consumption depends on engine movement through the air too. As David K pointed out in his answer, we can use momentum and kinetic energy of accelerated air to get power needed for unit of thrust.

  With some simplifications, thrust is mass flow rate through the engine/prop multiplied by change of flow speed it causes. $T = \dot m \cdot (v_{\rm out} - v_{\rm in}) = \dot m \Delta v$. Power needed for this is $P = \dot m \cdot {1\over2}(v_{\rm out}^2 - v_{\rm in}^2)=\dot m\Delta v\cdot(v_{\rm in} + {\Delta v\over 2})$. Thus $$ {P\over T}=v_{\rm in} + {\Delta v\over 2}\,. $$

  For stationary engine holding against gravity, higher thrust compared to flying fixed wing aircraft is needed as shown above. If we do not "cheat" by increasing mass flow rate through engine (like making it helicopter rotor or using multiple engines), $\Delta v$ has to be increased in order to achieve the necessary thrust. So you not only need more power because of increased thrust but also more power because of increased Watts per unit of thrust. Note that even "helicopter cheat" does not work too well. To match power consumption of engine generating less thrust thanks to the wing's L/D you need to improve P/T too – by decreasing $\Delta v$, thus increasing mass flow (rotor/propeller radius even more than proportionally to increased thrust).

  What about decrease of P/T due to the movement through air? Well, it depends on particular engine and its $\Delta v$. It will be typically in similar order of magnitude as airspeed (or even less), so we can not neglect $v_{\rm in}$ in watts-per-thrust equation above. There is a efficiency penalty when engine works on moving aircraft. But it should be still worth of it as the gain provided by lift is greater.

  A simplified example: We have an engine capable of producing enough thrust to lift aircraft vertically. It can be throttled by changing $\Delta v$ without any practical problems or change in its internal efficiency. And let's assume that mass flow rate through it is fixed area $S$ multiplied by air density and multiplied by arithmetical average of speeds of entering and exiting air. For hovering aircraft and stationary engine producing thrust equal to aircraft's weight, $w$ it is $$w=\dot m \Delta v_{\rm hover} = \rho S \Delta v_{\rm hover}^2 / 2\,;\quad \Delta v_{\rm hover}=\sqrt{2 w \over \rho S}$$ and thus $$P_{\rm hover}=w\cdot \Delta v_{\rm hover}/2=\sqrt{w^3\over 2\rho S}\,.$$

  The same aircraft flying on its wings does only need $w\over L/D$ of thrust. Airspeed is $v_{\rm air}$. Equation for the thrust: ${w\over L/D} = \dot m \Delta v_{\rm flight} = \rho S \cdot (v_{\rm air}+{\Delta v_{\rm flight} \over 2}) \cdot \Delta v_{\rm flight}$. Thus $$\Delta v_{\rm flight}=\sqrt{{2w\over(L/D)\rho S}+v_{\rm air}^2}-v_{\rm air}$$ and $$ P_{\rm flight}={w\over L/D}\cdot(\sqrt{{w\over 2(L/D)\rho S}+{v_{\rm air}^2\over 4}}+{v_{\rm air}\over 2})\,. $$

  Unfortunately, I do not see any way how to simplify and compare $P_{\rm hover}$ and $P_{\rm flight}$ so some concrete numbers:

  • Light aircraft, 1 ton, 100 knots, $S = 5\,\rm m^2$, $L/D = 15$: $P_{\rm hover} = 290\,\rm kW$, $P_{\rm flight} = 35\,\rm kW$.

  • Heavy aircraft, 100 ton, 200 knots, $S = 50\,\rm m^2$, $L/D = 15$: $P_{\rm hover} = 90\,\rm MW$, $P_{\rm flight} = 7\,\rm MW$.

  So, based on these simplifications flying with wings with similar kind of engine should be significantly more efficient in energy terms too. And, additionally, you are already moving forward using power $P_{\rm flight}$. For vertical engine extra power would be necessary for overcoming air drag due to the movement.

Answer 2

With regard to energy expenditure and power, for a given amount of force that is to be produced by accelerating an air mass, more power is required when you accelerate a small air mass in each period of time than when you accelerate a large air mass. This is because the force is proportional to the change in momentum of the air mass, whereas the power is proportional to the change in kinetic energy; and while momentum is $mv,$ kinetic energy is $\frac12 mv^2.$

The typical airplane engine grabs relatively small parcels of air and propels them backward at high velocity. A large propeller, or a high-bypass turbofan with a large intake, will do better than a small propeller or a turbojet with a small intake. But the wing of a typical conventional aircraft "grabs" a much larger parcel of air during any unit of time than its engines do. By pushing the wing forward through the air, the aircraft converts the relatively inefficient production of force by its engines (taking small parcels of air from in front of the aircraft and accelerating them rapidly backward) into the much more efficient production of force by its wings (taking large parcels of air above the aircraft and accelerating them relatively slowly downward).

Simply turning the typical engine (jet or propeller) of a conventional aircraft downward does not allow the aircraft to accelerate nearly as much air downward as the wing can when the aircraft is in normal flight.

In a helicopter (also known as a "rotary wing aircraft") the engine turns the wing (aka rotor), thereby pushing it through the air and accelerating air above the aircraft downward whether the fuselage is moving forward through the air mass or not. A helicopter can therefore take off vertically with a relatively small powerplant compared to what you would need to take off vertically with anything like a conventional fixed-wing aircraft engine. If you think of a helicopter rotor as a "downward-pointing fan," then it actually works reasonably well.

Answer 3

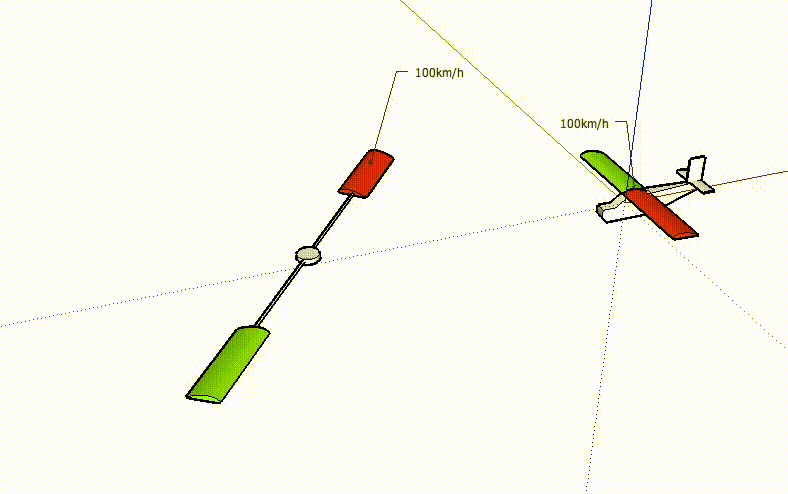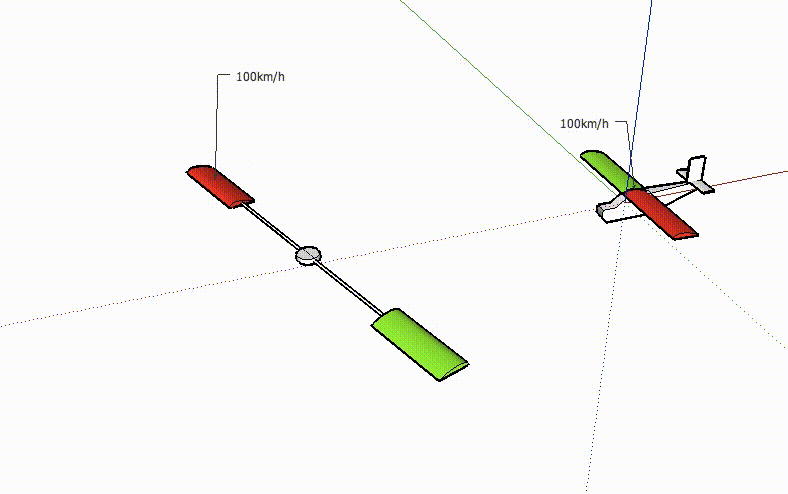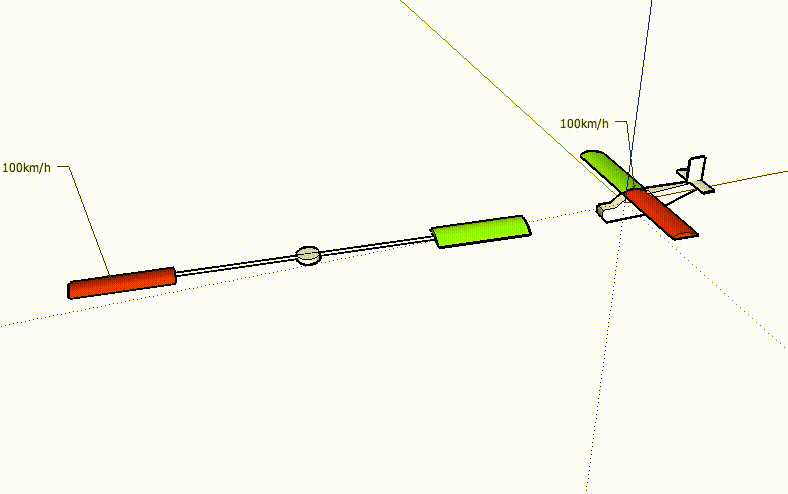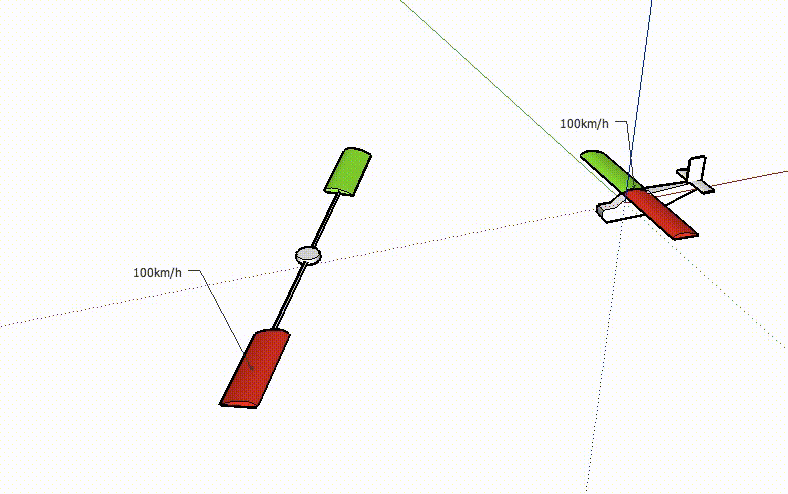
In a traditional aircraft the majority of the power from the engine is used to keep the aircraft moving forward at a certain speed. Very little of that power is actually needed to create lift.

Consider a simple paper airplane. It flies for a long time with no engine at all, until the drag on it causes it to slow down and if loses lift and descends to the floor.

In the hands of a skilled pilot, gliders can stay aloft for hours with no engine at all.

I'm not going to get into the argument about whether wings work by directing air downwards or not because it is simply irrelevant. The basic truth is, when a wing is oriented in a way that it provides lift when moving forward, all you need the engine to do is drive that wing, and the rest of the aircraft, forward at that speed.

The wing and the body of the aircraft create an effective drag as they are pulled, or pushed, forward and the engine needs only to create that amount of force so as not to slow down. That force is A LOT less than you need to lift it directly.

Most aircraft engines simply do no have the thrust force to lift the aircraft on their own. During early aircraft development many attempts were made to do so and failed because engines of sufficient strength simply were not available.

Wings had been around a long time before the Wright brothers came along, but flight was unpredictable and uncontrolled. The first true aircraft was invented because the brothers discovered and invented a mechanism to allow them to control the wing(s).

In short, it is far easier to provide lift with wings than by using thrust vectoring.

HOWEVER: At this point you are probably still scratching your head wondering how you can lift an aircraft without actually getting that amount of power from the engine.... So let me try to explain.

Let's say you have a car, and I tell you to lift it 6 feet... Well, unless you are this guy it's just not going to happen...

But what about if you do the following?

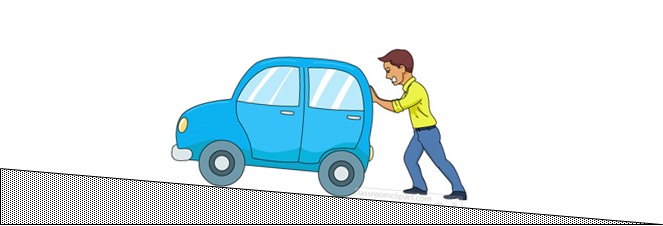
Well, you might complain and be out of breath, but you can see how, if the ramp were a long enough slope, you could use our muscles to get the car up to that height.

Because we are slow-moving creatures we think of air as nothing at all. However, air becomes a different thing when you try to move it out of the way very quickly. It becomes significantly "hard".

An aircraft in flight can therefore be thought of climbing an air ramp as shown below.

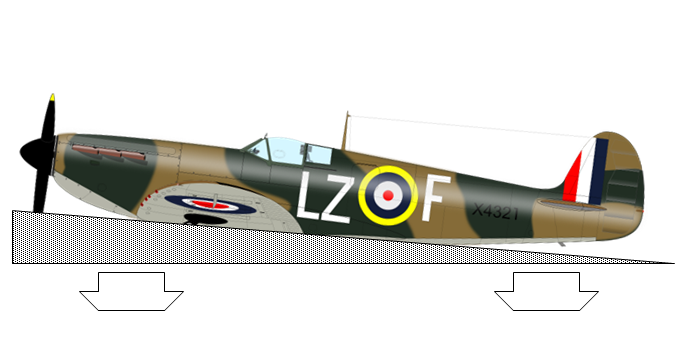
The aircraft and wings cut through the air reasonably easily, but the air under the wings, and body, acts like a ramp. The bigger the wings, the harder and more solid the ramp. This provides the lift.. keeping the aircraft up.

Of course the ramp is not solid, and effectively drops as we push the aircraft forward. In other words the aircraft is dropping and climbing at the same time. When in level flight the ramp is dropping at the same rate as the aircraft is climbing it.

What that means is the wings give you the mechanical advantage of using a ramp to reduce the force needed to perform work. Ignoring drag, the work required is the same as if you lifted it vertically, but since you spread the work over a long forward distance the effort required from the engine is divided significantly.

Efficiency:

Now is that more efficient? Well, traditionally ramps and other mechanical advantage devices are less efficient than a straight lift because there are losses involved in extra friction in the apparatus.

However, vertical propulsion based lift systems themselves are horrendously inefficient.

As we discussed about, air is harder to move the faster you try to move it. This means doubling the power of the engine does NOT translate into doubling the thrust, it is more of a exponential function. That is, you need to burn more than twice as much gas to get double the thrust.

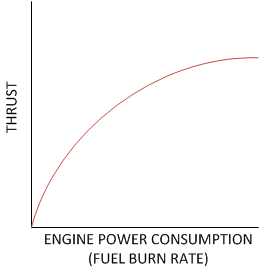
Worse, for any given engine, there is a limit to how much thrust it can produce. Eventually the air cavitates in front of it. It will, if it can turn fast enough, suck ALL of the air from the intake so fast that a vacuum forms. At that point the engine is starved of air and can go no faster no matter HOW MUCH fuel you pump in. That means, in order to get more thrust, you need a bigger engine, which means more weight, which means you need more thrust.... Do you see where I am going with this?

And remember, that is just to keep you up, you still have to use more power to go from point A to point B.

As such, even with the drag losses, winged flight still uses a lot less gas for any given journey distance.

Answer 4

Engines (let's say piston engines) do not provide lift. Engines drive wings. Each blade of a propeller is a wing. Each wing (at same size, airfoil, angle of attack, relative speed, altitude) provides the same amount of lift.

Both devices below provide the same lift, one flies straight forward, other flies in circle. One is a plane, other is a propeller. Pointing the thrust of an engine downwards = pointing the flight direction of the blades horizontal. Hope this helps.

Answer 5

If we ignore the losses, maintaining the aircraft at a given altitude requires no power, since no work is done on it. It does, however, require a force, and you seem to confuse force and power. The term efficiency has no meaning (at least no well-defined meaning) when talking about forces.

For example, I can hold a weight of 20 kg in my hand, and I could hold 200 kg using a 1:10 lever. Sure, you can say that the lever is 10 times more efficient, and in that sense wings are more efficient than vertical takeoff engines: you can take off with an engine which has 10 times less thrust. The corollary is that you will need 10x more time to reach a given altitude, just like I would lift a 200 kg weight 10 times slower using a lever than I would lift a 20 kg weight with my hands.

Answer 6

The way "basic" flight was always explained to me is that because of the shape of the wing, air over the top has further to go, thus is "stretched" the air under the wing has less distance to travel. So the air under the wing has more "pressure" then over the wing. At no time are you actually "pushing air down". At least not exactly. The weight of the craft does cause the air under the wing to "smoosh" (or displace) the same way a boat does, while there is no force (aside from the lift) pushing "up" on the wing.

This is all a very simple explanation. But the core part, the very very important part is that in no aircraft, fixed wing (plane) or rotary (helicopter) is ANY lift generated by pushing air down. Lift is generated by there being less air pressure "on top" of the wing then there is "under" the wing when combined with the downward force of gravity. It's the pull downward that actually makes planes go up, as odd as that sounds.

Now in your question you want to know why it takes less energy to fly "plane like" then "helicopter" like. Again, remember that pushing air down doesn't do squat until you get into rocket engines.

To answer that we take a look at what each engine is trying to move. In a small plane the engine has to move a propeller. Lets say about 70 lbs. With that engine turning 70lbs of weight it can "pull" (in much the same way as the wing) a small plane about 140 knots. That is more then enough "speed" to make the wingy bits of the plane generate lift. Keep in mind "lift" doesn't have to be this huge large force, it just has to be a tiny bit stronger then gravity.

In contrast, a helicopter's "blades" (there just wings that spin around) weight around 250 lbs. It's hard to convert the rotational speed to knots but at 650 feet/s that's roughly 385 knots (the math on that is very rough)

So, it takes far less energy to pull an aircraft forward at 140 knots. Then it does to spin a set of wings at 384 knots.

Keep in wind that the wings on a plane can be MUCH larger then the wings on a helicopter. That extra surface area creates more lift at slower speeds.

To make it more complicated, all of the "planes" energy is used to move the craft forward. That's it. A plane only goes one direction. They don't really turn as much as they "fall" in a prescribed direction (by generating less lift on one side or the other along 3 axis). The helicopter on the other hand has to spend some of it's energy to move "forward". It's "forward" motion is basically prescribed falling just like the plane, but then has to have energy spent on generating more lift, where as the plane just moves forward.

TL;DR I'ts not really apples to apples, but it takes less energy to generate the same lift by moving forward then it does to spin wings in a circle and generate lift that way.

HUGE NOTICE I used the speeds, and flight profiles from many aircraft. The plane I used was "a Cessna" but I took numbers where I could find them so some are the beloved 172 other are the other variants. The helicopter numbers are even more varied. I tried to keep it to lighter weight helicopters but I may not have succeed. The important part is that the theory is right, but don't try to count on the math in any real way.

Another note some VTOL craft do actually push air down, but this is even less efficient then spinning tiny wings. In short pushing down to go up is like a rocket, creating less pressure on top and "floating" up is a plane.

Answer 7

I just want to add something that I feel has generally been overlooked here. As the specific airspeed over the wing/propeller increases, the drag increases more than just linearly, its exponential. In other words, since the airflow(in mass) over a wing is a lot higher, it can produce x amount of lift at a low airspeed, while with an engine, since it has a lower airflow(again in mass) needs to move the air faster over the propellers in the engine to generate the same lift. As the drag is not linear, it needs considerably more power to overcome the drag from the engine, which is what causes the inefficiencies.

Answer 8

Skimming through the answers, I'm missing a very simple approach to explain the difference:
Listing the inefficiencies for both design solutions

Fixed wing

• non-lift-producing drag - Some of the drag is not related to producing lift, e.g. friction of air on wing surface.
• wing tip vortices - The pressure difference between above and below the wing seeks to equalize by a flow of air from below to above. This can be mitigated by winglets and/or high aspect ratio of the wing.
• anything - This is a placeholder for anything, I might've missed. See below for its counterpart.

Rotary wing

• non-lift-producing drag - Same as the equivalent point above, except for different wing profile, airfoil and varying airspeeds. See below for more on varying airspeeds.
• wing tip vortices - Same as the equivalent point above, except wing length and thus aspect ratio is more limited in design choice. Winglets would cause lots of structural problems and increase drag over-proportionally, because they are by definition on the (fast moving) tip.
• anything - Basically anything that applies to fixed wings does apply for rotary wings, too. Plus, often times the non-uniform airflow (see below) does not allow for optimizations in wing profile, airfoil, etc.
• non-uniform distribution of air speeds over the blade - The tips of a rotor are moving faster through air than its base. Thus optimal airspeed is hard to obtain everywhere on the rotor blade at once.
• different airspeeds for preceding vs. receding blade - Forward speed of the aircraft is added to the air speed over the preceding blade but subtracted from the receding. This difference adds to the problem of achieving optimal airflow.
• need for counter torque - The tail rotor in the classic helicopter design requires power from the main engine without adding to lift or forward thrust. It's basically a "useless necessity". Twin rotor designs may suffer from increasingly "disturbed airflow" (see below).
• circular motion - Circular motion is basically accelerating towards the center. "Flying straight" would be more efficient, e.g. there are bearings on the rotor that leak the angular momentum. In comparison a fixed wing doesn't leak its momentum besides the other inefficiencies. This also poses structural requirements on the rotor blades that may limit other design optimizations.
• disturbed airflow - The previously receding blade moves through wake of the previously preceding in the next revolution. The disturbed air doesn't create as clean an airflow as it would undisturbed. This reduces lift to drag ratio.
• unoptimized airfoil - Multiple points above already mention this, either by making it necessary (structural requirements in circular motion) or by preventing optimization (non-uniform distribution of air speeds over the blade).

To come back to the original question:
As a rule of thumb, we can assume that the longer the list of inefficiencies the less efficient the design. Especially when everything (and anything) on the one list appears on the other, too. There would need to be big qualitative differences in each point for the rule of thumb to be violated.

Answer 9

A major advantage a wing has versus an engine is that in typical use it will constantly get to encounter relatively undisturbed air. A downward-pointed engine would create an area of low pressure above it, and air flowing into it will be moving downward even before the plane gets to do anything with it. The only way the plane can generate thrust will be to accelerate the already-moving air to an even higher speed. The amount of energy required to accelerate a cubic meter of air from 9m/s to 10m/s is almost twice as great as the amount required to accelerate 10 cupic meters of air from 0m/s to 1m/s, but the amount of lift generated by the latter will be ten times as great.

Answer 10

The article makes a misleading generalization, as it was one of the first things I learned during flight training. Airplanes don't fly by "throwing air down", they work by generating reduced air pressure that lifts the airplane up (hence, "lift") and forward (thrust). Both wings and propellers (and turbines) are airfoils whose upper curved surface accelerates air as the airfoil moves through it, thereby reducing air pressure. The relatively decreased air pressure above the wings and relatively increased air pressure below the wings and behind the propeller floats the airplane up and forward.

A modicum of truth is that the air flowing over the wing is deflected downward, and some air is compressed beneath the wing as the airplane moves, but is a much smaller component of what keeps the airplane flying.

Lower air pressure behind the wing produces drag and is a byproduct of lift. Surface friction of the lifting surfaces and body of the airplane are also components of drag. A vortex generated at the wingtip as the higher- and lower-pressure airflows converge and spiral around each other can also be a strong component of drag, as well as causing turbulence that can affect other aircraft.

To address another part of your question, producing lift and thrust follow the same principles using conventional piston and jet engines (rocket engines produce thrust by expanding gases). Perhaps one of the best visible examples is the Osprey tilt-rotor aircraft with large propellers that can produce lift, thrust, and any combination in between, depending on the angle of the engines.

@ymb1's analogy about pushing a box was an excellent choice. Moving perpendicular to gravity (i.e. moving wing forward) takes less force than simply opposing it (i.e. thrusting downward). So, wings are a more efficient choice, from both a structural and complexity perspective.

Answer 11

Let's go back in time to ask the pioneers attempting human powered flight. The wing design offered efficiency over designs that relied on vertical thrust. Such efficiency encouraged further research.

The lift force developed by a wing at proper airspeed relies on pressure. Pressure is also responsible for the lift (buoyancy) force in boats (water pressure instead of air). You can ask a submarine designer about abandoning ballasts and adding downward propellers to maintain depth.

Although a different mechanism, a hot air balloon can demonstrate that different amounts of energy is needed to produce the same lift using a different engineering principle. A small jet engine can actually be used to generate enough hot air to lift the balloon with carriage. However, pointing that small engine downward will not provide enough thrust for equivalent lift.

Answer 12

Isn't this a bit like comparing apples and oranges? Given no outside forces, wings without an engine to drive them do very little to get you into the air.

An engine can create 'lift' by pointing it downwards. To get off the ground the engine would have to generate thrust to counteract its weight. If you add wings, you can get into the air with far less thrust. So wings INCREASE the efficiency of an engine when it comes to how much thrust is required to become airborne.

Answer 13

Addng to @Dmitry Gregoriev excellent answer: it could be that your question boils down to: why is a fixed wing more efficient than a disk shaped wing.

Because of the lifting line theory. Creating a given amount of lift over a finite span is the more efficient the larger the span.

Answer 14

Regarding the article:

You say that your understanding is that the article says that wings on an airplane basically create lift by "pushing" air down.

This statement is incorrect to the extent that this implies that lift is created only by the bottom of the wing. (I am not 100% sure that is what the article is saying since it merely states that "Planes fly by throwing air down.")

The theory that lift is generated only by the bottom of the wing is referred to in the NASA webpage described in earlier comments as the "Skipping Stone" theory. The webpage states that this theory is incorrect because it does not recognize that lift is also created by the top of the wing.

Another NASA webpage explains that "Lift is a force generated by turning a moving fluid" and that "Both windward [bottom] and leeward [top] parts deflect a flow." (words in square brackets added)

Let me add some other evidence and observations:

The book "Stick and Rudder" written by Wolfgang Langewiesche in 1944 has sometimes been cited in support of the "Skipping Stone" theory because the book talks about "slamming" the air downward. However, in his explanation, the author states that:

"The main fact of all heavier-than-air flight is this: the wing keeps the airplane up by pushing the air down. .... It shoves the air down with its bottom surface, and it pulls the air down with its top surface; the latter action is the more important." [p. 9]

Another observation that highlights the importance of lift created by the top surface is the fact that a stall involves the loss of lift produced only by the top surface. The bottom of the wing continues to produce lift, as before. But it does not provide enough lift alone to keep the airplane aloft.

The importance of lift generated by the upper surface is also familiar to any pilot who has flown into icing conditions. As noted in "Aircraft Icing" AOPA Safety Advisor, Weather No. 1, p. 2:

"Wind tunnel and flight tests have shown that frost, snow, and ice accumulations (on the leading edge or upper surface of the wing) no thicker or rougher than a piece of coarse sandpaper can reduce lift by 30 percent and increase drag up to 40 percent. Larger accretions can reduce lift even more and can increase drag by 80 percent or more."

I also recall having read a couple of anecdotal accounts of what happens when there is interference to the air flowing across the upper wing, but can't provide a cite. One involved Martin Caiden who set a record for number of skydivers jumping at one time when 19 skydivers were hanging along the top of the wing of his Ju 52 "Iron Annie".

I do know that business jet manufacturers used to have to add strakes on the top of their airplane wings to insure that the air along the top surface flowed as originally intended. The engineers at Cessna were quite proud when they were able to design a wing (the Citation X) which did not require any strakes.

Answer 15

Wings are an economical way to accelerate a mass of air downwards. The fact that natural selection has chosen that system over other alternatives possibly means that wings are the most economical solution...