The effect you noticed is the result of the combination of Doppler effect and sound absorption.
Sound absorption in a fluid is proportional to
$exp(- \alpha \nu^2 x)$
where $\alpha$ is a dimensional constant, $x$ is the traveled path, and $\nu$ is the sound frequency.
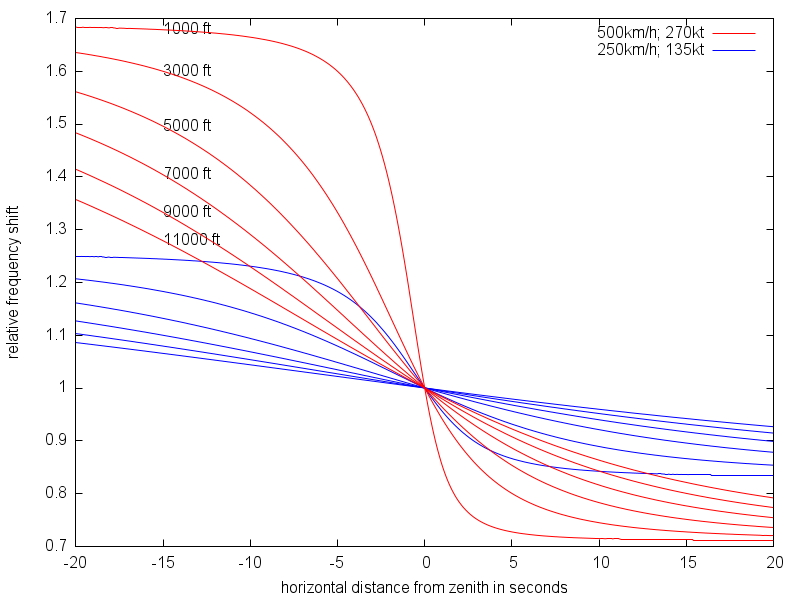
In its own reference frame, the airplane emits always the same sound, irrespective of whether it is approaching or receding from the observer. But for the observer, the frequency of a wave (which is $\nu_0$ in the plane frame) seems $\nu_a = \frac{\nu_0}{(1-v_j/c_s)}$ when the airplane is approaching, and $\nu_r = \frac{\nu_0}{(1+v_j/c_s)}$ when the plane is receding (here $c_s$ is the sound speed and $v_j$ the airplane's speed). Thus the perceived frequency jumps by a factor
$F = \left(\frac{1+v_j/c_s}{1-v_j/c_s} \right)$
This means that the absorption coefficient, at the time when the airplane is passing overhead (thus $x \approx H$, the plane's height over the ground), jumps from
$D_a = \exp \left(\frac{-\alpha H \nu_0^2 }{(1-v_j/c_s)^2}\right)$
to
$D_r = \exp\left(\frac{-\alpha H \nu_0^2 }{(1+v_j/c_s)^2}\right)$
For typical values for the atmosphere, and the propagation of sound waves in it, this means that $D_a \ll 1$, while $D_r \approx 1$.
Hence, what is happening is that, as the airplane passes roughly overhead, the sound goes from being deeply absorbed, especially at high frequencies, to not being absorbed at all (its normal sound, as you call it). The strange sound you noticed is what you perceive as the lower frequencies are becoming less and less absorbed, and thus the sound spectrum changes.
EDIT 1:
after I wrote my reply, DClayton (in a comment above) gave a very important hint that this is exactly what is happening when he noticed that the same effect appears with gliders, hence it is unrelated to engine sounds (I ignored this, thanks).
EDIT 2:
it is also worthwhile remarking what happens for high-altitude planes, when observed from the ground: in that case, the traveled distance $x$ is sufficiently large that all frequencies audible to human ears are absorbed, so that the plane goes from being nearly silent when it is approaching to being very clearly noticeable as it is receding.
You may try this on a clear day with high humidity at high altitudes, when over-flying jets leave white wakes: you will be unable to hear them as they approach (even though you can see them thanks to the presence of the white wake), but you can easily hear them as they go away, but just keep in mind that all of this is delayed by the (substantial) time it takes for sound to reach the ground, which, for 33,0000 feet or more, is of order 30 seconds. In other words, you will hear the noise level grow perceptibly about 30 seconds after the jet has passed the point closest to the observer, i.e. 30 seconds after flying directly overhead.