How can the glide ratio in a balanced turn be estimated?

Question

I am trying to estimate the bank angle/turn radius required to lose a given amount of altitude during a full orbit. This has led me to trying to estimate glide ratio in proportion to $L/D_{MAX}$ of an aircraft in a balanced gliding turn, knowing only $V_{MD}$ and $L/D_{1g}$ for $V_{MD}$.

My approach is to use the following relationships:

$D = D_0 + D_I$

$D_{0} = C_{D_0} 0.5{\rho}v^2 S$

$D_I = C_{D_I} 0.5{\rho}v^2 S$

$C_{D_I} {\alpha} {C_L}^2 $

$C_L {\alpha} L $

$L {\alpha} n $

$n = \frac{1}{cos({\phi})}$ (${\phi}$ = bank angle)

Assuming $D_0 = D_I$ at $V_{MD}$ I think I should be able to calculate:

$L/D_{n_g} = \frac{L/D_{1g}}{0.5 + 0.5(1/cos^2({\phi})}$

Since altitude lost in a full orbit becomes $\frac{2{\pi}r}{L/D}$ where r = turn radius and

$r = \frac{v^2}{g tan(\phi)}$

Altitude lost in a full orbit should ${\alpha} \frac{1}{cos^2({\phi}) tan({\phi})}$ which looks like this. Does my method look alright, or have I made any incorrect assumptions or errors?

Answer 1

I get a variation of the glide ratio with $\frac{2}{\bigl(\cos\varphi + \frac{1}{\cos\varphi}\bigr)}$ for circles flown with a bank angle of $\varphi$ when compared to straight flight at the respective best speed for optimum L/D.

Your initial assumptions are right:

Lift: $L = c_L\,\rho\,\frac{v^2}{2}\, S\, n_z$

Load factor: $n_z = \frac{1}{\cos\varphi}$ with the bank angle $\varphi$

Drag: $D = D_0 + D_i = \Bigl(c_{D0} + \frac{c_L^2}{\pi\, AR\,\varepsilon}\Bigr)\,\rho\,\frac{v^2}{2}\, S$ (zero-lift drag + induced drag), and both are equal at optimum $L/D$:

$$\Bigl(\frac{L}{D}\Bigr)_{\rm opt} = \Bigl(\frac{c_L}{c_D}\Bigr)_{\rm opt} = \frac{\pi\, AR\,\epsilon}{2\, c_L}\text{ for straight flight at }1\, g \text{ ($n_z = 1$)}.$$

Now with load factor $n_z = n$ and unchanged speed:

$$\frac{L_{ng}}{D_{ng}} = \frac{\frac{c_{L_{1g}}}{\cos\varphi}}{\frac{c_{L_{1g}}^2\bigl(1 + \frac{1}{\cos^2\varphi}\bigr)}{\pi\, AR\,\epsilon}} = \frac{\pi\, AR\,\varepsilon}{c_{L_{1g}} \bigl(\cos\varphi + \frac{1}{\cos\varphi}\bigr)}.$$

The ratio between both glide ratios is $\frac{2}{\bigl(\cos\varphi + \frac{1}{\cos\varphi}\bigr)}$, which makes sense because it will become 1 for $\varphi = 0$ and become less than 1 for nonzero values of $\varphi$. Note that the glide ratio at n g doesn't carry an "opt" subscript anymore, because by increasing $c_L$ we move away from the optimum. If the best L/D would be maintained for circling flight, speed would need to increase, not lift coefficient.

The altitude loss in straight flight of a distance equal to the diameter of a circle with the radius $R = \frac{v^2}{g\tan\varphi}$ is $2\,\pi\, R \, \frac{D}{L}$. When flown at the correct bank angle, this altitude loss per circle $\Delta h$ will become $$\Delta h = \frac{\pi\, v^2}{g \tan\varphi} \Bigl(\frac{D}{L}\Bigr)_{1g}\Bigl(\cos\varphi + \frac{1}{\cos\varphi}\Bigr).$$

Plot of the glide ratio with bank angle divided by the glide ratio for straight flight (blue line). I also added the altitude loss for a full circle (red line, Basis: $L/D = 18$ at 100$\,$m/s in straight flight), both for flight at constant speed and constant lift coefficient:

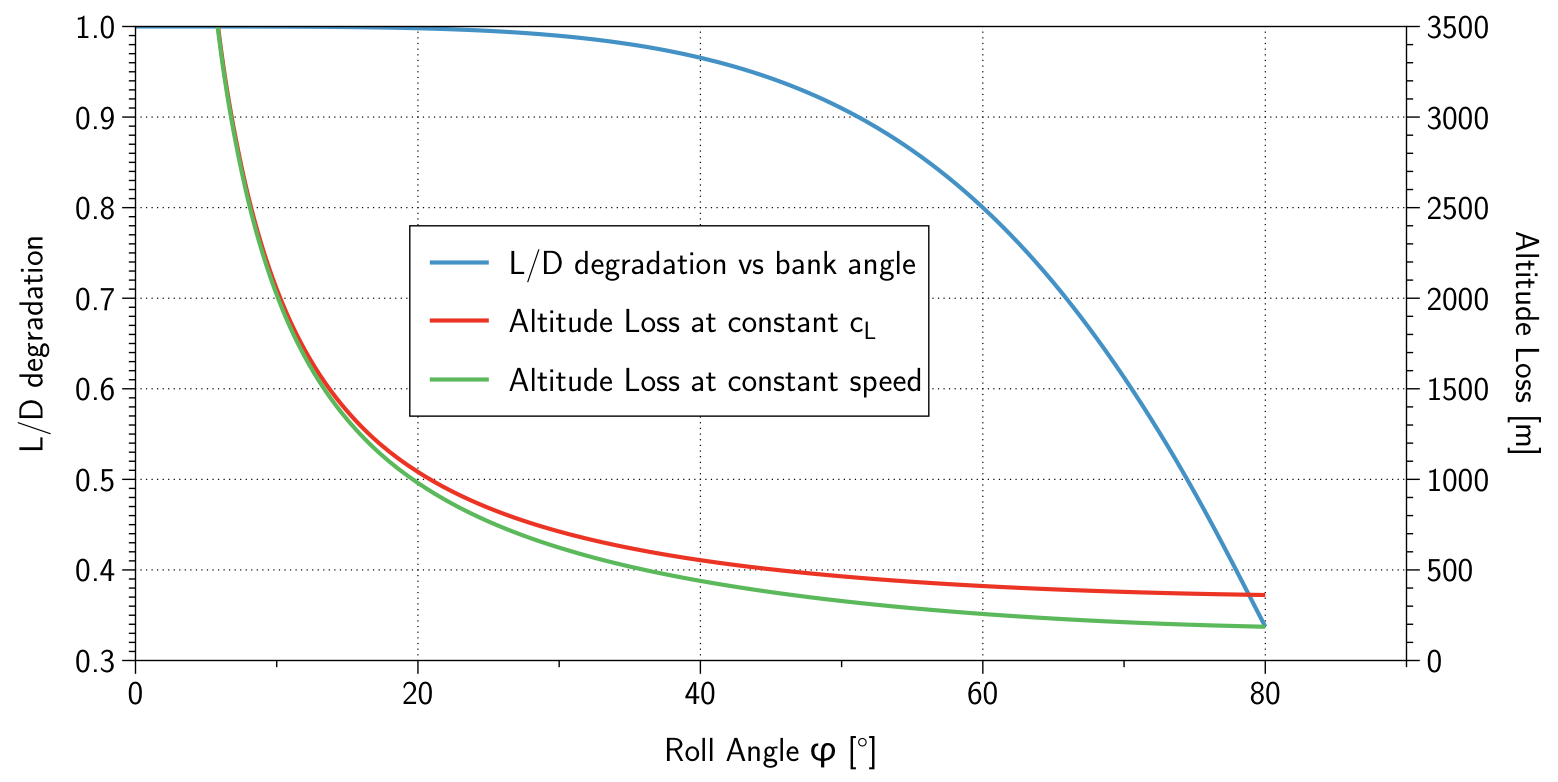
Use the results from this plot with caution at higher bank angles: Lift would need to double at a bank angle of 60°, doubling $c_L$, so we fly much more slowly than any pilot would. The red line gives more realistic values, since here the aircraft speeds up for flying higher bank angles. Flying at constant speed gives less altitude loss simply because the circle becomes smaller than when flying at constant $c_L$. Note that for every meter flown the altitude lost is greater when flying at constant speed, indicated by the blue glide ratio degradation curve.

Two factors conspire to increase aerodynamic losses when flying at constant speed with higher bank angles:

1. You need to create more lift, which causes higher induced drag, and
2. Your optimum $L/D$ is at a higher speed when the bank angle increases, which again increases aerodynamic losses because you move away from the optimum.

If you try to express the altitude loss per circle $\Delta h$ as a function of $\varphi$, you will get a quadratic equation and two solutions, one high and one low bank angle: $$\frac{\cos^2\varphi + 1}{\sin\varphi} = \frac{g\,\Delta h}{\pi\, v^2} \Bigl(\frac{L}{D}\Bigr)_{1g}.$$ Maybe MathSE can solve this algebraically; I would resort to iteration or tabulation here.