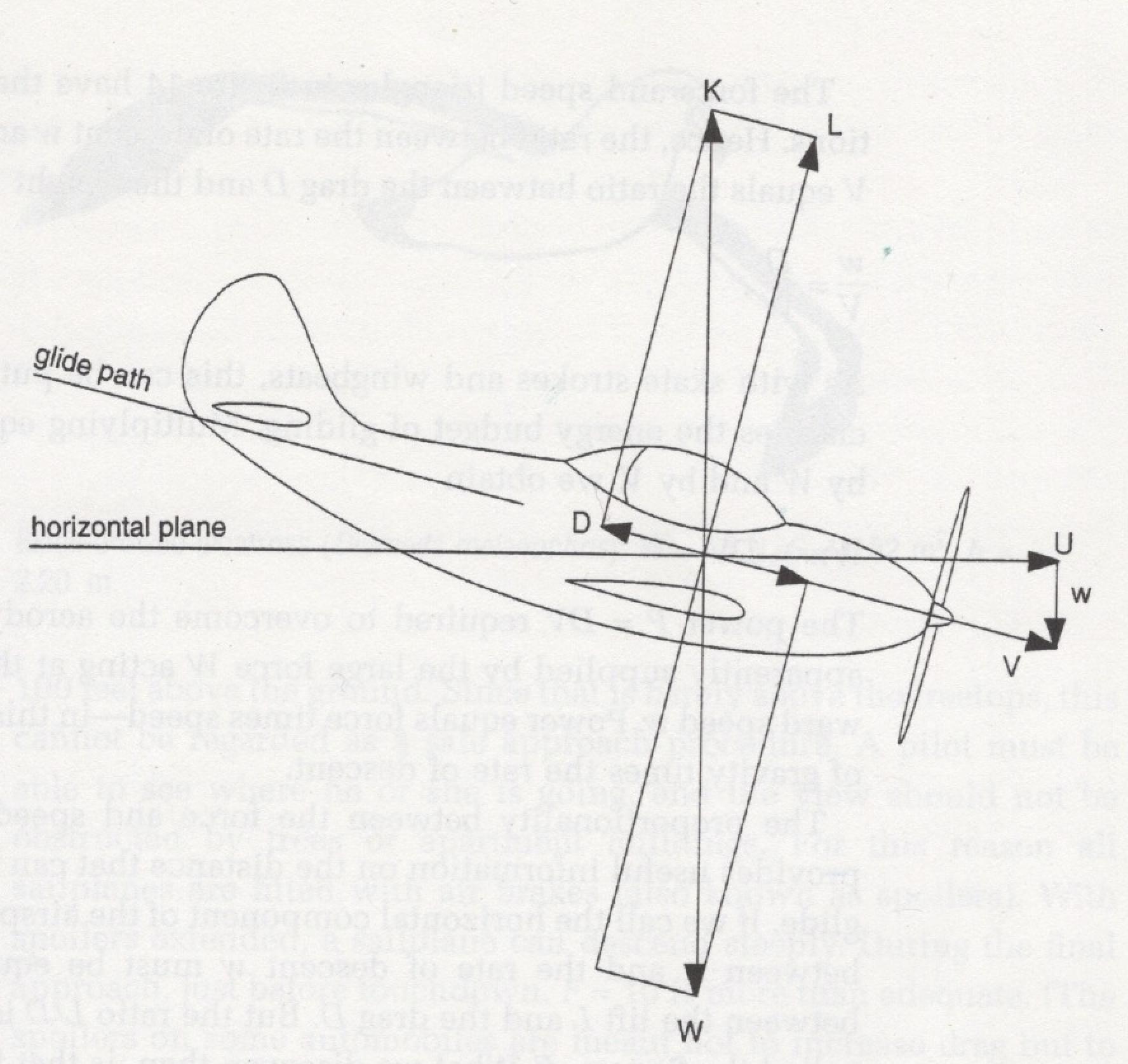
Lower altitude means higher air density, which means more liff, which means a glider can maintain a given glide slope at lower airspeed.
As a rule, aircraft tend to have higher peak L/Ds at low airspeeds, due to a reduced parasitic drag coefficient.
So, for a given design, I would expect the best glide airspeed to increase with altitude, and best L/D to decrease, and best glide slope to steepen.
Yes, altitude does affect glide ratio, but only slightly.
With increasing altitude, air temperature drops and with it the Reynolds number of the flow. This is mitigated, but not completely offset, by the increase in true air speed, such that the Reynolds number of an aircraft in the tropopause is less than half of the Reynolds number at sea level.
Since the Reynolds number is the ratio of inertial to viscous forces, a lower value signifies more viscous effects and, hence, drag for the same lift.
Altitude does not affect the glide ratio, since air density cancels out in the ratio L/D.
L/D is numerically the same as glide ratio (in conditions of zero wind). In this figure of a gliding plane, taken from 'The simple science of flight', by Henk Tennekes, it's clear that the triangle of forces L-D and the triangle of velocities U-w are similar. Hence, the ratios L/D and U/w are the same...
The L/D ratio will be the same at any altitude as the Lift and drag are related to the dynamic pressure experienced by the aircraft, which is a function of Indicated Airspeed, however, the True airspeed will be higher at higher altitudes. This means that a glider descending from 10000 feet to 9000 feet will cover the same distance over the ground as if it descended from 2000 ft to 1000ft, but it would take less time as its ground speed would be higher. This would give the impression that the glider had a higher performance at high altitudes.
