Would building a large asphalt square or painted dark black area create a "thermal generator" for glider use?

Question

Imagine if you build a "parking lot" square of asphalt and paint it the deepest black possible to absorb maximum heat...would that spot essentially be a thermal generator that you could fly over with a glider and reasonably expect good thermals? Assume it's summer and no other obvious obstructions to solar radiation on the day.

Even on a day when there's a relatively low altitude temperature inversion, could this make the air so hot that it would take a good vertical distance for the thermal generator's "parcel" of air to cool to the ambient temperature (and still make a flyable thermal above the normal inversion altitude)? Or, do thermodynamics not really allow you to get very far with this...assume usual 5.4 degrees Fahrenheit of cooling per thousand feet.

Answer 1

Yes, if it was large enough you could expect decent thermals from it, but not all the time. I've flown over mall parking lots where there is maybe 20 acres of asphalt, expecting a boomer, but no joy. Thermal sources can be frustratingly unreliable.

On the other hand, at my glider club there is a nearby solar panel farm, with panels covering probably 30 acres. It is actually a fairly reliable thermal source, seemingly better than a parking lot and I often head over to it when I get low and need to stay near the airport; I can usually expect at least some weak lift from it.

As far as paving goes, you get nearly as good thermals from dry dirt fields, so spending a million or two to pave 20 acres seems like quite a waste of money, especially when you really want to have thermal sources distributed all through the area you want to soar in. Lots of farm fields with low moisture content and minimal vegetation cover will provide plenty of lift on a sunny day.

If there is an inversion capping the convection layer, it seems to inhibit all the thermal sources, and you won't find a "breakthrough" thermal coming from a parking lot. Even smoke columns from large fires, much stronger than a typical thermal, tend to stop and spread out when they hit an inversion.

Answer 2

I made a short LES simulation

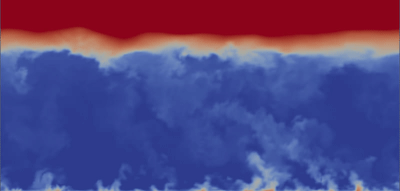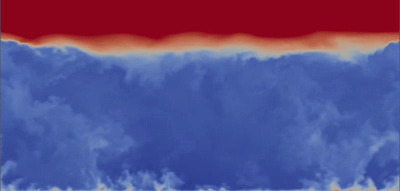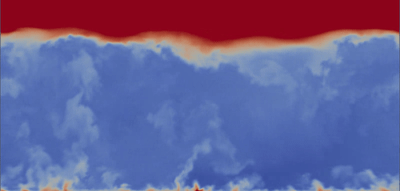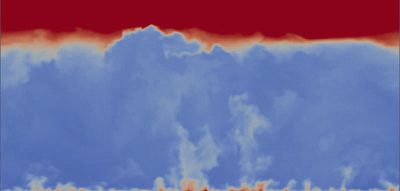
The color is thhe potential temperature and the scale span is 1.8 K between blue and red. There is a hot patch 100 m x 100 m in the middle. There is 0.1 K.m/s (~110 W/m^2) sensible heat flux elsewhere but 0.3 K.m/s (~330 W/m^2) in the that square. The wind speed is very low, only 1 m/s (~2 knots), still any significant long-term stationary plume is hardly visible. The BL height is about 1 km (900 m at the start, but the gif starts later). The case is completely dry for simplicity. With 30 fps it is 2 minutes of real time per every second of the animation.

Even after 1 hour averaging, the increased temperature is only visible quite localized close to the ground and the vertical velocity field is just determined by those large convective cells, any rising current from the patch cannot be identified in the 1-hour-averaged vertical velocity field at all. Note that the closest point is 5 m from the ground so we do not see the extremely hot layer very close to the surface, but it is properly parametrized.

The 100 m x 100 m ("football field") patch was simply too small to have a significant effect. It will help you in the surface layer, and may get you from the winch altitude to some bigger thermals, but it certainly won't get you to the inversion. Or it will be good for soaring birds.

Those sensible heat fluxes I applied are not low but rather typical. Remember that normally a large part of the total heat flux is taken by the latent heat flux.

---

Firstly, please note that surface heterogeneity is not necessary for thermal convection. Convection happens because of the surface heat flux and thermodynamic instabilities, not because of heterogeneities. Heterogeneities may introduce secondary circulation and various currents.

According to the simplemost particle method, an air particle will raise roughly to the height where its temperature will equal the temperature of the surrounding atmosphere. Your 5.4 K per 1000 ft corresponds roughly to the dry adiabatic lapse rate, that means that the potential temperature is constant. That happens in the mixed part of the convective boundary layer and after the capping inversion with some step rise it will then increase with some gradient. If you heat your thermal say 1 K above the mixed layer potential temperature, then it will rise until it equalizes with the surroundings. If your pot. t. gradient above the mixed layer is 1 K / 100 m, the inversion is weak, it may rise roughly another 100 m. 1 K is already a lot. It won't get you kilometers above the capping inversion. Only large convective clouds can do that.

Even regular thermals do enter the stably stratified layer above the capping inversion and cause entrainment. This is the entrainment layer.

The real thermodynamic temperature is less convenient because it decreases in the mixed layer (9.8 K per 1 km) and then may either increase or decrease above the capping inversion (end still be stably stratified, the sign is not that important).

Answer 3

Yes. Sunlight is about 1000 W/m². Absorbing it is a good way to get some heat.

We can improve on your design a bit. A good portion of sunlight is visible to the eye, but some of it is not. Not all "black" materials will perform equally. Pick a material that absorbs the invisible infrared and ultraviolet, too. Choose a material that is thermally insulating (like asphalt or most soils) to increase the amount of heat that goes into the air.

If you heat the ground everywhere then you have no control over the location of your thermal. Surround your dark area, which should probably be a circle rather than a square, with a white area. This will help your thermal stay put when the wind is light.

Your dark area will be most effective (per unit area) when the sun is directly perpendicular to the surface, which only occurs in the tropics (for horizontal surfaces).

Reference: https://www.nrel.gov/grid/solar-resource/spectra-am1.5.html https://en.wikipedia.org/wiki/Black_body

Answer 4

It would take more than a giant flat horizontal square.

This is not based on any numerical proofs but from my observations of weather in my area. I decided to add this answer after reading the discussion in the comments of Robert DiGiovanni and Vladimir F on Robert's answer about moisture and convection. To get a good strong thermal you are better off finding rather than building, but you may be able to enhance an existing location.

What you want is a large water area upwind probably 1-2 miles from steep (over 1:1 slope) hill and then place your thermal enhancement on the slope of the hill someplace below and not immediately before the crest. This should enhance the land breeze and the thermal energy will be concentrated in the area just at the crest.

If you download Vladimir F's graphic and look at it in a tool that stops the loop, you can see that at frame 200 and again at frame 300 convective plumes are generated that track downwind from about the 1/3 point to the 2/3 point . Individually they do not have enough energy to push though the boundary layer, although the one at 300 does make a strong attempt. I wonder if this simulation was allowed to run out to frame 2000 or more if there would be a greater convective progression, but I don't know the time equivalent scale of the frames to be sure.

The key is elevation change to impart an upward trend to the moist air mass and the additional thermal energy from a dense heat moment to accelerate moist air up through the boundary layer.

This is how clouds form downwind of Lake Erie in the summer. At the lake edge there are wind and solar farms, inland about 20 miles there are more wind farms on the taller hills. Convective cloud formation happens about 5-10 miles inland after the maximum 200NM fetch across the relatively shallow lake which late in the season can see water temperatures in the mid 70's.

Also if you are in the Northern Hemisphere, generally, a Low Pressure system north and east of your location will increase airflow into a western facing slope, as will a High Pressure system south and West of it. These are reversed in the Southern Hemisphere.

Answer 5

If you added a thin layer of water over it, or some type of steam generator, you might have something there.

H2O has a molecular weight of only 18, whereas air, predominantly N2 (28) and O2 (32) is much heavier. Steam has even been considered as a lifting gas!

It is humidity indeed that powers the strongest of thermals, found in thunderstorms, the builders of hurricanes. The ability of moist air to hold heat a bit longer allows it to continue rising in dryer air.

clue: "moisture content...has a strong moderating and energy storage effect, and the thermals will be weaker, but present later in the day".

So, now we see how to really "unstick" a thermal, by bringing in cooler, dryer air from around the "generator" and allowing it to rise with moisture added. Water vapor content has the added benefit of being strongly absorbent of solar radiation in the IR range, literally acting as airborne "asphalt" to sustain the rising column. Your design may then look like a giant bulls-eye, surrounded by a low heat absorbing surface.

A "circulation" of rising and sinking air is also helpful to create a sustained, strong local thermal, so right next to your square do create another one that will cool and dehumidify the air, making it as dense as possible.

Analysis of a plume from a propane burner (which has water vapor from combustion) vs an electric hotplate (dry heat) can be used to measure strength and height of thermals produced, which may lead to better modeling before full-scale production of a thermal square begins. If you succeed in forming a cumulus cloud, you may be in business (just don't get carried away).