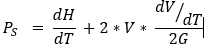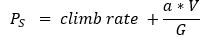What is maximum acceleration that can be achieved by afterburner in level flight?

Question

I am wondering how a fighter jet (let's take F-22 for example) would compare to other vehicles in terms of acceleration while in flight.

For cars and motorcycles, achieving 0-100 KPH in 2 seconds is doable and this means their highest acceleration would be around 14 m/s^2.

I know fight jets do not beat cars and motorcycles in the first 2 seconds of drag races and so while on ground, they do not achieve this level of acceleration. But how about a flying with an afterburner?

Is there any available data on the time required to accelerate a fighter jet from say mach 0.6 to mach 0.8 using afterburner? Can this be calculated anyhow?

Answer 1

The F-22 has a published static thrust of 156 kN per engine with afterburner, or 312 kN total thrust. At gross weight (29,410 kg), according to Newton's second law (F = ma), it would accelerate from a standstill at 10.6 m/s² or just over 1 g. (This calculation ignores static and dynamic friction from the landing gear, but those forces are quite negligible in comparison to 312 kN of engine thrust.)

A jet aircraft will not accelerate faster from a higher speed than from a standstill. Jets have a thrust curve that looks like the dotted line below (source):

As flight speed increases, drag increases, but thrust doesn't (much - certainly not enough to offset drag). Therefore acceleration goes down.

Answer 2

To answer your third paragraph, the aircraft with the highest thrust to weight ratios have figures of just over 1, so acceleration would be around 11m/s^2 under ideal conditions (i.e. if air resistance were negligible. However, if one were to loop a fast jet and hit full power in a vertical dive with low initial airspeed then it might be possible to achieve 20m/s^2 briefly. But then if you drive a lawnmower over a cliff it will achieve nearly 10m/s^2 so it’s hardly a fair comparison.

Answer 3

The attached chart illustrates the "Specific Excess Power" available to an F-16 at 15,000 feet MSL. The bold curves that start on the left at sea level, go upwards and to the right, peak at approximately 0.9 Mach, and then decrease and eventually terminate back at sea level are Ps curves. Ps stands for specific excess power, meaning Power per pound of aircraft. they are labeled with their respective values in units of Ft/Sec (speed) and is equivalent to V * (T-D) / W.

A formula for Specific Excess Power is:

This is equivalent to:

These specific excess power values represent the maximum combination of climb rate and/or energy gain due to acceleration, that the aircraft can achieve at the specified point in the flight envelope. Where they are negative, it represents the minimum rate of descent, and/or deceleration (from energy loss)that the aircraft will experience.

If you are in level flight, then climb rate is zero and the acceleration can be solved by
a = Ps * G / V

Looking at the diagram, it is clear that the highest Ps, and therefore the highest climb rate and maximum possible energy gain acceleration, at any altitude, occurs at or above 0.9 Mach.

This is because subsonic, as airspeed increases, jet engine power (Thrust * Velocity), increases much more significantly than total drag does, due to increased mass flow through the engine.

Energy gain, however, as pointed out by @TypeIA, is not the same thing as acceleration. This answer is therefore, not on point to the OPs question. I will leave this here anyway as, corrected, it is informative.

However, as the Ps chart is one of altitude vs TAS (Velocity), the actual acceleration (~ Ps/V) can be estimated by the slope of the Ps contours. Where they are angled at more than 45 degrees from the horizontal, acceleration is increasing as velocity increases.

Answer 4

Acceleration from a standing start is based on Force and Mass until the object gains enough velocity for drag to become a considerable factor.

One must consider the increase in drag as well as the mass accelerating from Mach 0.6 to 0.8. There is also the Mach effect with additional drag at high subsonic speeds.

The Raptors twin Pratt&Whitney F119 engines produce 2 x 26,000 lbs of thrust each dry and 2 x 35,000 lbs of thrust in afterburner. The afterburners add an additional 18,000 lbs of thrust force. For 60,000 lb weight, afterburners accelerate the aircraft:

18000 lbf × 4.45 N/lbf = 80068 N = 80068 kg m/s$^2$

80068 N = 27000 kg x acceleration

acceleration from afterburners = 3.0 m/s$^2$

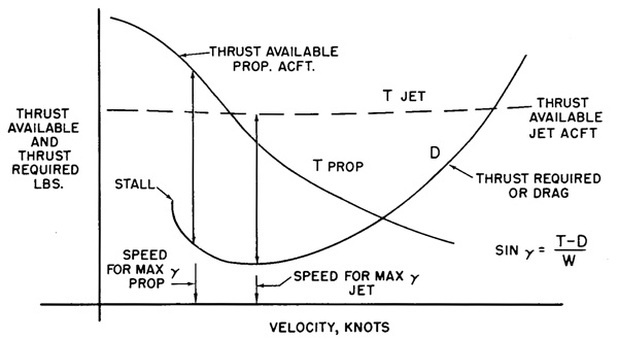
If you had some idea of the thrust required to go Mach 0.6 and Mach 0.8 at a constant speed, then you subtract the drag penalty from the known thrust value with afterburner. The excess thrust is available for acceleration.

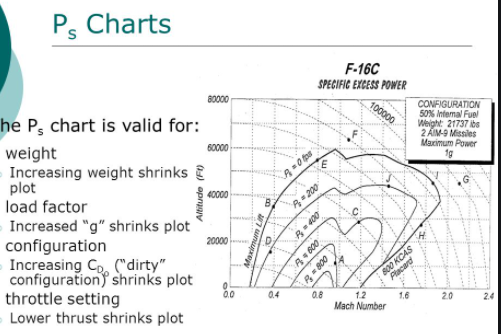
The main parameters for optimal performance are minimum weight, minimum drag and maximum thrust.

From a standing start, the motorcycle (and the cheetah) will be ahead of the F-22, but not for long.

The F-22's 70,000 pounds of thrust will continue to accelerate its 60,000 lbs of mass as the cheetah, then the motorcycle reach their top speeds.

But off the line, the cheetah and motorcycle acceleration is greater than that of the F-22. It should be noted that the best acceleration for the jet will also be at lower speeds, where there is less drag, but will never approach that of a cheetah's best.

Fast twitch muscle acceleration rates come from boxer data:

Here a 25 mph throw (11 meters/second) covers a distance of around 0.7 meter. The average velocity is 5.5 m/s. The distance is covered in: 1 s/5.5m × 0.7m = 0.13 seconds

The initial acceleration is 11m/0.13s$^2$ = 85 m/s$^2$

Top speed of 30 m/s in around 1.5 seconds yielding an acceleration of 20 m/s$^2$ (cheetah has least mass but also lowest thrust. Drag factors rapidly slow acceleration).

Time to cover 100 m:

d acc = 1/2 × 30 m/1.5 s$^2$ x 1.5$^2$ = 22.5 m

d rem = 100 m - 22.5 m = 77.5 m t rem = 77.5 m / 30 m/s = 2.6 s

Total cheetah time 100m = 1.5 s + 2.6 s = 4.1 s (hungry)

The motorcycle is 14 m/s$^2$ 0 to 30 m/s in around 2 seconds Top speed around 60 m/s

d acc = 1/2 × 14 m/s$^2$ × 2$^2$ = 28 m

d rem = 100 m - 28 m = 72 m

The motorcycle (using gears) continues to accelerate by (estimated) 5 m/s$^2$, covering 30.5 m in second 3, and 35.5 m in second 4

After 4 seconds it's distance traveled is:

d trav = 28 m + 30.5 m + 35.5 m = 94 m

After 4.1 seconds:

94 m + (38 m/s × 0.1 sec) = 97.8 m (very close!)

Special note to Hyabusa fans:

400 m (1/4 mile) ≈ 1/2 × a × 10 s$^2$

Acceleration = 8 m/s$^2$

This racing motorbike can develop 180+ horsepower by revving to very high rpm before going through its gears. It's observed acceleration is remarkably constant. Estimated time to 100 m:

100m = 1/2 × 8 m/s$^2$ × t$^2$

t = around 5 seconds (we're in the ballpark!)

The F-22 acceleration rate from a standing start with afterburners would be:

70000 lbf × 4.45 N/lbf = 311500 N

311500 N / 27000 kg = 11.5 m/s$^2$

Because drag only marginally affects its acceleration at lower speeds and huge N thrust, it is constantly accelerating at 11.5 m/s$^2$. After 4.1 seconds:

d trav = 1/2 × 11.5 m/s$^2$ x 4.1$^2$ = 97 m !

From this we can see the cheetah and motorcycle acceleration will be greater than the F-22 initially, with the rapidly decreasing acceleration of the ground competitors after a few seconds making an actual 100 meter race a very entertaining match up. The Navy figured all this out years ago, which is why they have catapults on their aircraft carriers!