As written above, why does using carburetor heat increase fuel consumption? Because I found such information in a few SOPs for piston airplanes and I do not understand why does it happen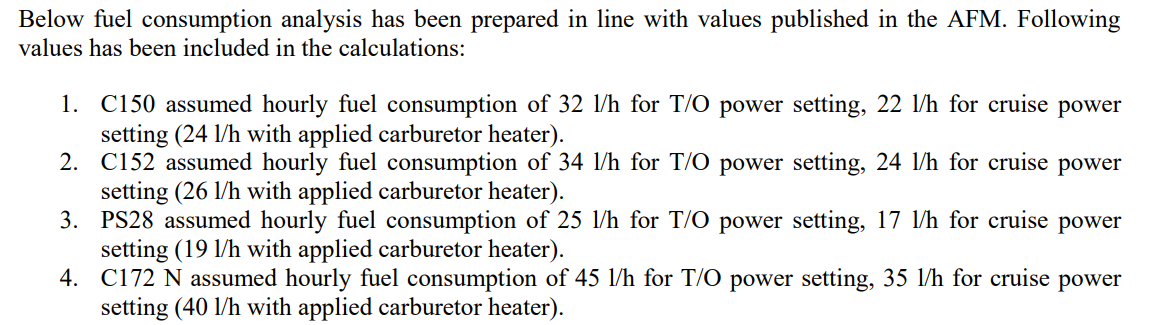
$\begingroup$
$\endgroup$
6
-
4$\begingroup$ Why not include one of the SOPs in your question? $\endgroup$– Robert DiGiovanniCommented May 2, 2021 at 11:14
-
$\begingroup$ Those are in polish so hardly anybody will understand. There are just tables with fuel consumption with and without carb heat and those with carb heat have higher values. Maybe those tables are wrong, I don't know, I hope somebody here knows ;) $\endgroup$– KonradCommented May 2, 2021 at 11:31
-
$\begingroup$ Using carb heat means hotter, less dense air is sent to the carburetor (to clear ice). This would change the fuel/air mixture. It would depend where you were on the "lean to rich curve". There is a possibility, if the engine ran too hot, the fuel mixture might have to be made more rich, affecting miles per gallon efficiency. What type of engine are we talking about here? $\endgroup$– Robert DiGiovanniCommented May 2, 2021 at 11:56
-
$\begingroup$ It is a Rotax 912 $\endgroup$– KonradCommented May 2, 2021 at 12:16
-
$\begingroup$ It's worth remarking that in practical terms carb heat can actually reduce fuel consumption, if it results in the fuel/air mixture being better distributed across the engine cylinders. This is something which Mike Busch has demonstrated when finding techniques to run engines lean-of-peak. The heat helps vaporize the fuel, ensuring that the liquid fuel doesn't favor one intake pipe over another. $\endgroup$– Kenn SebestaCommented May 3, 2021 at 21:33
|
Show 1 more comment
2 Answers
$\begingroup$
$\endgroup$
3
I can think of two reasons:
- The efficiency of heat machines depends on the difference between lowest and highest temperature in the cycle, relative to the highest temperature, as formulated first by Sadi Carnot. Simply put, the efficiency cannot be larger than $$\eta_{max}≤\frac{T_{max}-T_{min}}{T_{max}}$$ where all temperatures are expressed relative to absolute zero. If the entry temperature $T_{min}$ is raised by carburetor heat, the value of $\eta$ will drop. For the nitpickers: Piston engines are best described by the Otto cycle which uses an isochoric addition of heat and is a bit more messy to describe than the ideal Carnot cycle. If you want to include Diesel engines, use the Trinkler or Seiliger cycle.
- With the higher entry temperature, all temperatures in the cycle are higher and you need to lean less in order not to exceed the desired cylinder head and exhaust gas temperatures. More fuel is needed for cooling, so the richer mixture will drive up fuel consumption.
-
$\begingroup$ I have a funny thought concerning the cycle: why can't we include the carburetor heat as part of the cycle, and keep T_amb = T_min? Ultimately, part of the heat that we put into the carburetor will be even extracted during the expansion (at engine efficiency), wouldn't it? And if we used exhaust heat for the carburetor (which would just be wasted otherwise), wouldn't we even increase the efficiency? $\endgroup$ Commented May 3, 2021 at 20:59
-
1$\begingroup$ @CarlBerger A funny thought indeed. But you want to first compress, then add heat. If you look at the T-s-diagram of the Otto cycle, you want to maximize the enclosed area relative to the area between x-Axis and the diagram plus the enclosed area. Initial heat will only shift the enclosed area up and increase exhaust heat, which is wasted. Or take the the p-v-diagram: Initial heating will shift it right (lower density) while pressures stay the same. Shifting the process right reduces the enclosed area and, therefore, the work that can be extracted. Better to add this heat after compression … $\endgroup$ Commented May 3, 2021 at 21:27
-
$\begingroup$ yes obviously.. I guess i shouldn't write comments that late at night. $\endgroup$ Commented May 4, 2021 at 5:14
$\begingroup$
$\endgroup$
5
Heating the air causes the air to be less dense.
We all know that as you climb, the air becomes less dense and you have to lean the mixture for better performance and fuel economy. This also applies to Carb Heat. If you don't lean the mixture after applying Carb heat, the mixture will be richer and fuel economy will be reduced.
-
$\begingroup$ That’s the point that I do not understand. Ofc hotter air is less dense, so with the same amount of fuel the mixture will be overally richer. But on Rotax 912 there isn’t any manual mixture adjustment lever/knob, so why does the fuel consumption increase since we are not able to do any mixture adjustments $\endgroup$– KonradCommented May 3, 2021 at 12:45
-
5$\begingroup$ @Konrad If you do nothing but heat the intake air, the fuel consumption does not change, but the engine power drops. If you were flying trimmed and level, then turned on carb heat but did nothing else, you would start to lose altitude because the engine would no longer be producing as much power as it was before (the now rich mixture being unable to burn all the fuel it could just moments ago). If you don't want to fall out of the sky, you will increase the throttle - burning more fuel than before now to hold altitude. $\endgroup$– J...Commented May 3, 2021 at 12:58
-
$\begingroup$ If there’s no manual mixture adjustment then the reduction in fuel viscosity may increase the flow rate, this may be more significant than the reduction in fuel density $\endgroup$– FrogCommented May 3, 2021 at 19:31
-
1$\begingroup$ @J... - That, in itself, is an answer. You should make it one instead of just a comment. $\endgroup$– Dean F.Commented May 3, 2021 at 19:57
-
1$\begingroup$ I don't know the detail of the Rotax, but if the pilot doesn't have an adjustment knob in the cockpit, it doesn't necessarily mean that the value isn't adjusted. For unchanged mixture though, the reduced air mass flow means less power- and if you have to drive the engine to a higher powered operating point, that might be less economic. I guess we have too many technical possibilities for different engines that make a single general answer quite difficult.. $\endgroup$ Commented May 3, 2021 at 21:09
