Why does the temperature rise in the boundary layer after a shock wave?

Question

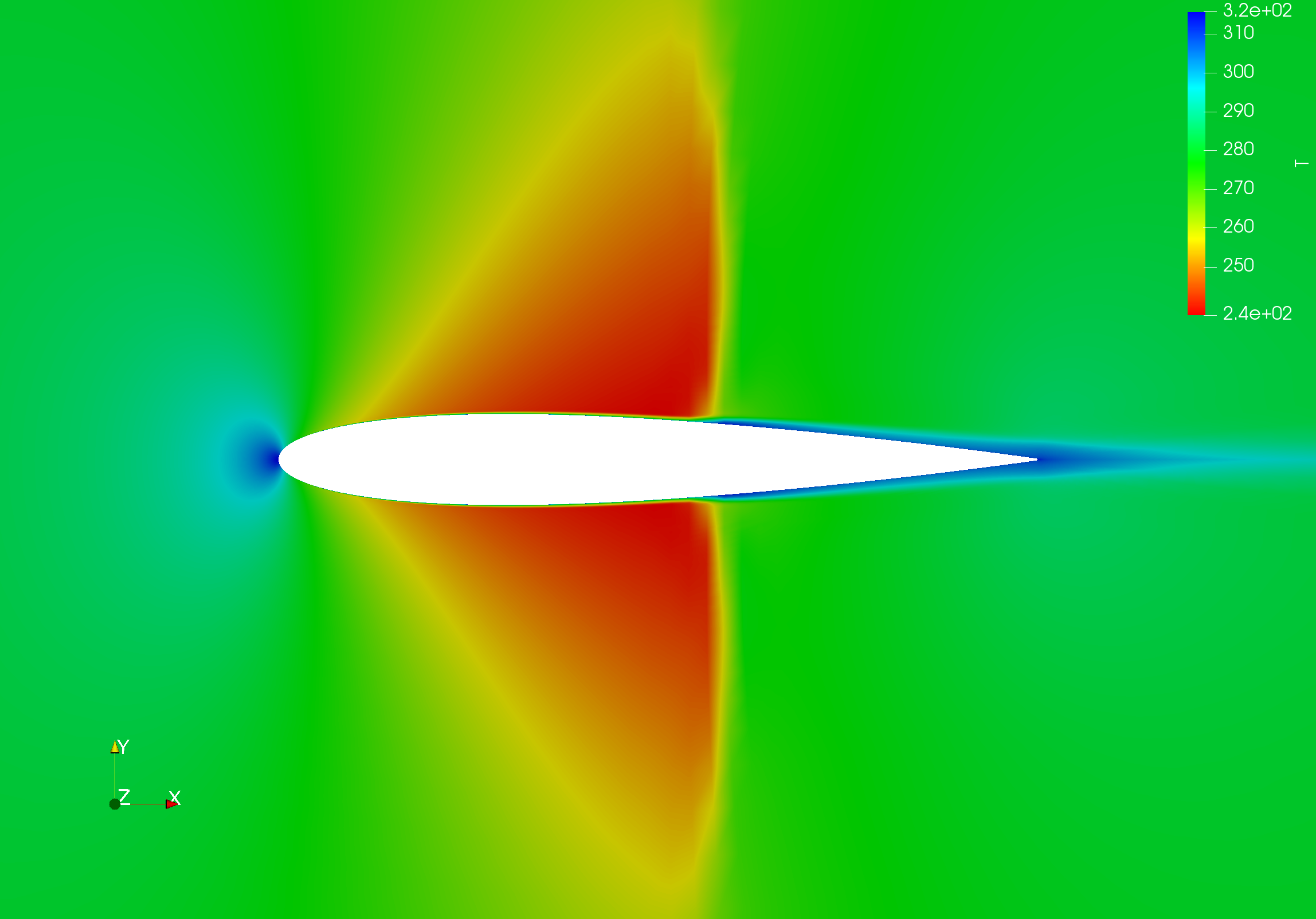
Is it because the BL thickens after the shock so the drag increases, causing a increased friction? Here M=0.82 far from the airfoil, the picture represents the static temperature in Kelvins around the symetrical airfoil at angle of attack 0°.

This is the total temperature for a supposedly everywhere adiabatic flow.

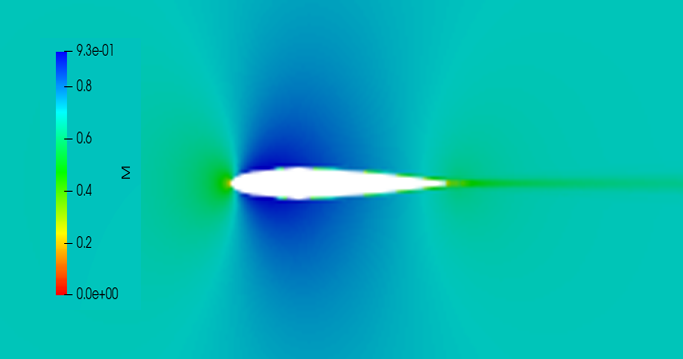
This is not the static temperature but the Mach number, for $M_{\infty}=0.70$

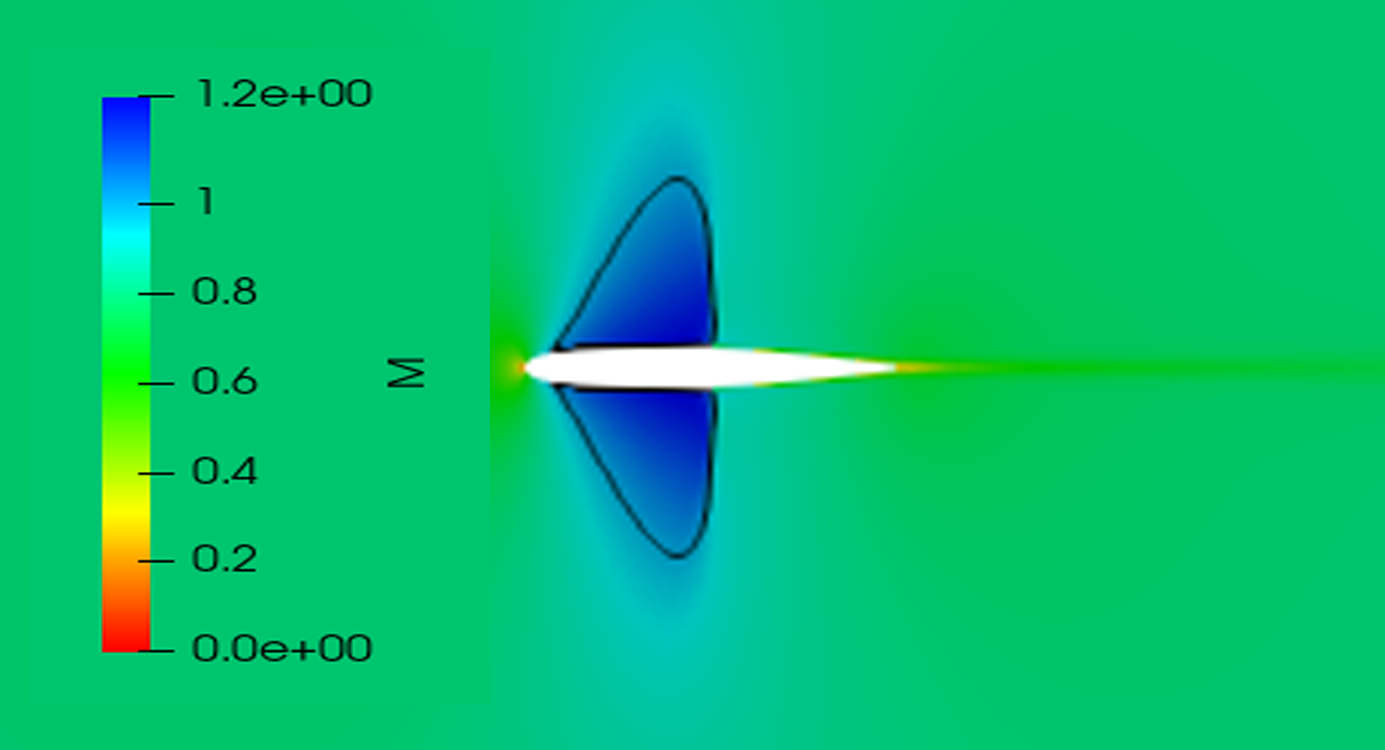Mach number for $M_{\infty}=0.80$

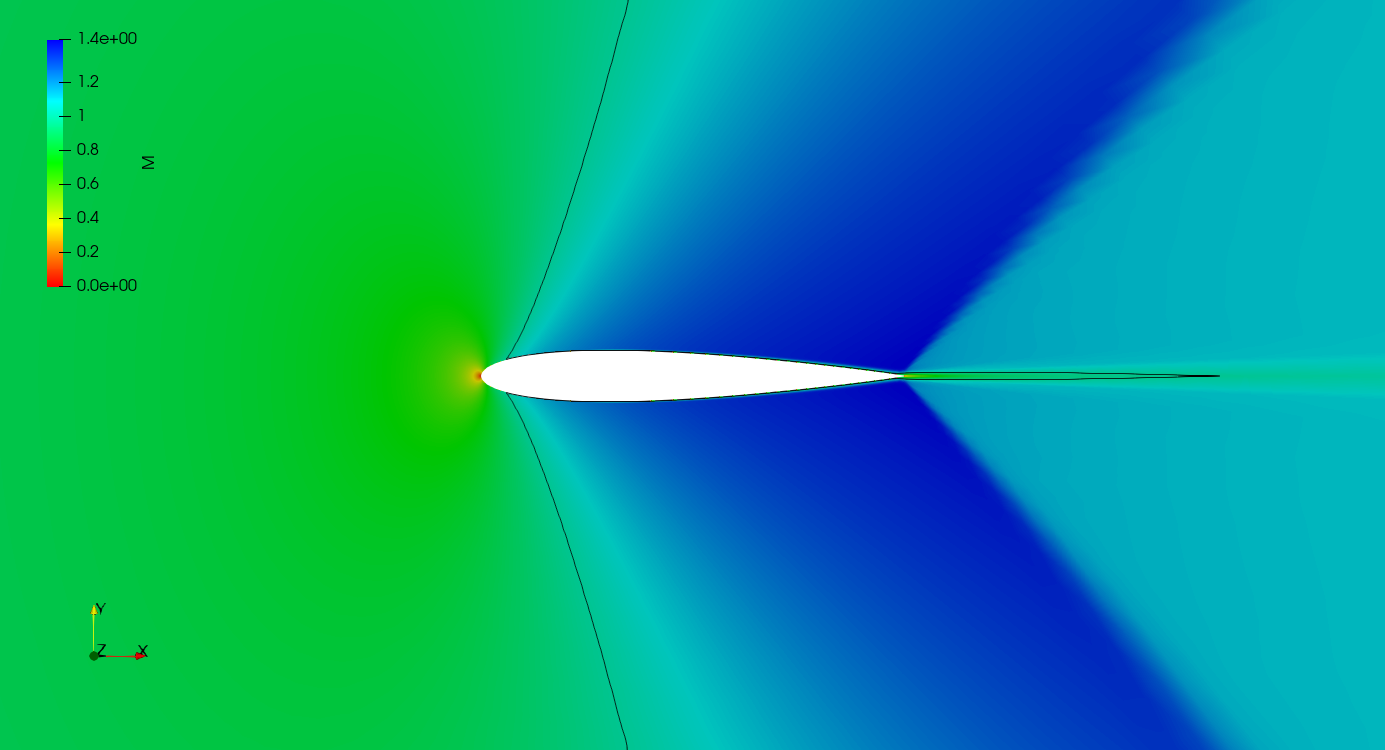Mach number for $M_{\infty}=0.99$

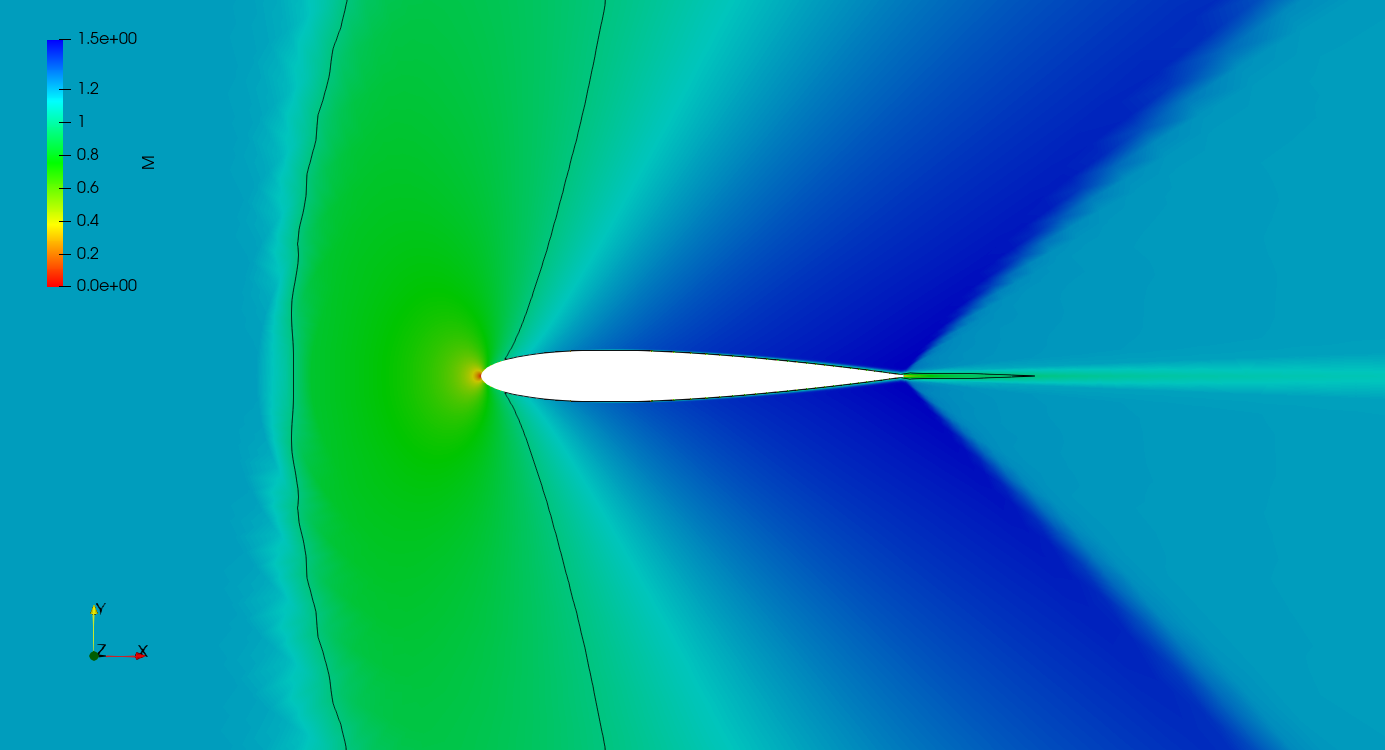Mach number for $M_{\infty}=1.20$

I understand that as velocity decreases, temperature rises, but I have another file that shows the evolution of static pressure and it does not increase as static temperature does, after the shock in the BL.

Thank you

Answer 1

Due to no-slip condition, the flow immediately adjacent to the wall has no velocity relative to the wall. If the flow is adiabatic everywhere and there is no significant viscous stresses (more on that later), then the total enthalpy ($h_0$) is constant; if we assume that the gas is calorically perfect (good assumption for air at low supersonic speeds) and that the heat capacity is constant (good assumption for small temperature variation), then:

$$h_0=c_pT_0=c_pT+\frac{1}{2}V^2$$

where $T$ is the static temperature, $T_0$ is the total temperature, $V$ is flow speed.

Immediately adjacent to the wall, the flow static temperature will increase toward the total temperature (around 315K in your example). This occurs in boundary layer both before the shock and after. The boundary layer before the shock is thin, so you can't really see it when zoomed out. After the shock, the boundary layer is greatly thickened along with an amplification in turbulence, so the effect is much more prominent.

Now let's revisit the part about viscous stresses, which are prevalent in the boundary layer. Viscous deceleration is not exactly adiabatic (Ref. Hill, Mechanics and Thermodynamics of Propulsion), so there is decreased total temperature immediately adjacent to the wall (characterized by the recovery factor), and an increase in total temperature farther away, before tending to the free-stream value.