How does a weak shock-wave boundary-layer interaction create wave drag (other than through direct shock losses)?

Question

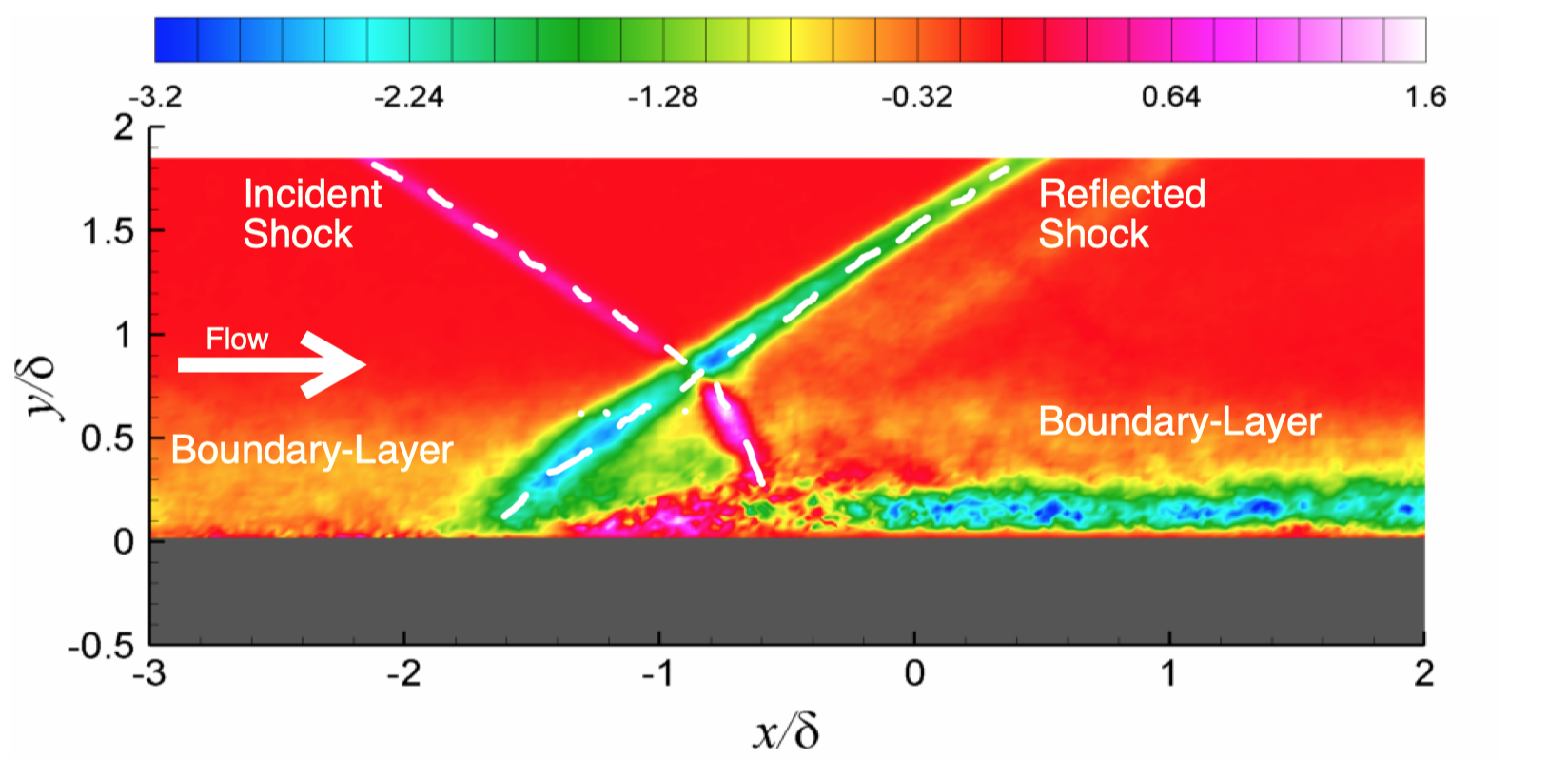
I'm trying to understand the physical cause of wave drag, beyond the simple statement "the presence of shockwaves increases the drag".

As far as I understand, in the case of a weak BLSWI (so without separation), the drag increase is due to:

• Direct shock losses (momentum deficiency of freestream flow through shockwave)
• A change in the state of the boundary layer due to the compression waves in side the BL

But how exactly does the second point work? If the BL becomes thicker after the SW, wouldn't that mean that the drag decreases because the velocity gradient and therefore the shear stress decrease?

I feel like I'm missing some tiny aspect...

---

Edit: I've found the following statement in this report, which maybe explains my problem better:

The increase in drag occurs directly because of the wave drag associated with the presence of shock waves. However, the drag also increases because the boundary layer thickness increases due to the sudden pressure rise on the surface due to the shock wave, which leads to increased profile drag. Lynch has estimated that at drag divergence the additional transonic drag is approximately evenly divided between the explicit shock drag and the shock induced additional profile drag

Why does the increase in BL thickness cause more drag? Shouldn't the velocity gradient at the wall reduce, leading in less shear stress? Or should I see it in a way that the already turbulent BL "takes up more energy in its vortical structures" from the flow, due to the increase in thickness?

Answer 1

As you know, wall shear stress $\tau_w$ (and thus friction drag) can be described as: $$ \tau_w = \mu \frac{\partial u}{\partial y} \vert_{y=0} $$ where $\mu$ is the dynamic viscosity of the fluid and $\frac{\partial u}{\partial y} \vert_{y=0}$ is the fluid velocity gradient at the wall.

The shock-wave boundary-layer interaction (SBLI) can change these two factors through several mechanisms:

1. A change in boundary-layer thickness: As you noted correctly, an increase in boundary-layer (BL) thickness will decrease the velocity gradient, compared to an otherwise similar BL velocity profile. However, my conclusion after reading several publications is that the increase in BL thickness doesn't always occur. BL thickness seams to scale with shock strength and very weak shocks actually produce a thinner BL.

2. A decrease in fluid velocity: The shock-wave will obviously result in a decreased velocity outside of the boundary layer, which decreases the velocity gradient. This will also decrease wall shear stress, so it cannot be the dominant mechanism either, since it contradicts our experience of increased friction.

3. An increase in turbulence: The turbulence of the BL is significantly amplified by the shock-wave through different mechanisms. The image below visualises the distribution of reynolds shear stresses, which are a measure for turbulence. A high magnitude (positive or negative) of reynolds stresses means high turbulence: ^{Reynolds shear stress distribution of a SBLI. Notice the strong negative values in the lower BL behind the SBLI. Negative values indicate slow fluid moving upwards and fast fluid moving downwards. Also notice the value is zero very close to the wall, because the wall prevents vertical fluctuations. Image Source (I added the white markings)}
   The increased turbulence causes an increased momentum exchange between the lower and slower part of the BL with the higher and faster part. This momentum exchange changes the velocity profile of the BL. There are higher velocities closer to the wall. This results in a higher velocity gradient at the wall. I believe this must be the main cause of increased wall shear stress. The stronger turbulence will probably decay downstream, so the velocity profile will slowly change back to the normal turbulent flat plate type.

4. Increase in pressure and temperature by the shock-wave: The rising temperature and rising pressure (not so much) increase the dynamic viscosity of the fluid and thus increase shear stress.

Most likely there are other factors that also play a role. I am no expert in SBLIs, I only read through a fair amount of literature to come to my conclusions.

---

Literature that I found especially helpful:

• Anderson, J.: Fundamentals of Aerodynamics : My standard book for aerodynamics. Fairly easy to read. Section 9.10 briefly covers SBLI.

• Schülein, E.: Skin-Friction and Heat Flux Measurements in Shock/Boundary-Layer Interaction Flows This paper shows the skin friction along an SBLI for different shock intensities. It provides schlieren images and figures that show the thickness of the boundary layer. It also discusses the causes for the observations.

• Schülein, E.: Documentation of Two-Dimensional Impinging Shock/Turbulent Boundary Layer interaction Flow Here the velocity profile of the BL is measured at different locations for the same test setup as above. All the interesting data is presented in the appendix. You can see how the SBLI changes the velocity profile and BL thickness. It also explains how to calculate the skin friction from the velocity profile data (quite complicated)

• Humble, R. Et al.: PIV Measurements of a Shock Wave/Turbulent Boundary Layer Interaction This is also the source of the image I used. They used Particle Image Velocimetry to measure the flow field of an SBLI. The part about turbulence is very interesting.

• Anyiwo, J. and Bushnel, D.: Turbulence amplification in shock-wave boundary-layer interaction This paper investigates the mechanisms that amplify turbulences in an SBLI. Also very interesting.

• Schlichting, H. and Gersten, K.: Boundary-Layer Theory Great book, every aspect of BLs. Detailed, long, harder to read than Anderson.