Why does indicated stall speed change?

Question

Aviation confusing me... I’ve read that stall speed doesn’t change (IAS) no matter what altitude you’re flying - of course under specific conditions ISA, 1G level flight, no wind, gross weight etc. then why I keep witnessing for example in the Boeing 737-800 PFD (primary flight display, intend to the barber pole) that at low altitude let’s say your stalling speed is around 140CAS when at cruise level stalling speed is way above - approximately 220CAS (random number) Why is that ? Doesn’t stalling speed must to be the same at all altitudes?

Thank you all for your answers!

Answer 1

It happens because of a compressibility error in the airspeed indicator (yes, even in the digital ones, since the error is not mechanical, but a physical property of the air).

As you might be aware, the speed indicated on your instruments is not really a speed at all, it is actually a pressure. Your pitot measures a deltaP between static and pitot and displays that pressure difference on a scale noted in knots.. we call that Indicated Airspeed.

This indication, because of changes of density, can be quite a bit different from your actual True Airspeed, but people didn’t care all that much about this difference, since the way the wing flies is reliant on that deltaP pressure difference anyway, so that you will actually stall at the same Indicated Airspeed, but not at same True Airspeed. (there is less density higher up but you are now traveling a bit faster, so the effect cancels out and the wing will behave the same)

The instrument was kept as it is in the cockpit (even though today we call it Calibrated Airspeed after a few changes) as a very usefull indication to the pilots.

Ok, now back to the question: turns out when planes begun flying faster and faster, a second error, caused by compressibility appears, that makes the airspeed indicator over-read. The pitot pressure is reported higher than it should be because at high speeds the air compresses in the pitot and makes the pressure there artificially higher, the deltaP is higher, thus our Indicated Airspeed is higher. Of course, this pitot tube phenomenon is not happening on the wing, so now we have a problem: we have a high speed indicated at the instrument, but most of it is actually just compressed air. Our Equivalent Airspeed is in fact much lower. And the wing will in fact always stall at the same Equivalent Airspeed. That means your Indicated Stall Speed in the cockpit will be ever higher as you climb because of compressibility.

Now, instruments were still kept to display IAS instead of the EAS, and a provision has been made where the stall barber pole advances up as you climb to make up for compressibility error

Then why haven’t the instruments been changed to show EAS directly? That is a harder question to answer definitively. I suppose by now people were already too used to having IAS in the cockpit, and the error is only really a factor for jet planes flying above (say) + 20 000 feet at speeds above Mach 0.5.

Answer 2

The difference is the change in Mach number over altitude.

And it is more than just the compressibility error in the IAS indication.

The maximum lift coefficient of a wing goes down with Mach number. While at sea level and 140 KIAS you fly at 21% of the speed of sound (Mach 0.21), at cruise altitude (I guess that means 30,000 ft) the true speed is already 360 KTAS which -- together with the decline of the speed of sound at lower temperature -- translates to Mach 0.63.

In order to estimate the change in maximum lift coefficient, look at the factor maximum lift coefficient times Mach squared: Above maybe Mach 0.4 to 0.5, this is what should stay (roughly) constant. A typical value for a modern wing would be 0.4, so we divide this by 0.63² = 0.397. Thus your maximum lift coefficient at Mach 0.63 has dropped to about 1.0. At lower altitude the maximum lift coefficient of the clean wing is closer to 1.6.

Technically, the wing might even be able to create higher lift coefficients at Mach 0.63, but buffeting will make this intolerable. The stall speed at cruise level, therefore, is the buffet speed and cannot be directly compared with the (real) stall speed at sea level.

Answer 3

I'll assume you're talking about the barber pole presentation on the speed tape, flaps up at low altitude and flaps up at high altitude, with the pole moving higher on the tape at high altitude. The barber pole indication and shaker firing point isn't related to the actual stall; it includes a computed safety margin that takes into account various factors like pitch motions and G loads. That's why the barber pole moves around as you maneuver and pull pitch.

So the actual "indicated" stall speed doesn't change with altitude but what does change is the Stall Protection Computer's stick shaker (and pusher on airplanes that have them) trigger margins and barber pole indications, which have to allow for huge increase in inertial effects at high altitude (the mass is the same, but the air is thin).

The high you go, because of the magnified inertia effects relative to aerodynamic pressure (indicated speed)in the thin air, the more the Stall Protection Computer has to "lead" a change in angle of attack to provide a shaker trigger point that gives a decent margin above the actual aerodynamic stall.

Answer 4

The key of this question is the way the drag changes with speed and the maximum thrust the engine can develop at very high altitude, please read carefully the answer, it is a bit complicated, but I am confident

Let us first of all understand what is Vimd (Indicated minimum drag).

Said simply it is the speed of minimum drag, but let us understand a bit more

Drag has two components: induced drag and parasitic drag

• induced drag is directly related to lift production and is greatest at low speeds and high angle of attack
• parasitic drag increases in proportion to the square of the aircraft speed.

At any speed total drag is the sum of the above two components

For obvious economical consideration it is desired to fly with the lowest total drag which is also compatible with the desire of not using oversized engines

The following curve gives the graph of total drag versus speed

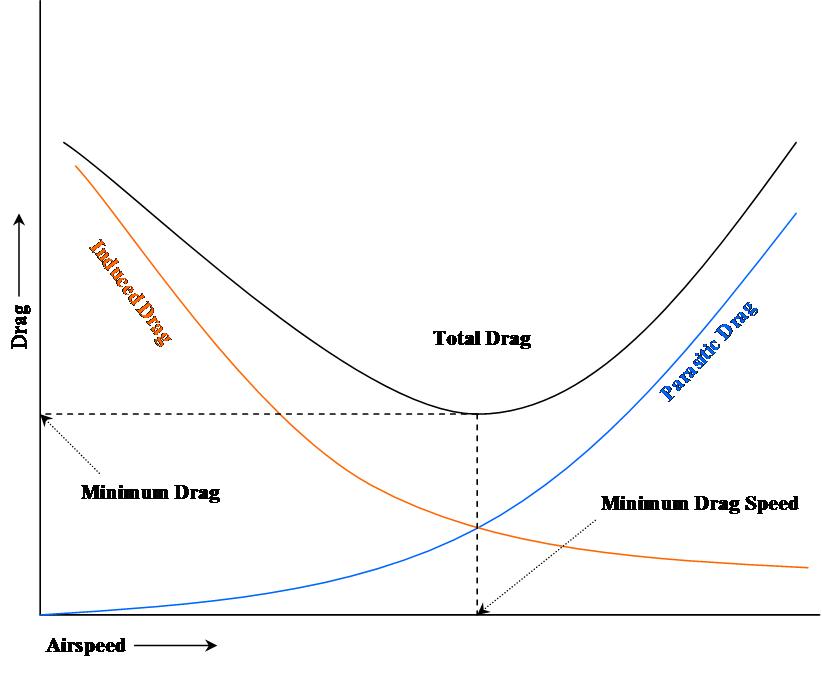
It appears that Vimd corresponds to the intersection point of the induced drag curve and parasitic drag curve

To answer the question

at low altitude let’s say your stalling speed is around 140K IAS and at cruise level stalling speed is approximately 220K IAS, Why is that?

For better understanding let us consider straightforward what happens at very high altitude.

When flying at high altitude, that is at an altitude where the engines thrust is limited by the low air density, and the pilot being therefore flying very closely to Vimd, if for a reason or another he reduces his speed, or his speed decreases, though still above the stalling speed, his actual engines thrust will rapidly become insufficient to overcome the increasing total drag increased by the speed reduction, as visible on the above curve. To maintain altitude he has to pull on the stick,( thus increasing the AOA and the drag), and simultaneously increase the thrust rapidly; if the thrust is not adequately increased, his speed will rapidly decrease and he might suddenly hit the real stalling speed. To avoid the above scenario the barber pole minimum speed is voluntarily well above the stalling speed, to increase the pilots reactivity.

Normally at high altitude the autopilot and the auto throttle are engaged happily safely they look for the barber pole speed limit and not for the theoretical stalling speed.

the higher the altitude the larger the gap between the barber pole minimum speed and the theoretical stalling speed

Note, June 22, 2019. slightly edited for better comprehension.