Source: Wolfgang Langewiesche - Stick and Rudder; An Explanation of the Art of Flying
In the first chapter the author mentions, in a glide, if you want to descend more steeply, you want to want to point your nose less steeply. And if you want descend less steeply you point your nose more steeply.
What is the reason for this?
The statement is only true if you fly at a speed below the best glide speed. For simplicity, let's look at a glider: Below you see the plot of the L/D ratio over speed of the DG-1000 two-seater glider:
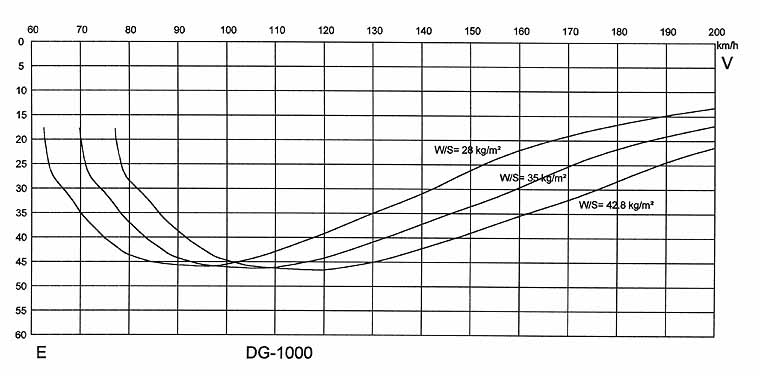
Glide ratio over speed diagram for three different wing loadings (source). If we just look at the lowest wing loading (leftmost curve), the best L/D can be achieved when flying at 95 km/h. Minimum speed is around 62 km/h, so it is entirely possible to fly along at 80 km/h. When the pilot reduces the angle of attack, the aircraft will pitch down and speed up. If it now flies at 90 km/h, he has just improved his L/D from 43 to 46, so he will descend less steeply when dropping the nose.
Note that our flight path angle is now less steep, and the change in attitude is less than the commanded change in angle of attack. Nevertheless, the pitch attitude is reduced and the pilot has the impression that he dropped the nose.
The same is true in reverse when slowing down. When approaching the stall speed, large angle of attack changes will result in relatively small speed changes, so the effect of a steeper descent when pitching up becomes more pronounced as the airplane slows down.
The underlying reason is the fact that drag will increase when the pilot moves away from the best L/D speed. Slowing down will increase drag, and more drag will result in a steeper descent. As soon as he shifts his starting point to higher speeds, things reverse and the aircraft will descend more steeply when the nose is lowered.
Adding an engine will not fundamentally change this behavior. It is only easier to explain when using gliders.
That comment by Wolfgang Langewiesche in his book "Stick and Rudder" has caused lots of discussion over the years. Maybe that is what he intended when he worded it so ambiguously.
"By pointing the nose down less steeply, you descend more steeply. By pointing the nose down more steeply, you can glide further"
This is really only true if you are flying just a little bit slower than best glide speed (best Lift /Drag ratio) or (best glide distance). Also, that statement is really only true if you point the nose up or down just a little.
Best glide distance means the speed at which the most distance is traveled for the least amount of sink. If you are flying slower than the best glide speed, putting the nose down and going faster, so that you are closer to the best glide speed, will allow you to cover more distance as the aircraft sinks.
If you are already flying at the best glide speed and point the nose down, you will no longer be at the beast lift to drag ratio. You will increase the speed and distance traveled, but you will also increase the sink rate and drag from the higher airspeed. That will result in a steeper descent because you are no longer at the best lift to drag ratio.
If you point the nose down to 60 degrees below the horizon I guarantee you will descend more steeply not less steeply and NOT glide farther as Langewiesche says in the book.
The key thing here is that he is referring to the ANGLE of descent, not the RATE of descent.
If you push the stick forwards the rate of descent will always increase, but the angle of descent may actually decrease. This is because you go faster so you generate more lift, thus your overall angle of descent will decrease.
A typical beginner's mistake some glider pilots make is that they get worried they are not descending fast enough when they are landing so they push the stick forwards to dive--a big mistake. What happens is they go a lot faster, so they tend to overshoot even more. Pulling back on the stick, slowing the aircraft down and flattening the pitch is what to do to steepen the angle of descent, just as Langewiesche says.
This is apparently talking about maneuver around the best glide speed. The behavior of an airplane is counter-intuitive then.
Pushing the nose down, increases the speed and causes the angle of descent to decrease.
Pulling the nose up, decreases the speed and causes the angle of descent to increase.
