At the hypersonic speeds typical of space vehicles reentry (Mach number much bigger than 5) air becomes an incandescent plasma mixture of ions O$^+$ and N$^+$ and electrons and radios do not work anymore. It is a complicated chemical matter but, on the other hand, the aerodynamic behaviour becomes extremely simple. To explain subsonic and supersonic aerodynamics, at least a couple of books of hundreds of pages are needed. But to explain hypersonic aerodynamics a short chapter might be enough.
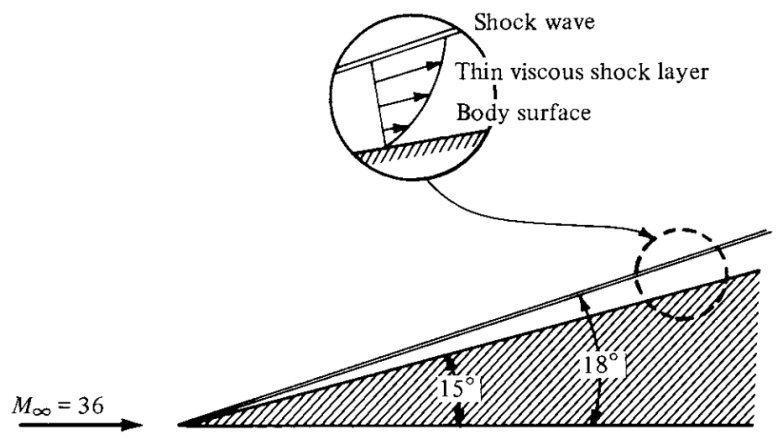
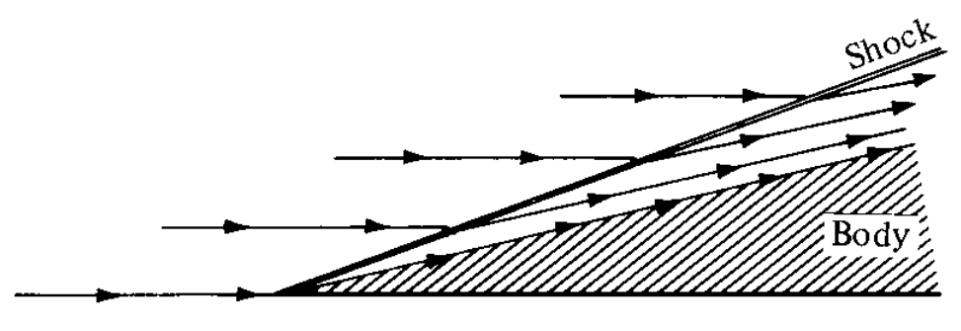
Indeed, at these extreme Mach numbers, the shock wave becomes so bent toward the body that it practically coincides with the body surface itself¹:
This is a huge simplification: if we follow one particle of air, it simply flies on a straight line until it bumps into the body; at that point it just bends its trajectory to follow the body surface:
The particles of air flowing over the surface of the body do not impact with it and they just keep on flying straight on: the "shadowed" surface do not experience any aerodynamic force and the air there just remains undisturbed: only the surface of the body being directly impacted by the air experiences an aerodynamic force.
That's the all story about hypersonic aerodynamics! Very simple indeed.
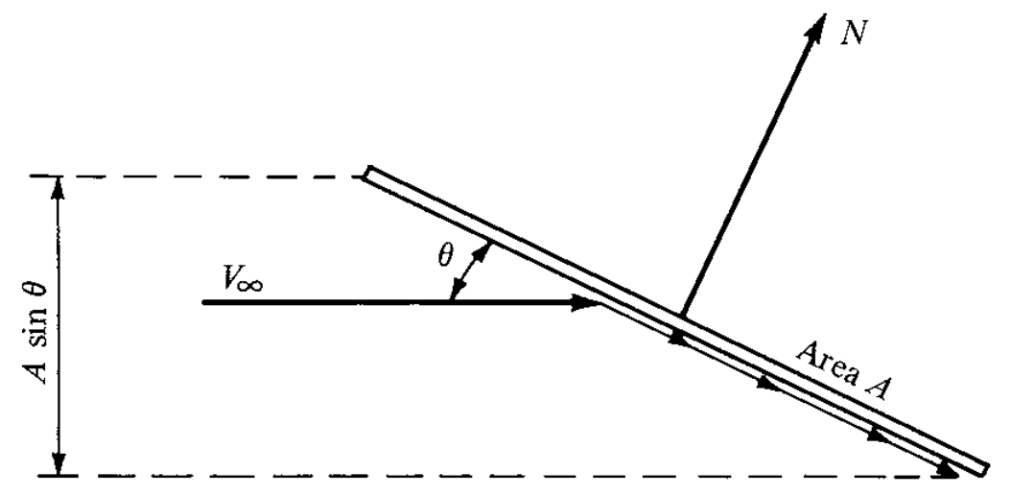
Since upon impact the particle has changed its speed (the speed perpendicular to the surface has become zero), then by Newton's second law ($F=ma$) all the particles of air bumping into the body generate a force ($N$ in the following picture) which is:
- perpendicular to the body surface and;
- with a value proportional to the mass flow times its perpendicular deceleration to zero:
For a flat plate the maths becomes really easy and simply returns:
- $C_l= 2sin² α \times cosα$
- $C_d= 2sin³α$
which look something like that ($C_l$ in red, $C_d$ in blue, my own work):
A couple of comments are definitely worth noting:
- $C_l$ is not linear as it is instead at subsonic speeds.
- $C_l$ starts at 0 at 0°, reaches a maximum and then decreases going again to 0 at 90°; the fact that it posseses a maximum has nothing to do with viscosity or stall or delta wing but only with the fact that at hypersonic speed the aerodynamic force is always perpendicular to the surface; there's no stall at hypersonic speeds.
- For the same reasons, $C_d$ increases from 0 at 0° to 2 at 90° with an increase that, at least for small AoA, is cubic with AoA i.e. proportional to "³"; at subsonic speeds $C_d$ is simply constant at small AoA.
- $C_{l_{max}}=0.77$ @ 54.7°; for a standard subsonic NACA 0012, $C_{l_{max}}$ is some 1.6 @ 22°, i.e. twice as much @ half AoA.
- Obviously in reality at zero AoA $C_d$ is not zero but equals the drag due to viscosity $C_{d_0}$ and the whole plot can be simply shifted upward of $C_{d_0}$.
- Maximum efficiency $L/D$ is between 6 and 7 while for the same NACA 0012 of before it is more than 20 i.e. three time as much.
- Albeit being deduced for a flat plate, the two equations for $C_l$ and $C_d$ show a very good agreement with the aerodynamic characteristics of 3D slender bodies.
Now that we have learned the whole hypersonic aerodynamics (as promised in just one short chapter), how elevons worked on the space shuttle is easy to be understood:
- first of all just forget about subsonic aerodynamics and any subsonic phenomenon related to moving surfaces; as seen, aerodynamics does not work in that way at hypersonic speed! There's no stall, lift is half at best and not linear with AoA, drag is bigger and so on.
- Second of all, in order to present its belly to the atmosphere at reentry, the space shuttle maintained an AoA of 36 to 40°.
This is important: from the previous plot it can be seen that, at those AoA, lift and drag at hypersonic speeds have basically the same magnitude; the subsonic NACA 0012 of before at the same $C_l$ of 0.65 has a $C_d$ of 0.03 i.e. 20 times smaller! Furthermore, $C_l$ is already close to its maximum value: deflecting the moving surface downward does not have a big impact on the total $C_l$ (it might actually even decrease it) while it does modify $C_d$ a lot.
So, due to the peculiar aerodynamic characteristics at hypersonic speeds and due to the high AoA of the reentry phase, manoeuvring the space shuttle was achieved acting more upon its drag than its lift. And this finally explains why:
To effect a right turn, we actually deflected the right elevon down which created more drag on the right, yawing the vehicle right.
¹ all pictures from here