4
$\begingroup$

Looking at navigation theory right now and I'm stuck in the understanding of TAS and GS. How does GS determine the time interval it takes for an aircraft to fly from the DP to the DEST, if TAS determines how the fast the aircraft is really going through the air.

My current guess is that GS accounts for the wind, so that determines how fast it is going relative to the ground; thus determines the time interval.

Improve this question
$\endgroup$
1

2 Answers 2

Reset to default
6
$\begingroup$

Computing GS takes into account the wind and how fast you are actually traveling through the air-TAS

For example, if you are flying east bound through a mass of air (the "wind" refered to in your question) that is moving from east to west (opposite direction to your flight path) at 100 knots (pretty big headwind) and your true airspeed is 250 knots (your actual speed through the air) then your ground speed (speed over the ground) will be 150 knots. (TAS - Headwind: 250 knots - 100 knots = 150 knots GS)

So, without applying any other variables, the time from your departure point to your destination will be based on 150 knots ground speed. If your destination is 150 nautical miles from your departure point, your enroute time (time interval in your question) would be 1 hour.

So, why do you need to know your TAS?

During preflight planning you need to know what your planned TAS will be (from your aircraft's performance information/tables) based on the altitude you plan to fly, temperature, power setting, etc. Then, after consulting the forecasted enroute weather (e.g. wind direction, wind speed, temperature, etc.) that you will encounter along your route, you can compute your expected ground speed. So, knowing what your TAS will be is a necessary step in determining what your ground speed will be.

Simple as that. (Ground speed) "GS accounts for the wind" as you say in your question.

Improve this answer
$\endgroup$
9
  • 1
    $\begingroup$ An interesting fact is, if you were to turn around and fly back to the original point with the exact same wind component, the tailwind will never be able to make up for the lost time due to the headwind. A return flight in still air will always take less time. $\endgroup$
    – Mike Sowsun
    Commented Dec 1, 2021 at 3:21
  • $\begingroup$ @MikeSowsun: your point has always been an interesting brain teaser. But when you put the arithmetic to the question the conundrum seems a bit more clearly understood. Eastbound into 100 kt headwind with a true airspeed of 250 kts results in a GS of 150 kts. Flying the reverse (westbound at 250 kts true airspeed) with a tailwind of 100 kts results in a GS of 350 kts. More than double the benefit provided by the tailwind over the detriment of the headwind, (all other things being equal). $\endgroup$
    – user22445
    Commented Dec 1, 2021 at 3:43
  • $\begingroup$ After a couple more read throughs of my textbook, as stated my GS is the time interval it will take for me to travel btn point A and point B. What's the importance of TAS then? $\endgroup$
    – JandyPilot
    Commented Dec 1, 2021 at 5:45
  • $\begingroup$ TAS is the “true” airspeed through the air. GS is the speed over the ground which is the result of any adding or subtracting to account for the movement of the air mass (wind). In still air TAS and GS are equal. TAS is important in flight planning so you can estimate what your GS will be. Once airborne your GS is important to know so that you can calculate how long the trip will take, and if you have enough fuel on board to make it your destination. $\endgroup$
    – Mike Sowsun
    Commented Dec 1, 2021 at 14:46
  • $\begingroup$ @user14397644: In preflight planning you need to know what your planned TAS will be (from your aircraft's performance information/tables) based on the altitude you plan to fly, temperature, power setting, etc. Then after consulting the enroute weather (e.g. wind direction and wind speed) you will encounter on your route you can compute your expected ground speed. So, knowing what your TAS will be is a necessary step in determining what your GS will be. I have expanded my answer above based on your comment. $\endgroup$
    – user22445
    Commented Dec 1, 2021 at 17:00
3
$\begingroup$

How does GS determine the time interval it takes for an aircraft to fly from the DP to the DEST, if TAS determines how the fast the aircraft is really going through the air.

The simple answer to this question is when you calculate the time to fly from DP to DEST you need to use distance and velocity t = d/v. But d and v need to be referenced to the same frame of reference.

  • DP and DEST locations are referenced to the ground frame.
  • The distance d is therefore computed in the ground frame.
  • v must be expressed in the ground frame, this is GS.

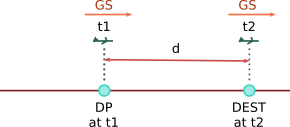
enter image description here

Computation of d in the ground frame

This is really simple but you need to know GS. If you know TAS and wind velocity, then the next method can be used.

While d and v must be in the same frame, this frame could be the air mass frame as well:

  • TAS is aircraft velocity measured relatively to the air mass frame.

  • To compute d in the air mass frame you would need to take into account that DP and DEST are moving relatively to the air mass frame due to the wind.

    The distance d to cover is not the distance on the ground, but the distance between points in the air mass which coincide with the verticals of DP and DEST at the time the aircraft reaches these air mass points.

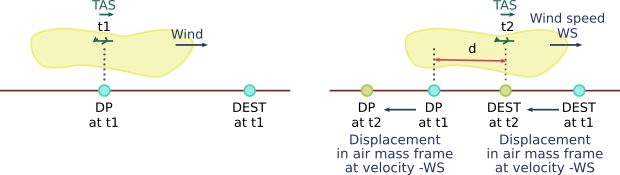
enter image description here

Computation of d in the air mass frame

Therefore to determine d you would need to take into account the air mass velocity relative to the ground, that is the wind velocity (more accurately the wind velocity vector projected on the flight path).

This time you need to know both TAS and wind velocity.

My current guess is that GS accounts for the wind

As you infer correctly there is no mystery when using GS, GS is the sum of TAS and wind:

enter image description here

GS is related to TAS via wind velocity

So if you're lucky enough to know GS and use the ground frame, which is more simple, to compute the trip time, it's because someone or a tool already did the sum.

The third reference frame, the aircraft frame, could be used as well, d and v would need to be expressed in this frame. "I let the reader do the exercise" :-)

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .