2
$\begingroup$

Considering aeroelastic flutter, when an eigenfrequency of the wing meets with an aerodynamic frequency, the commonly mentioned solutions make sense: avoid the convergence zone by limiting speed, or by increasing the wing's eigenfrequency trough stiffness, reduced weight, or reduced span.

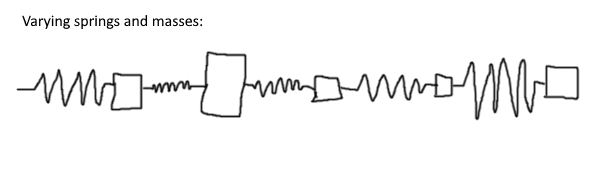
Makes sense, especially if I use the spring and mass model for the wing: enter image description here

I'll use the analogy with the conventional spring as it makes things easier to understand for me.

So Wikipedia says: "Prediction involves making a mathematical model of the aircraft as a series of masses connected by springs and dampers which are tuned to represent the dynamic characteristics of the aircraft structure. [...] Small carefully chosen changes to mass distribution and local structural stiffness can be very effective in solving aeroelastic problems."

My 3 questions are:

1: Do I understand correctly that with this approach, the wing will act similarly to the following model, where the motion is rather chaotic?

enter image description here

2: This should have no certain frequency. Does this mean flutter is avoided?

3: Does this mean flutter can be avoided by redistributing mass around the wing, without increasing stiffness or reducing mass?

Improve this question
$\endgroup$
3
  • $\begingroup$ so flutter is avoided here by having so many random eigenfrequencies in a single object that neither can really get excited much? $\endgroup$
    – Abdullah is not an Amalekite
    Commented Nov 3, 2020 at 15:27
  • $\begingroup$ According to my understanding, yes. $\endgroup$
    – GZoltan
    Commented Nov 3, 2020 at 15:34
  • $\begingroup$ 3: redistributing mass without reducing mass means increasing mass or introducing weak spots. That might avoid flutter, but it would definitely harm performance in many other ways. $\endgroup$
    – Camille Goudeseune
    Commented Nov 3, 2020 at 17:57

1 Answer 1

Reset to default
4
$\begingroup$

Do I understand correctly that with this approach, the wing will act similarly to the following model, where the motion is rather chaotic?

No, your combination of masses and springs will have several eigenfrequencies. For aeroelastic analysis only the lowest eigenfrequency counts because this will couple with aerodynamic frequencies at the lowest flight speed. Once you fly faster, the next eigenfrequnecy in line will resonate, but that is only hypothetical because flutter caused by the lowest frequency has already destroyed the airframe.

In very rare cases the lowest eigenfrequency will already couple with aerodynamic oscillations at speeds below stall speed. Then the aircraft can be operated in the gap between the lowest and the second-lowest eigenfrequency.

This should have no certain frequency. Does this mean flutter is avoided?

No, since it has several eigenfrequencies, flutter is as certain as before.

Does this mean flutter can be avoided by redistributing mass around the wing, without increasing stiffness or reducing mass?

Flutter cannot be avoided, only its speed shifted up by damping. The rule always is to place the center of gravity ahead of the axis of rotation (that would be the elastic axis in case of wing torsion), so any movement causes an inertial damping force. This is the reason for the forward location of wing mounted engines.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ You could mention that hydraulic control surfaces operate unbalanced, with the CG well aft of the rotation axis by virtue of the "rigid" hydraulic connection that makes the surface effectively a fixed part of the trailing edge, and if unpowered, the damping ability of the idle actuator is considered sufficient to prevent the imbalanced surface from reacting/magnifying movement in parent structure. If the damping ability of a dead actuator(s) is found to be insufficient, then additional passive flutter dampers are required. $\endgroup$
    – John K
    Commented Nov 4, 2020 at 3:33
  • $\begingroup$ "The damping ability of the idle actuator is considered sufficient..." VSS Enterprise? For moving/turning surfaces, how stable or unstable to be in the airstream seems to be the quandary, especially when Velocity goes well beyond hurricane force. $\endgroup$
    – Robert DiGiovanni
    Commented Nov 6, 2020 at 8:50

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .