2
$\begingroup$

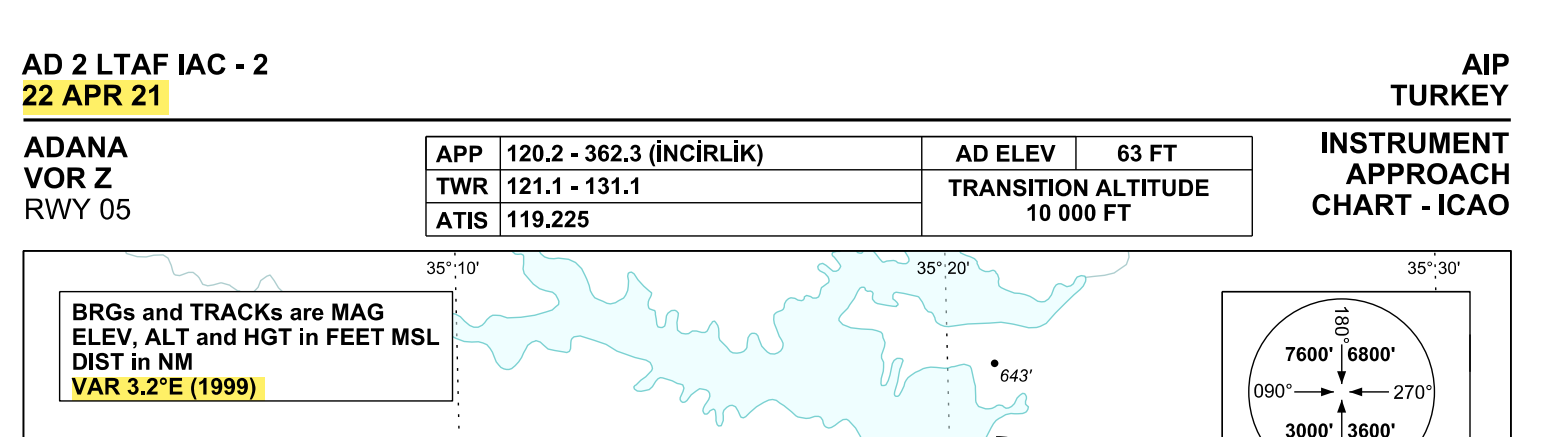
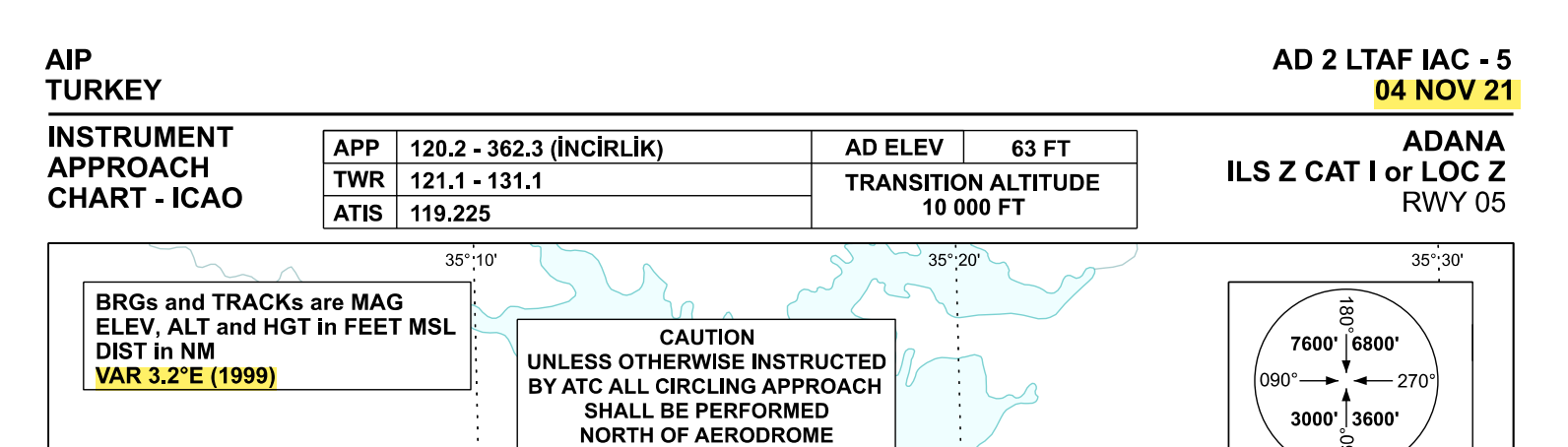
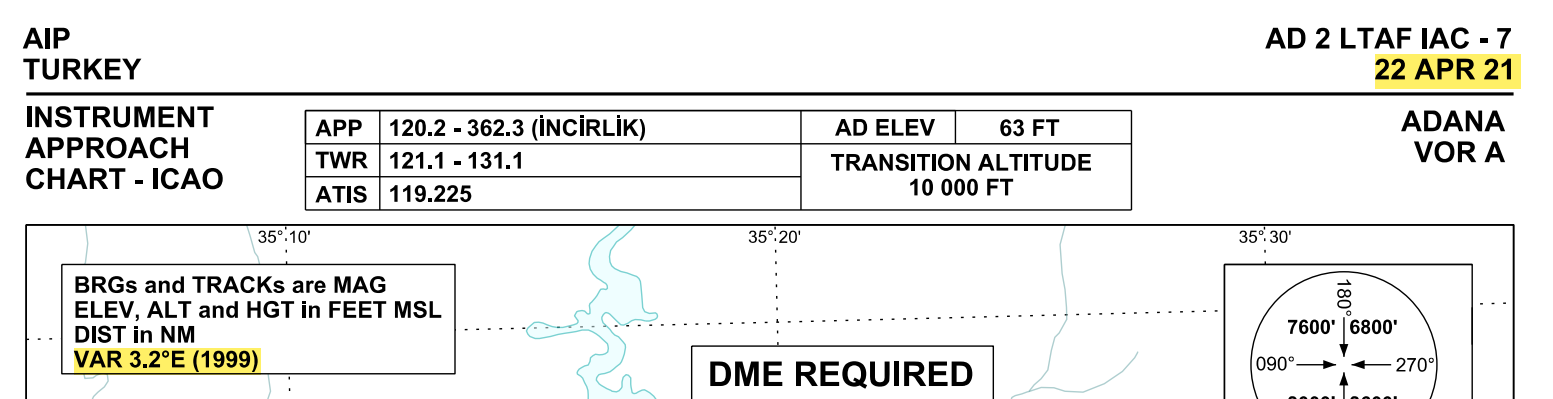
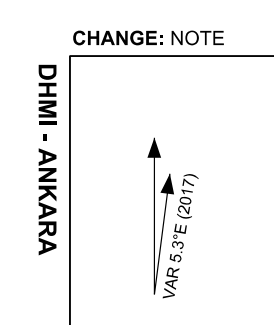
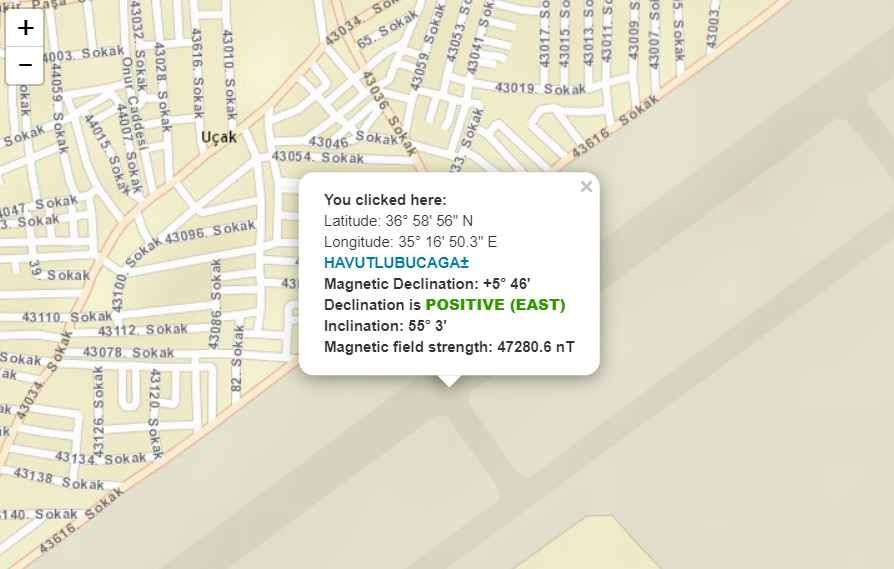
Why is the magnetic variation value from 1999, although the AIP Charts in my country are up to date? In all of the chart examples I shared below, the variation value is 3.2°E and belongs to 1999. According to my research, the current variation value of ADANA LTAF airport is 5° 46' E. The year the charts are published is 2021. In addition, the variation value of 2017, 3.5°E, is shared on the airport chart. Why are all these values out of date and not fixed? Why is such a way of sharing followed in the charts? Any ideas?

enter image description here enter image description here enter image description here enter image description here enter image description here

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ See this answer that is in response to a somewhat similar question involving magnetic variation updates in the U.S.:aviation.stackexchange.com/questions/100520/… $\endgroup$
    – user22445
    Commented Aug 24, 2023 at 16:53

1 Answer 1

Reset to default
-1
$\begingroup$

Because the magnetic declination in a given area changes slowly over time, as little as 2–2.5 degrees every hundred years or so, or by 1 degree every three years in locations closer to the poles. Depends what part of the world the map covers. Not many airport facilities close to the poles.

Improve this answer
$\endgroup$
4
  • 2
    $\begingroup$ The OP already knows that magnetic declination is changing with time. The question was about why the values on the charts are so old. $\endgroup$
    – Bianfable
    Commented Sep 6, 2023 at 17:12
  • 1
    $\begingroup$ @Bianfable Read my answer again. The reason is because the declinations change very slowly over time. Values will not be updated for small fractions in changes. $\endgroup$
    – Juan Jimenez
    Commented Sep 6, 2023 at 17:17
  • $\begingroup$ First of all, thank you for your answer. I can understand why it's not on every amendment chart because it changes so slowly. But when the new magnetic deviation is 5° 46' E, why are they still sharing the 1999 value of 3.2°E instead of changing it in the newly published chart? There is not a small difference, but a big difference of almost 2.5 degrees. Isn't this ridiculous? Because we have new data and they prefer not to share it in the current chart (2021) (?). Interesting.. $\endgroup$
    – pilot162
    Commented Sep 8, 2023 at 7:48
  • $\begingroup$ I suspect it's because few people are doing their navigation with methods that require taking deviations into account, and instead rely on GPS and other means of navigation data. $\endgroup$
    – Juan Jimenez
    Commented Sep 9, 2023 at 16:48

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .