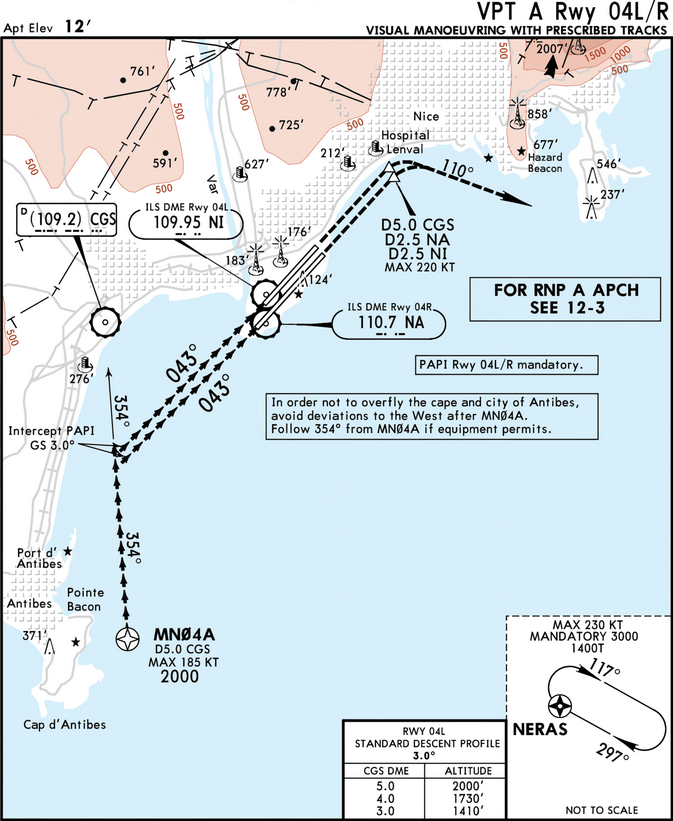
What you are describing is the VPT A (Visual Maneuvering with Prescribed Tracks) for Rwy 04L or 04R into Nice Côte d'Azur (LFMN) following the RNP A approach (which ends at MN04A):
Note that MN04A is the MAP (missed approach point) for the RNP approach with an MDA (minimum descent altitude) of 2000 ft. This means that you must be visual from that point on. The entire procedure shown above is flown entirely with visual references (you may use assistance to initially stay on the 354° track towards CGS, as the chart says, if equipment permits).
The point at which you would turn towards the runway is therefore determined by looking out of the window. There is no need to calculate anything, you just visually fly towards the runway. The only restriction is that you shouldn't deviate to the west on the initial segment towards CGS (to reduce noise in the city of Antibes). If you turn early, you can still correct to the left; if you turn late, you can still correct to the right. If you get the turn just right, you can intercept the PAPI for a visual descent towards the runway just after the turn.
If you misjudge the turn so bad that you can no longer safely correct your flight path to land, you would follow the Visual Balked Landing procedure (dashed lines) and then fly to NERAS (end of the missed approach procedure of the RNP A).
If the visibility is too poor to see the runway at MN04A or when there are clouds below 2000 ft, you would be cleared for the ILS or RNP Z approaches instead, which are both straight-in approaches overflying Antibes.
If you really want to calculate the point to start turning, consider the following sketch:
The first thing we need is the intersection point of the track 354° from MN04A and the extended runway centerline (I chose runway 04L here because that is the default runway for landing in Nice). I looked up the coordinates for the relevant points in my navigation database:
| Point | Latitude | Longitude |
|---|---|---|
| LFMN 04L | 43° 39' 9" N | 7° 12' 17" E |
| LFMN 04R | 43° 38' 48" N | 7° 12' 9" E |
| MN04A | 43° 33' 43" N | 7° 9' 14" E |
| CGS | 43° 38' 43" N | 7° 8' 45" E |
You can use the calculator on movable-type.co.uk to figure out the coordinates for the intersection point: 43° 36′ 28″ N, 007° 08′ 50″ E.
The next thing we need is the distance $D$ from the intersection point to CGS. The same page also has a calculator for that and gives $ D \approx 4.168 \, \text{km} \approx 2.251 \, \text{NM} $.
Now we calculate the turn radius $R$ using the formulas from this answer:
$$ R [\text{ft}] = \frac{V [\text{kt}]^2}{11.26 \tan \theta} \; , \qquad \omega [^\circ/\text{s}] = \frac{1 \, 091 \tan \theta}{V [\text{kt}]} $$
When flying a standard turn at 3 degrees per second, we can solve the second equation for $\tan \theta$ and insert it into the first one:
$$ R [\text{ft}] = \frac{V [\text{kt}]^2}{11.26 \, \omega [^\circ/\text{s}] \, V [\text{kt}] / 1 \, 091} \approx 96.89 \frac{V [\text{kt}]}{\omega [^\circ/\text{s}]} $$
Assuming 140 kt TAS, this gives a turn radius of $ R \approx 4522 \, \mathrm{ft} \approx 0.7442 \, \mathrm{NM} $. The corresponding bank angle is then given by (source):
$$ b = 57.3^\circ \arctan \left( \frac{V [\text{kt}]}{362.1 \, \text{kt}} \right) \approx 21.14^\circ $$
The last thing missing is the distance $d$ before the intersection point, where the turn should be started. With a bit of geometry, one finds that this is given by
$$ d = R \tan \left( \frac{\alpha}{2} \right) $$
with our known $ \alpha = 49^\circ $. This results in $ d \approx 0.3392 \, \text{NM} $.
This gives the final answer for the distance from CGS where the turn should be started:
$$ D + d \approx 2.59 \, \text{NM} $$
Note that this entire calculation does not take wind into account!