According to the relevant rules (for example 14 CFR 25.571):
The airplane must be capable of successfully completing a flight during which likely structural damage occurs as a result of -
- Impact with a 4-pound bird when the velocity of the airplane relative to the bird along the airplane's flight path is equal to Vc at sea level or 0.85Vc at 8,000 feet, whichever is more critical;
Note that in the calculation of the kinetic energy, the mass of the bird (4lb, 1.8kg) enters in the equation together with the designed cruising speed of the airplane Vc (and obviously not the bird's flying speed).
That value of kinetic energy should give you an idea of how piercing the impact can be. Translating the value of kinetic energy into a force is quite complicated since the force depends on the structural properties both of the bird and of the hit structure. That's why chicken guns are still in use despite the fancy simulation tools run during the design phase.
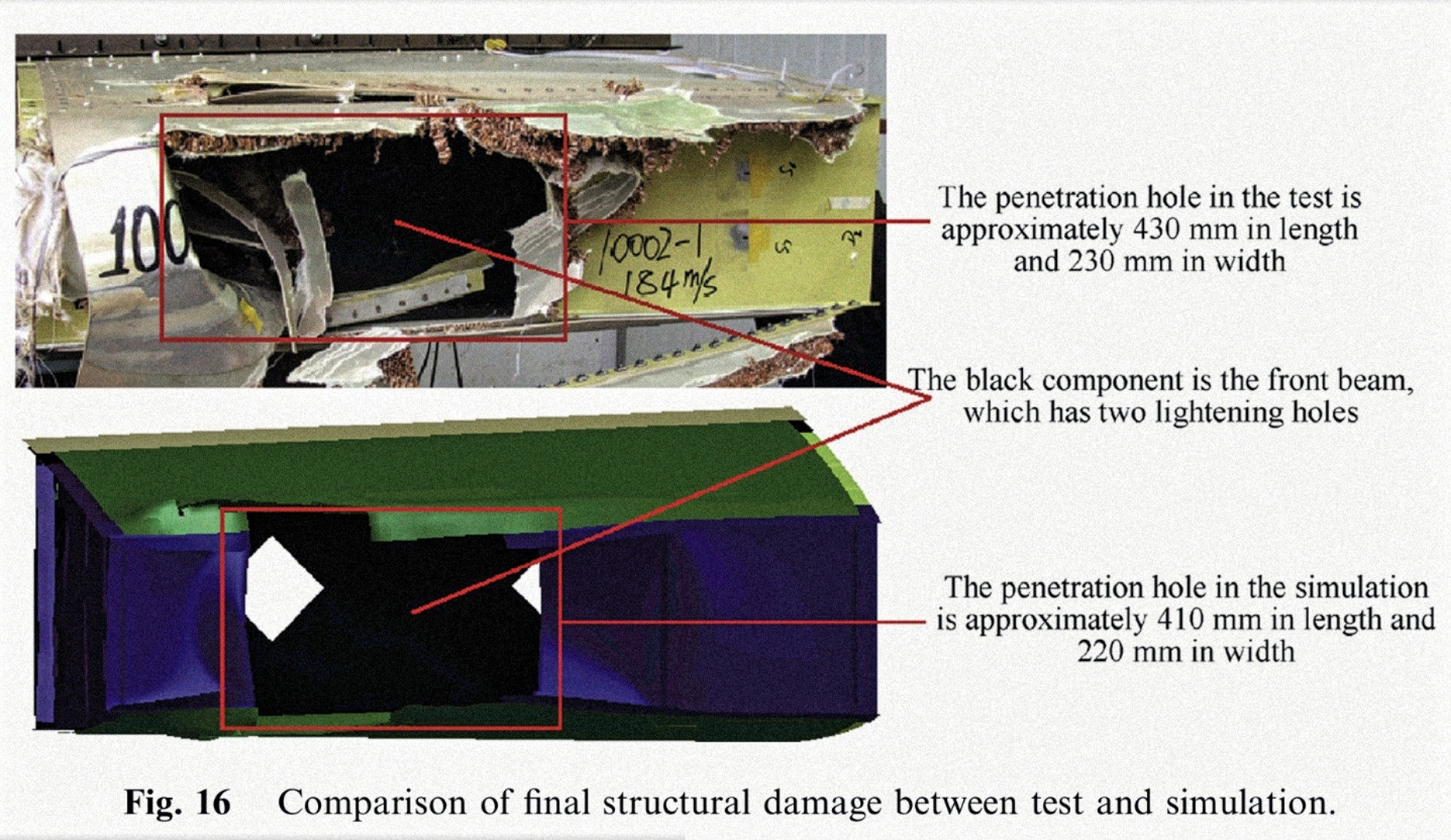
Just as example, this is how it looks like reality vs. simulation (all pictures from this paper):
Getting a ballpark estimate of the impact forces might be done using an energy approach with some simplification; we suppose that:
- the airplane's structure deforms in a spring-like way; actually after the impact the structure remains deformed and it might even be brocken, so it doesn't really behave like a spring;
- the impacting body does not disintegrate i.e. its kinetic energy is all transferred in deformation of the spring; this is also not perfectly true.
We have:
$E_\mathrm{k}=\frac12 mV^2=E_\mathrm{e}=\frac12 kx^2$
$F=kx$
Where $k$ is the rigidity of the spring, $x$ its deformation and $F$ the force needed to deform it.
Equating and extracting $F$ we get:
$F=\frac{mV^2}{x}$
Let's substitute some numbers:
- $m=1.8~\mathrm{kg}$
- $V=100~\mathrm{m/s}$
- $x=10~\mathrm{cm}=0.1~\mathrm{m}$
$F=180~\mathrm{kN}$