In short
This is counterintuitive. Ignoring Earth curvature not shown on maps, the routes which appear curved on the screenshots are actually nearly straight. Compare these two different projections:
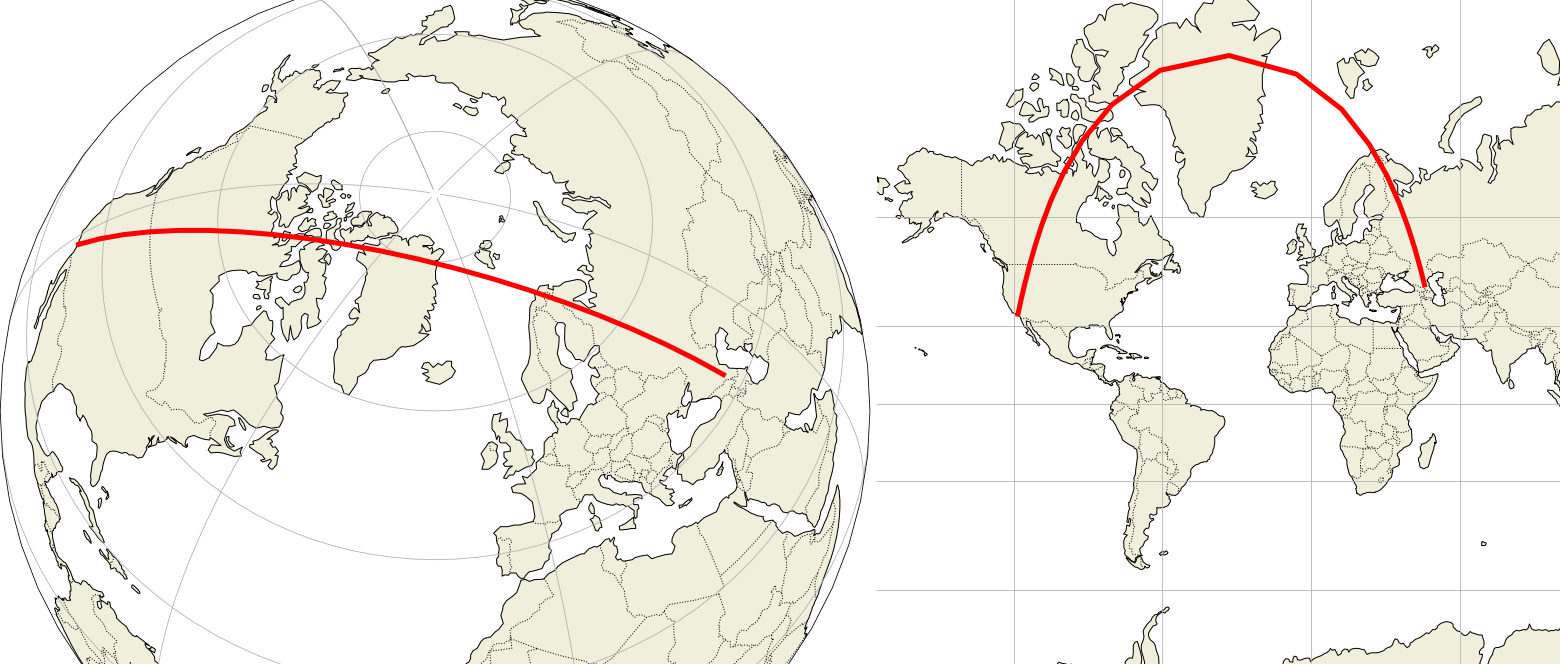
This is the same track, the same geographic landmarks are crossed. Still:
Left: We sense a bulging disk, the path is nearly parallel to two opposite meridians forming a circle, it must be a straight path.
Right: The path doesn't seem parallel to any meridian and we can't make sense of the heading change over Greenland. Difficult to see a straight path here.
Websites don't use the azimuthal projection of the left side because the map must be strictly straight (shortestdrawn with the path), but they try close to be. The reasonthe center, while Mercator projection is unique for the whole Earth. But there is a growing demand for an adaptive map representation, adjusting the projection while zooming in/out. With current server and client processing capabilities this is possible, see this demonstration.
Commercial routes are constraintsas straight as possible, deviations are due to be taken into accountconstraints, e.g. the maximum distance ofto available emergency airports, or the avoidance ofweather/conflict unsafe areas. But above that, crews avoid storms and often fly in areas of favorableunfavorable winds to reduce both trip time and fuel burnt. Winds found at cruise altitudes are commonly about 200 km/h.
Still the curves you show greatly distort actual routes, due to the map projection used to convert the 3D spherical surface into a flat surfaceDetails follow.
In 3D, a "straight path" on a sphere is a circle arccurve. Our brain is able to identify such circle as a straight linecurve "as straight" on azimuthal projections, exceptbut on someother types of map projection, specially on Mercator maps used to depict large areas, such equivalence makes no sense. Example with azimuthal projection on the left and web Mercator on the right:
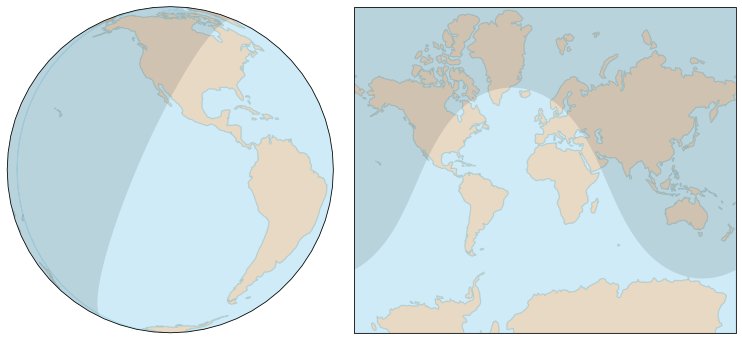
The map on the left projects the sphere on a disk (azimuthal projection), on the right it projects it on a rectangle (web Mercator projection). The shadow represents the night, and of course the separation between night and day is a circle (i.e., a straight path for an aircraft). This circle is easily identified onIt's not difficult to accept the azimuthal projection. The same circleidea the path is visible onstraight the Mercator projectionleft, but to be convincedon the right, it's nearly impossible. However looking closer, we need to look atsee the locations crossed and confirm they are indeedpath crosses the same landmarks.
The map on the right side is representative of maps used on websites, a Mercator projection using a cylinder tangent atto the equator:
The only places the map is accurate is along the equator. At any other latitude, it is distorted, the distortion increasesincreasing as we get closer to the poles. It's nonsense near the poles where the meridians never converge, giving Greenland (2 million km²) the size of Africa (30 million km²).
To limitOn the distortionimage above, the polar regionsfeatures are not projected and the projection doesn't usealong straight lines as shown above. Straight lines correspondLatitude $\small \phi$ corresponds to amap vertical position computed ascoordinate $\small y(\phi)=r \tan \phi$, but the. Mercator projection actually uses Mercator'sMercator function, equivalent to $\small y(\phi)=r \ln(\tan(\pi/4+\phi/2))$, in order to make it conformal, the goal Mercator was actually seeking after to make ship navigation easier. The aberrant polar regions are never shown.
Greenland, 2 million km², looks the same size as Africa, 30 million km² (seesee the True Size of Countries to understand how much this is wrong).
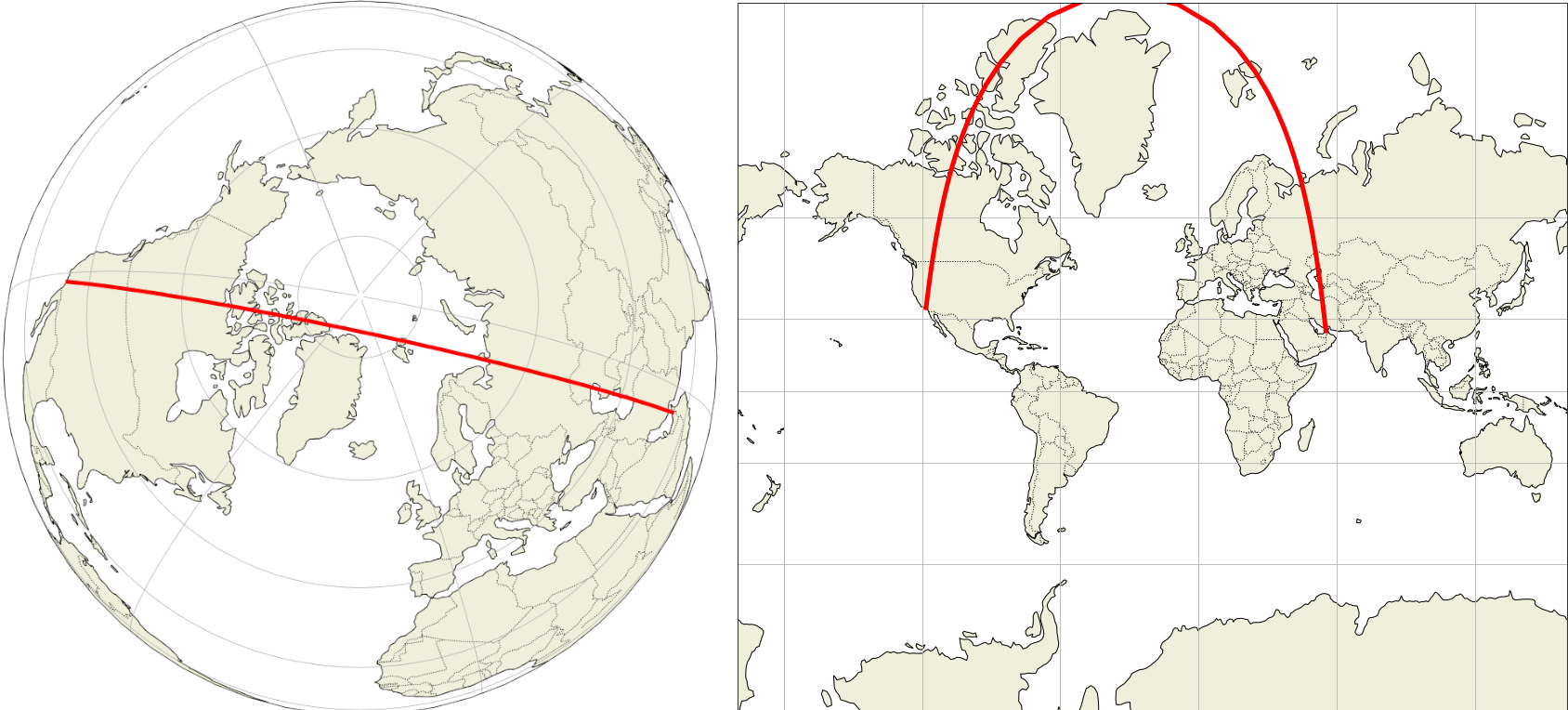
All great circles except for the equator and meridians are curved, more strongly at higher latitudes.
The heading is actually constant, but near the pole, the straight line (great circle) seems to make a U-turn. This is the most counterintuitive aspect.
The flat distance for LA-Grozny on the web Mercator projection (EPSG:3857) is 18,300 km, but the real ellipsoidal distance (on WGS 84 ellipsoid) is only about 11,300 km.