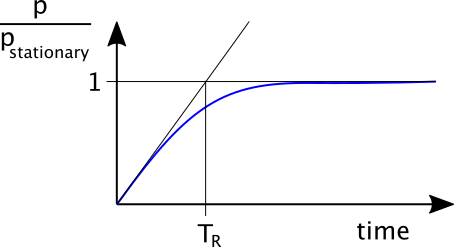
What you want is the roll constant $\text{T}_R$. This is basically one of the characteristics wich determines the equations of motion of an aircraft. It gives the slope of the roll speed increase over time with full aileron deflection and an ideally stiff wing, and equally the rate of decrease once the ailerons are set to neutral during a rolling maneuver.
With p the dimensionless roll rate, m the aircraft mass, i$_x$ its radius of the momentum of inertia about the roll axis, S its wing surface, b the wing span, v the flight speed, $\rho$ the density of air and $\text{c}_{lp}$ the roll damping coefficient, the formula is
$$T_R = \frac{2\cdot m\cdot\left(\frac{2\cdot i_x}{b}\right)^2}{\rho\cdot v\cdot S\cdot c_{lp}}$$
When the pilot moves the stick, the aircraft will accelerate into the roll but the acceleration will diminish with the square of roll speed until an asymptotic value is reached. This acceleration grows with increasing air density, flight speed, roll damping coefficient and lower wing loading and smaller square of the ratio of roll inertia over wing span. Same in reverse: Stopping will be fastest under the same conditions.
For the roll damping coefficient, use this approximation for wings with an aspect ratio AR larger than 4:
$$c_{lp} = -\frac{1}{4}\cdot\frac{\pi\cdot AR}{\sqrt{\frac{AR^2}{4}+4}+2}$$
Since this is a damping coefficient, it makes sense to be negative.
The asymptotic value is reached when the propelling moment from aileron deflection equals the retarding roll damping:
$$c_{l\xi} \cdot \frac{\xi_l - \xi_r}{2} = -c_{lp} \cdot \frac{\omega_x \cdot b}{2\cdot v_\infty} = -c_{lp} \cdot p$$
For an explanation of this equation and all the terms used please see this answer.
Please note that this is all only valid for a stiff airframe. Increasing dynamic pressure reduces aileron effectiveness because the wing will warp when ailerons are deflected. Assume a linear decrease with dynamic pressure until only a fraction of the ideal roll acceleration remains at top speed and low level flight.
Now you ask about roll damping torque and that cannot be explained in the comments. Look at the last equation – it is already there, albeit dimensionless. To get from there to an actual torque, multiply by wing area, semispan and dynamic pressure:
$$T=\frac{\rho}{2}\cdot v^2_\infty\cdot S\cdot\frac{b}{2}\cdot c_{lp}\cdot p = \frac{\rho}{8}\cdot v_\infty\cdot S\cdot b^2\cdot c_{lp}\cdot\omega_x$$
with $\omega_x$ the actual angular speed in rad/s. Do the unit check - this is actually a torsion moment [Nm]. Please note that I used the reference length for lateral moments used in Germany; the US uses the full span instead of the semispan. So make sure you check which reference length your sources use!