Blunt noses are best at subsonic speeds because they provide the best shape for the air to get out of the way. From this site:
The speed of "sound" is actually the speed of transmission of a small disturbance through a medium.
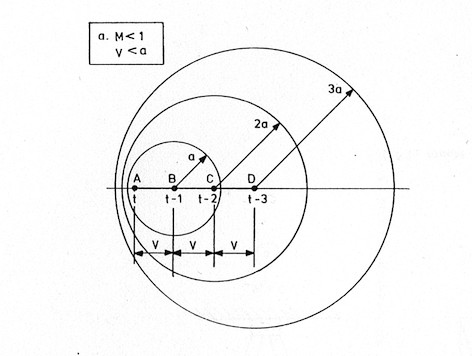
The picture shows a single point traveling at a constant speed V, emitting small disturbances :). The air in front of the travelling point is forewarned, and is pushed out of the way isentropically, without losses. It is this part of the subsonic airflow that is key to the optimal shape: a parabolical one. The point pushes air out of the way spherically, while travelling at a constant speed.
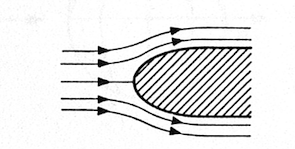
If our travelling disturbance is not an infinitesimally small point but an actual 3D body, its optimal shape is the same: parabolic, then rounded off at where the cylindrical body starts creating an elliptical shape. With this shape, the air in front of the body can get out of the way in the most orderly fashion.
The streamlines in the picture above are at equal distances. The air moves out of the way, creating a lower static pressure which sucks the nose into the airstream. With a hemispherical shape, the streamlines are closer together at some places, creating a pressure increase that negates the initial lower pressure.
Notice that in your figure 20 the first shape is parabolicelliptical and actually has a negative $C_D$ for the nose only: it sucks itself into the airflow. None of the other shapes do, not even the hemispherical shape 2. With subsonic incompressible flow, it's what happens in front of the nose that creates lower drag, not at or beyond the nose.
That's all valid when the shape travels at a certain speed, at zero Angle of Attack.
- At any other speed, the optimal parabolic shape is different, still a parabola though for an infinitely wide body, or an ellipse for a body with finite dimensions.
- At any other AoA, it is very difficult to create a 3D parabolicelliptical body shape, and it would be different for any AoA. But a spherical one comes close, as your Figure 20 shows - the first bit of a parabola is close to a sphere anyway. The larger the sphere radius, the closer it is to the optimum. And a sphere is a sphere at any angle.
Edit How can the shape suck itself into the air in front of it?
The pressure disturbances warn the air to get out of the way. Bernouilli is valid for low subsonic flow:
$$p_t = p_s + \frac{1}{2} \cdot \rho \cdot V^2$$
or: total pressure = static pressure plus dynamic pressure. Far in front of the moving body, $p_t$ is the total pressure is environment static pressure. As soon as the air starts to move, local dynamic pressure increases and local static pressure decreases.
Edit2
Yeah, elliptical for finite body dimensions.