Have I made a calculation mistake in this ATPL question that uses the CAP 698 Chart?

Question

I have been calculating this question but I just don't seem to get the correct answer.

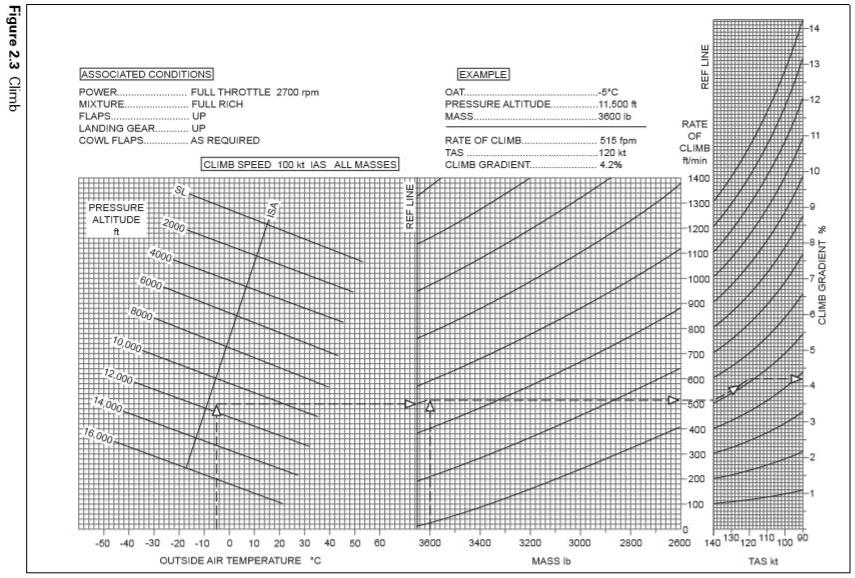
For this question use Performance Manual CAP 698 SEP1 Figure 2.3.

Using the climb performance chart, for the single-engine aeroplane, determine the ground distance to reach a height of 2000 ft in the following conditions:

---

Given:

OAT at take-off: 25°C

Airport pressure altitude: 1000 ft

Aeroplane mass: 3600 lb

Speed: 100 KIAS

Wind component: 15 kt Headwind

---

My Workings

*TAS = 104kt (from using CRP-5)

GS = 104kts - 15kts = 89kts* Climb Gradient = 10.7% (from chart)

Still Air Distance = (2000 / 10.7) X 100 = 18,691ft

Ground Distance = 18691 X (89/104) = 15,995ft

---

But the CAE Oxford ATPL says that 16,850ft is the answer.

The next closest answer is 15,750ft which is what I would choose.

Please help me out here, thank you in advance!

---

Reference: CAE Oxford ATPL Chapter 8, Question 4.

Answer 1

The relevant section (3.2.3) of CAP 698 explains how to calculate this as follows:

Distance to Reach given height.

To calculate the ground distance travelled in order to attain a given height above reference zero:
a) Convert the IAS 100 kt to a TAS, assume no position error.
b) Apply the wind component to the TAS to obtain the ground speed.
c) Determine the climb gradient from the graph.
d) Calculate the still air distance using the formula:
$$\text{Still Air Distance} (ft) = \frac{\text{Height Difference} (ft)}{\text{Gradient}} \times 100$$ e) Calculate ground distance using the formula: $$\text{Ground Distance} = \text{Still Air Distance} \times \frac{\text{Groundspeed}}{\text{TAS}}$$

Assuming your calculation of TAS is right, and your interpretation of the graph is right (which I agree with) you can plug this into the above calculations and confirm the answer is indeed:

Ground Distance = 15,995ft

However, if we play devil's advocate and reverse engineer the climb gradient from the expected answer, you'll see there might be some human/interpretation error at work.

Taking the published answer of 16,850ft and dividing by $\frac{\text{Groundspeed}}{\text{TAS}}$ gives us a ground distance of 19,690ft. Take this and Divide by 100 gives 196.9. Then doing $\frac{1}{196.9} \times \text{Height Difference} (ft)$ gives a resulting climb gradient of 10.2 - this is all just mathematics, no aviation knowledge required.

Now, can you say for sure that you've not misread/misused the chart? Once again I got the same result as you by looking at it in low def on a screen. But it's possible we both miscalculated the climb gradient.

---

Side note: I see question like this come up fairly regularly on other aviation forums, and a reasonable amount of time it is student error and a reasonable amount of time it is publication error. Make of that what you will.