6
$\begingroup$

Cd = Cdo + KCl2

Total drag = Parasite drag + Induced drag

When Cl = 0 , Cd = Cdo

Increasing in Cl introduces another component of drag (Induced Drag) and therefore the total drag Cd should be increased. But, Why in this plot Cd decreases initially with the small increment in Cl?

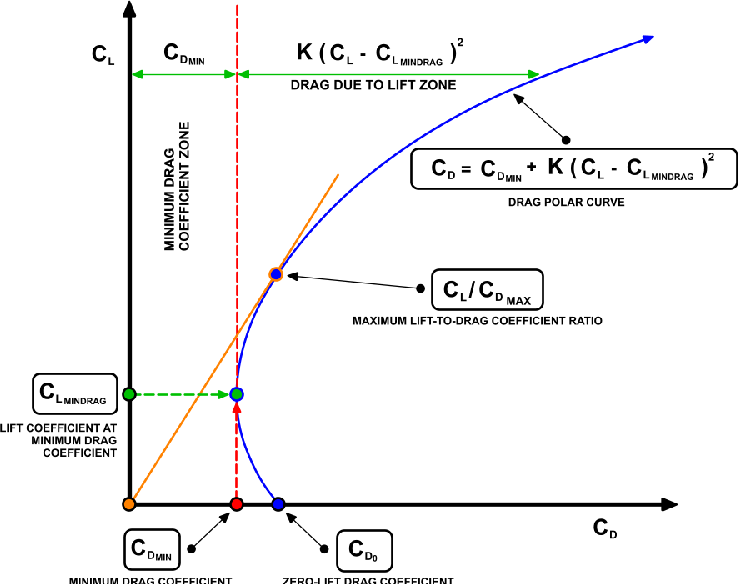
Cl vs Cd

Improve this question
$\endgroup$
4
  • $\begingroup$ I don't think the origin of the graph is at (0,0), so your question may be based on an incorrect premise. $\endgroup$
    – Mark Jones Jr.
    Commented Oct 16, 2019 at 16:27
  • $\begingroup$ Can you please add the source of the image? $\endgroup$
    – DeltaLima ♦
    Commented Oct 16, 2019 at 16:34
  • $\begingroup$ I'm not convinced that the origin is not at 0,0. $\endgroup$
    – quiet flyer
    Commented Oct 16, 2019 at 17:43
  • 1
    $\begingroup$ Possible duplicate of Is drag coefficient lowest at zero angle of attack? $\endgroup$
    – Manu H
    Commented Oct 17, 2019 at 9:20

3 Answers 3

Reset to default
9
$\begingroup$

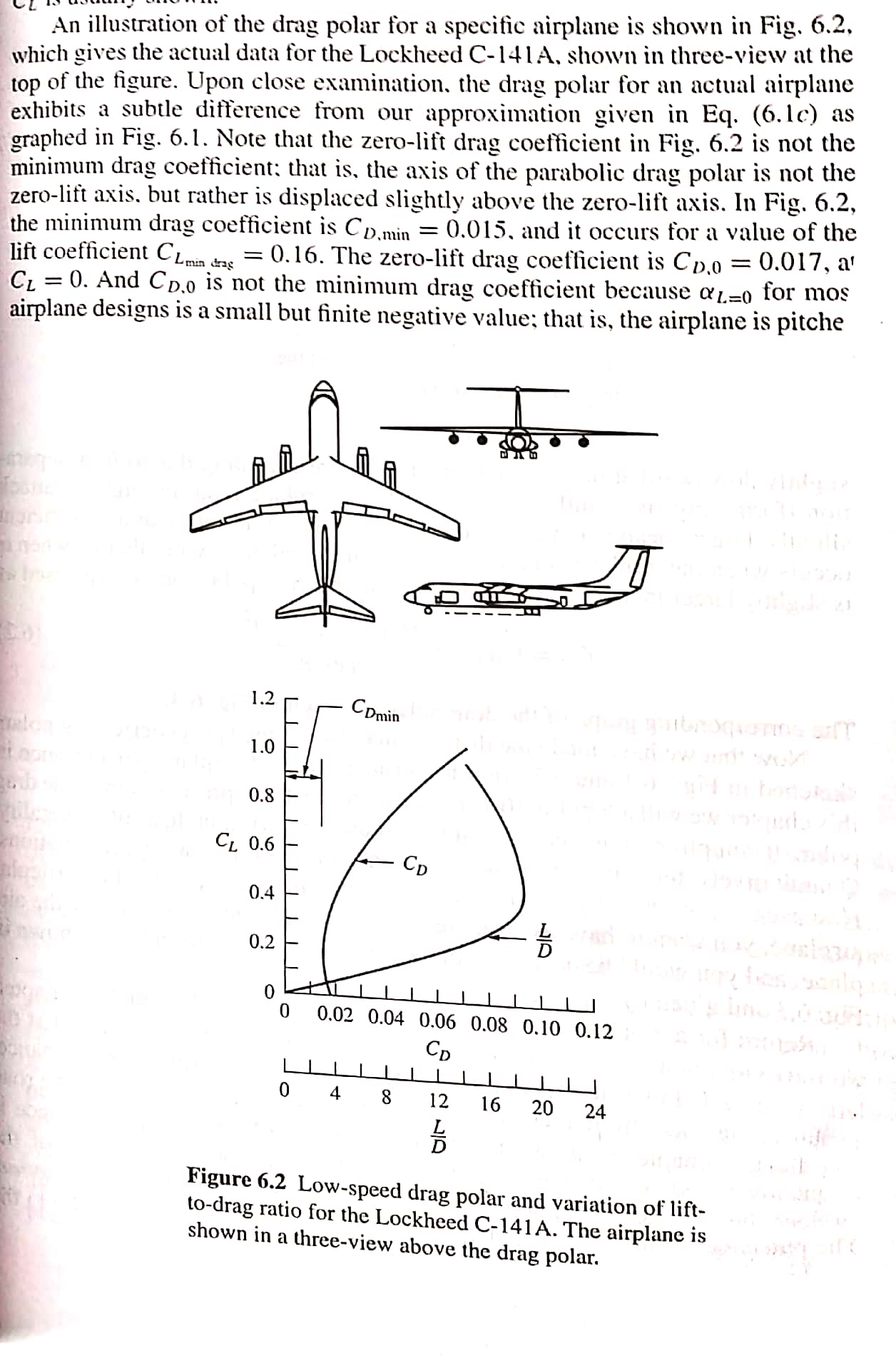
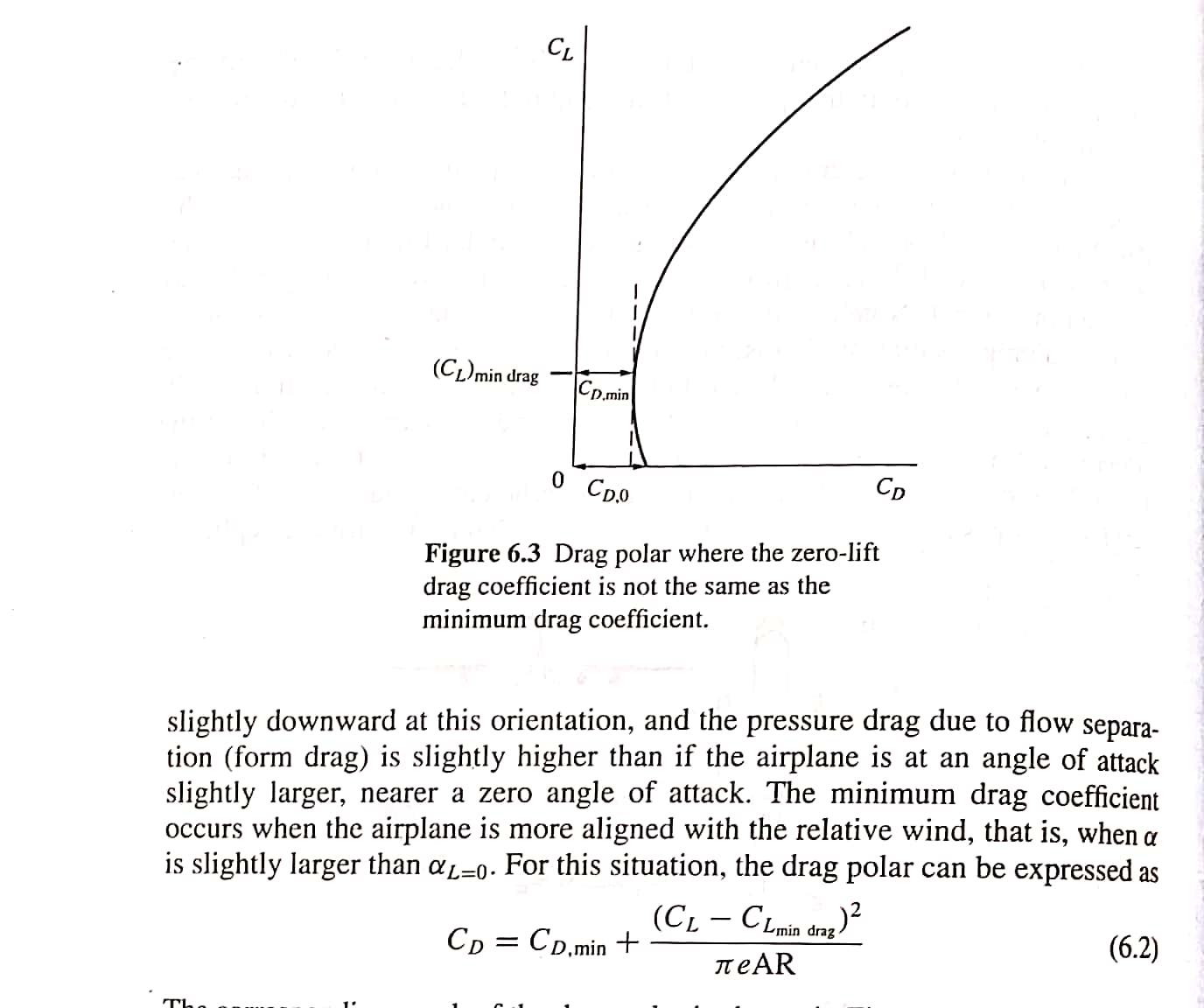
Think about it this way. Assume a cambered airfoil, not a symmetrical airfoil. Assume the wing has zero twist and zero incidence. To place the wing at the zero-lift angle-of-attack (zero CL), the fuselage and wing will have to fly at a somewhat nose-down pitch attitude relative to the airflow. This does not yield the lowest possible drag coefficient, regardless of whether we are looking at the wing, the fuselage, or the whole aircraft.

Bear in mind that in this part of the flight envelope (near the zero-lift angle-of-attack), drag is dominated by profile drag, not induced drag.

Related: Is drag coefficient lowest at zero angle of attack?

Now imagine using the same data to generate a graph of CD versus airspeed, assuming Lift=Weight. What would that look like? Also what would a graph of Drag versus airspeed look like?

Improve this answer
$\endgroup$
2
  • $\begingroup$ aviation.stackexchange.com/questions/62357/… $\endgroup$
    – Nirmal
    Commented Oct 16, 2019 at 18:29
  • $\begingroup$ Right, now incorporated, thanks. I guess it's a duplicate question or nearly so. Zero a-o-a and zero-lift a-o-a are not exactly the same thing. $\endgroup$
    – quiet flyer
    Commented Oct 16, 2019 at 19:02
5
$\begingroup$

The well-known 3D drag model of:

$$C_D=C_{D_0}+KC_L^2$$

only holds well for high aspect ratio, flap retracted wing configurations (source: ESDU Item 74035).

With flaps extended, a better model would be (source: ESDU Item 97002):

$$C_D=C_{D_0}+AC_L+BC_L^2$$

If you rearrange the terms a little bit, you get the model shown in your graph:

$$C_D=C_{D_{min}}+K(C_L-C_{L_{mindrag}})^2$$

Improve this answer
$\endgroup$
1
  • $\begingroup$ I don't think the flaps are the cause of the $C_{D_{mindrag}}$ and $C_{D_0}$ discrepancy. This phenomenon also occurs when the flaps are retracted (due to the reasons mentioned by quiet flyer). Also, it's not clear to me how the rearrangement of terms (in which equation, the one with the flaps?) should lead to the final result $\endgroup$
    – ROIMaison
    Commented Mar 21 at 12:39
2
$\begingroup$

Source: INTRODUCTION TO FLIGHT - John D Anderson

Introduction To flight - Anderson

Introduction to flight - Anderson

Improve this answer
$\endgroup$
1
  • $\begingroup$ might be copyright issues just posting part of a book like that $\endgroup$
    – jk.
    Commented Oct 17, 2019 at 10:36

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .